1 2 Divided By 5 8 As A Fraction
Treneri
May 11, 2025 · 4 min read
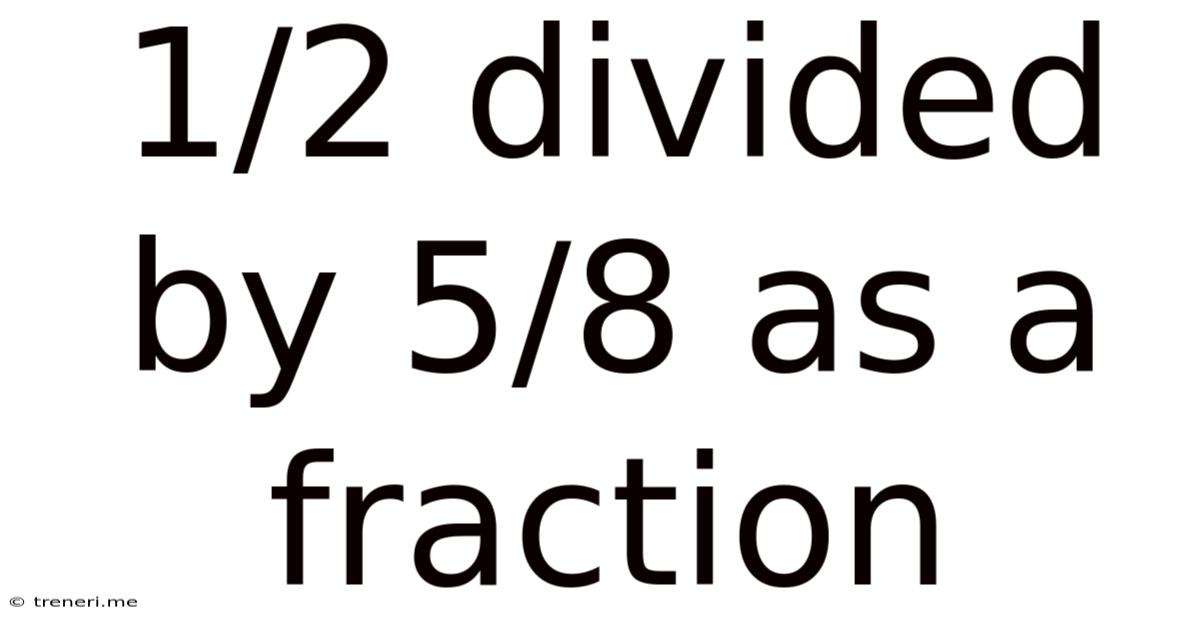
Table of Contents
12 Divided by 58 as a Fraction: A Comprehensive Guide
Understanding how to represent division problems as fractions is a fundamental concept in mathematics. This guide will delve into the process of expressing "12 divided by 58" as a fraction, covering various aspects including simplification, decimal representation, and real-world applications. We'll also explore related concepts to solidify your understanding.
Understanding Fractions and Division
Before we tackle the specific problem, let's refresh our understanding of fractions and their relationship to division. A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The fraction a/b signifies 'a' parts out of a total of 'b' equal parts.
Division, on the other hand, is the process of splitting a quantity into equal parts. The result of a division operation can always be expressed as a fraction. The dividend (the number being divided) becomes the numerator, and the divisor (the number you're dividing by) becomes the denominator.
Therefore, "12 divided by 58" can be directly written as the fraction:
12/58
Simplifying the Fraction
The fraction 12/58 is not in its simplest form. A simplified fraction is one where the numerator and the denominator have no common factors other than 1. To simplify, we need to find the greatest common divisor (GCD) of 12 and 58.
The factors of 12 are 1, 2, 3, 4, 6, and 12. The factors of 58 are 1, 2, 29, and 58.
The greatest common factor of 12 and 58 is 2. To simplify, we divide both the numerator and the denominator by 2:
12 ÷ 2 = 6 58 ÷ 2 = 29
Therefore, the simplified fraction is:
6/29
Converting to Decimal
While the fraction 6/29 is the simplest representation, it's often useful to convert it to a decimal for practical applications. To do this, we divide the numerator (6) by the denominator (29):
6 ÷ 29 ≈ 0.20689655...
This decimal representation is an approximation because the division results in a non-terminating, repeating decimal. For many purposes, rounding to a specific number of decimal places is sufficient. For example, rounded to three decimal places, we get:
0.207
Real-World Applications
The concept of expressing division as a fraction and simplifying it is crucial in various real-world scenarios. Here are a few examples:
-
Baking: If you have a recipe that calls for 12 ounces of flour for a 58-ounce cake, the fraction 12/58 (simplified to 6/29) represents the proportion of flour in the entire cake. This helps in scaling recipes up or down.
-
Budgeting: If you have $12 and want to divide it among 58 people, the fraction 12/58 (simplified to 6/29) shows how much money each person receives.
-
Probability: If you have 12 red balls and 58 total balls in a bag, the fraction 12/58 (simplified to 6/29) represents the probability of drawing a red ball at random.
-
Measurements: Imagine cutting a 58-inch long rope into 12 equal pieces. The length of each piece would be represented by the fraction 58/12 (simplified to 29/6).
Related Concepts: Improper Fractions and Mixed Numbers
Sometimes, the result of a division problem leads to an improper fraction, where the numerator is larger than the denominator. For example, if we were to divide 58 by 12, we'd get 58/12. This can be converted into a mixed number, which consists of a whole number and a proper fraction.
To convert 58/12 to a mixed number, we perform the division:
58 ÷ 12 = 4 with a remainder of 10
This means that 58/12 can be written as 4 and 10/12. Further simplification of the fraction part (10/12) gives us 5/6. So the mixed number is:
4 5/6
Equivalent Fractions
It's important to remember that a fraction can have many equivalent forms. For instance, 6/29, 12/58, 18/87, and so on, are all equivalent fractions because they represent the same value. They can be obtained by multiplying or dividing both the numerator and denominator by the same non-zero number. This concept is crucial when comparing and working with fractions.
Advanced Concepts: Rational Numbers
Fractions represent rational numbers, which are numbers that can be expressed as the quotient or fraction p/q of two integers, a numerator p and a non-zero denominator q. Understanding rational numbers opens the door to more complex mathematical operations and concepts, including algebraic manipulations, equation solving, and calculus.
Conclusion
Expressing "12 divided by 58" as a fraction involves a straightforward process: writing it as 12/58 and then simplifying it to its simplest form, 6/29. Understanding the relationship between fractions and division, along with the ability to simplify fractions, convert them to decimals, and work with related concepts like mixed numbers, is essential for various mathematical applications and real-world problems. Mastering these fundamental concepts provides a solid foundation for more advanced mathematical studies. This comprehensive guide aims to enhance your understanding and equip you with the necessary knowledge to confidently tackle similar problems. Remember to always practice regularly to build your skills and confidence in working with fractions.
Latest Posts
Latest Posts
-
60 Days From July 30 2024
May 13, 2025
-
2 Tbsp Olive Oil In Grams
May 13, 2025
-
How To Get Y Intercept From 2 Points
May 13, 2025
-
30 Quarts Equals How Many Gallons
May 13, 2025
-
Wie Viele Wochen Hat Das Jahr
May 13, 2025
Related Post
Thank you for visiting our website which covers about 1 2 Divided By 5 8 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.