1/3 + 3/5 As A Fraction
Treneri
May 12, 2025 · 5 min read
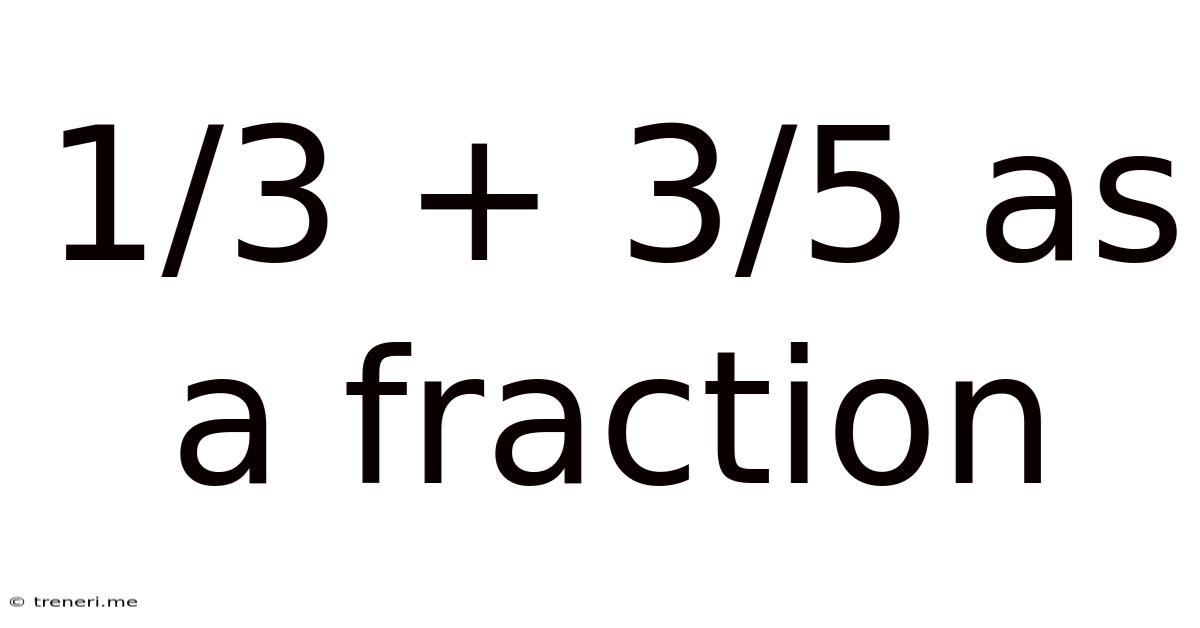
Table of Contents
1/3 + 3/5 as a Fraction: A Comprehensive Guide
Adding fractions might seem like a simple arithmetic task, but understanding the underlying principles ensures accuracy and builds a strong foundation for more complex mathematical operations. This comprehensive guide will walk you through adding 1/3 and 3/5, explaining the process step-by-step and exploring the broader concepts involved. We'll cover finding the least common denominator (LCD), simplifying fractions, and even delve into the practical applications of fraction addition.
Understanding Fractions
Before we dive into the addition, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates how many parts you have, while the denominator indicates how many parts make up the whole. For example, in the fraction 1/3, the numerator is 1 and the denominator is 3. This means you have 1 out of 3 equal parts.
Key Fraction Concepts
- Equivalent Fractions: Fractions that represent the same value, even though they look different. For example, 1/2, 2/4, and 3/6 are all equivalent fractions.
- Simplifying Fractions: Reducing a fraction to its simplest form by dividing both the numerator and denominator by their greatest common divisor (GCD). For example, 6/8 can be simplified to 3/4 by dividing both by 2.
- Improper Fractions: Fractions where the numerator is greater than or equal to the denominator (e.g., 7/4).
- Mixed Numbers: A combination of a whole number and a proper fraction (e.g., 1 3/4).
Adding Fractions: The Step-by-Step Process
Adding fractions with different denominators requires finding a common denominator—a number that both denominators can divide into evenly. The most efficient common denominator is the least common denominator (LCD).
1. Find the Least Common Denominator (LCD)
To add 1/3 and 3/5, we first need to find the LCD of 3 and 5. One method is to list the multiples of each number until you find a common one:
- Multiples of 3: 3, 6, 9, 12, 15, 18...
- Multiples of 5: 5, 10, 15, 20...
The least common multiple (LCM) of 3 and 5 is 15. Therefore, the LCD of the fractions 1/3 and 3/5 is 15.
2. Convert Fractions to Equivalent Fractions with the LCD
Now we need to convert both fractions to equivalent fractions with a denominator of 15:
- For 1/3: To get a denominator of 15, we multiply both the numerator and denominator by 5: (1 × 5) / (3 × 5) = 5/15
- For 3/5: To get a denominator of 15, we multiply both the numerator and denominator by 3: (3 × 3) / (5 × 3) = 9/15
3. Add the Numerators
Now that both fractions have the same denominator, we can add them by simply adding the numerators:
5/15 + 9/15 = (5 + 9) / 15 = 14/15
4. Simplify the Result (If Necessary)
In this case, 14/15 is already in its simplest form because 14 and 15 have no common divisors other than 1.
Therefore, 1/3 + 3/5 = 14/15
Alternative Methods for Finding the LCD
While listing multiples is a straightforward method for finding the LCD, especially for smaller numbers, other techniques are more efficient for larger numbers or when dealing with multiple fractions:
-
Prime Factorization: Break down each denominator into its prime factors. The LCD is the product of the highest powers of all prime factors present in the denominators.
For example:
3 = 3 5 = 5
LCD = 3 × 5 = 15
-
Using the formula: If you have two numbers a and b, their least common multiple (LCM) can be found using the formula: LCM(a,b) = (a × b) / GCD(a,b), where GCD is the greatest common divisor. In our case, the GCD of 3 and 5 is 1, so LCM(3,5) = (3 × 5) / 1 = 15.
Practical Applications of Fraction Addition
Adding fractions is not just a theoretical exercise; it has numerous real-world applications:
- Cooking and Baking: Recipes often require fractional amounts of ingredients. Adding fractions is crucial for calculating the total amount of an ingredient needed.
- Construction and Engineering: Precise measurements are essential in construction and engineering. Working with fractional dimensions is commonplace, and adding fractions is necessary for calculating total lengths, areas, or volumes.
- Finance: Calculating portions of budgets, interest rates, or shares often involves adding fractions.
- Data Analysis: Representing and analyzing data frequently uses fractions and requires adding them to understand the overall picture.
Beyond Simple Addition: Working with Mixed Numbers and Improper Fractions
The process extends to mixed numbers and improper fractions. Let's look at an example with mixed numbers:
Example: 1 1/2 + 2 2/3
-
Convert Mixed Numbers to Improper Fractions:
- 1 1/2 = (1 × 2 + 1) / 2 = 3/2
- 2 2/3 = (2 × 3 + 2) / 3 = 8/3
-
Find the LCD: The LCD of 2 and 3 is 6.
-
Convert to Equivalent Fractions with the LCD:
- 3/2 = (3 × 3) / (2 × 3) = 9/6
- 8/3 = (8 × 2) / (3 × 2) = 16/6
-
Add the Numerators:
9/6 + 16/6 = 25/6
-
Convert back to a Mixed Number (if necessary):
25/6 = 4 1/6
Conclusion
Mastering fraction addition is fundamental to a deeper understanding of mathematics. By understanding the underlying principles, such as finding the least common denominator and simplifying fractions, you can confidently tackle more complex problems involving fractions. Remember to practice regularly to solidify your understanding and build your mathematical skills. The ability to add fractions accurately is a valuable skill applicable across numerous fields and everyday situations. From cooking to engineering, finance to data analysis, the ability to work with fractions is essential for precision and accuracy. So, next time you encounter the task of adding fractions, remember the steps outlined in this guide and approach it with confidence.
Latest Posts
Latest Posts
-
How Many Ounces Is In 3 Pounds
May 13, 2025
-
What Is A 60 As A Grade
May 13, 2025
-
Cuanto Es 100 Gramos En Cups
May 13, 2025
-
How Long Is 44 Days In Months
May 13, 2025
-
A Wave Of Frequency Of 1000 Hertz Vibrates At
May 13, 2025
Related Post
Thank you for visiting our website which covers about 1/3 + 3/5 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.