1 4 Divided By 1 3 In Fraction
Treneri
May 14, 2025 · 5 min read
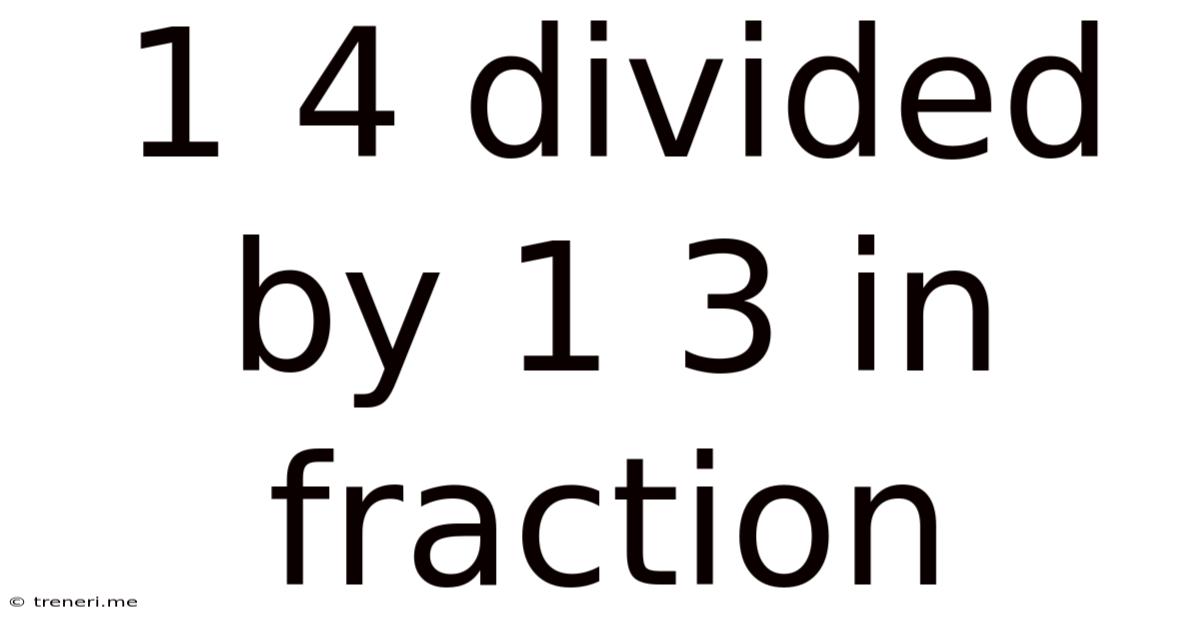
Table of Contents
1 4/1 ÷ 1 3/1 in Fraction: A Comprehensive Guide to Division of Mixed Numbers
Dividing fractions, especially mixed numbers, can seem daunting, but with a structured approach, it becomes manageable. This comprehensive guide will walk you through the process of dividing 1 4/1 by 1 3/1, explaining the concepts and providing step-by-step instructions. We'll explore different methods, ensuring you grasp the underlying principles and can confidently tackle similar problems in the future. Understanding this fundamental arithmetic operation is crucial for various applications, from baking and crafting to more advanced mathematical fields.
Understanding Mixed Numbers and Improper Fractions
Before diving into the division, let's solidify our understanding of mixed numbers and improper fractions. A mixed number combines a whole number and a fraction, like 1 4/1 (one and four-fifths). An improper fraction, on the other hand, has a numerator larger than or equal to its denominator, such as 9/5. It's important to be comfortable converting between these two forms.
Converting Mixed Numbers to Improper Fractions
To convert a mixed number to an improper fraction, follow these steps:
- Multiply the whole number by the denominator of the fraction.
- Add the result to the numerator of the fraction.
- Keep the same denominator.
Let's apply this to 1 4/1:
- 1 (whole number) * 1 (denominator) = 1
- 1 (result) + 4 (numerator) = 5
- The denominator remains 1.
Therefore, 1 4/1 is equivalent to the improper fraction 5/1.
Similarly, let's convert 1 3/1:
- 1 (whole number) * 1 (denominator) = 1
- 1 (result) + 3 (numerator) = 4
- The denominator remains 1.
Thus, 1 3/1 becomes 4/1.
Dividing Fractions: The Reciprocal Method
The most straightforward way to divide fractions is by using the reciprocal. The reciprocal of a fraction is simply the fraction flipped upside down. For example, the reciprocal of 2/3 is 3/2.
The process of dividing fractions involves:
- Converting mixed numbers to improper fractions (as we did above).
- Changing the division sign to a multiplication sign.
- Replacing the second fraction with its reciprocal.
- Multiplying the numerators together.
- Multiplying the denominators together.
- Simplifying the resulting fraction (if possible).
Let's apply this method to our problem, 1 4/1 ÷ 1 3/1:
-
Convert to improper fractions: We already did this: 5/1 ÷ 4/1
-
Change to multiplication and use the reciprocal: 5/1 * 1/4
-
Multiply numerators and denominators: (5 * 1) / (1 * 4) = 5/4
-
Simplify: The fraction 5/4 is already in its simplest form.
Therefore, 1 4/1 ÷ 1 3/1 = 5/4. This improper fraction can be converted back to a mixed number: 1 1/4.
Alternative Method: Long Division
While the reciprocal method is generally quicker, you can also use long division to solve this problem. This method offers a different perspective and might be helpful for those who prefer a more visual approach.
Since we're dividing 1 4/1 by 1 3/1, this translates to dividing 5/1 by 4/1. Remember, dividing by a fraction is the same as multiplying by its reciprocal. Let's express it as a long division problem:
1.25
4 | 5.00
-4
10
-8
20
-20
0
This shows that 5 divided by 4 is 1.25. To convert this decimal back into a fraction, we can write it as 1 1/4 (since 0.25 is equivalent to 1/4).
Real-World Applications: Why This Matters
Understanding fraction division isn't just about passing a math test; it's a practical skill with applications in many areas:
-
Cooking and Baking: Recipes often require dividing ingredients. For instance, if a recipe calls for 1 1/2 cups of flour and you want to halve it, you'll need to divide by 2.
-
Sewing and Crafting: Cutting fabric or other materials accurately necessitates fractional calculations. Dividing lengths to create multiple pieces requires a solid grasp of fraction division.
-
Construction and Engineering: Precision is paramount in construction. Dividing measurements to fit materials or plan layouts often involves fractions.
-
Data Analysis: In fields like statistics and data analysis, working with fractions and proportions is common. Understanding fraction division is essential for interpreting and manipulating data.
Advanced Concepts: Extending Your Knowledge
This problem provided a solid foundation. However, let's briefly touch upon scenarios involving more complex fractions:
-
Dividing fractions with different denominators: Remember to find a common denominator before adding or subtracting fractions. With division, you'll convert to improper fractions and then use the reciprocal method.
-
Dividing fractions with larger numbers: The process remains the same, but the calculations might be more involved. A calculator can assist with the arithmetic, but understanding the underlying principles is key.
-
Dividing fractions that result in repeating decimals: Some fraction divisions yield repeating decimals (e.g., 1/3 = 0.333...). It's crucial to know how to express these decimals accurately in fractional form.
-
Word problems: Applying fraction division to real-world scenarios is a crucial skill. Practice solving word problems to enhance your understanding.
Conclusion: Mastering Fraction Division
Dividing mixed numbers, like 1 4/1 by 1 3/1, might appear challenging initially. However, by breaking down the process into manageable steps – converting to improper fractions, using reciprocals, and simplifying – it becomes significantly easier. Understanding the underlying principles empowers you to solve a wide range of fraction division problems confidently. Mastering this skill is valuable not only for academic success but also for practical applications in various aspects of life. Remember to practice regularly to solidify your understanding and enhance your problem-solving abilities. Through consistent effort, you'll develop proficiency in handling fractions and unlock a broader range of mathematical possibilities.
Latest Posts
Latest Posts
-
100 Pies En Metros Cuanto Es
May 14, 2025
-
How Many Cups In 32 Ounce
May 14, 2025
-
How Many Days Is 90 Years
May 14, 2025
-
What Is 25 Off Of 100
May 14, 2025
-
Greatest Common Factor Of 54 And 81
May 14, 2025
Related Post
Thank you for visiting our website which covers about 1 4 Divided By 1 3 In Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.