1 8 Is Equal To What Fraction
Treneri
May 15, 2025 · 5 min read
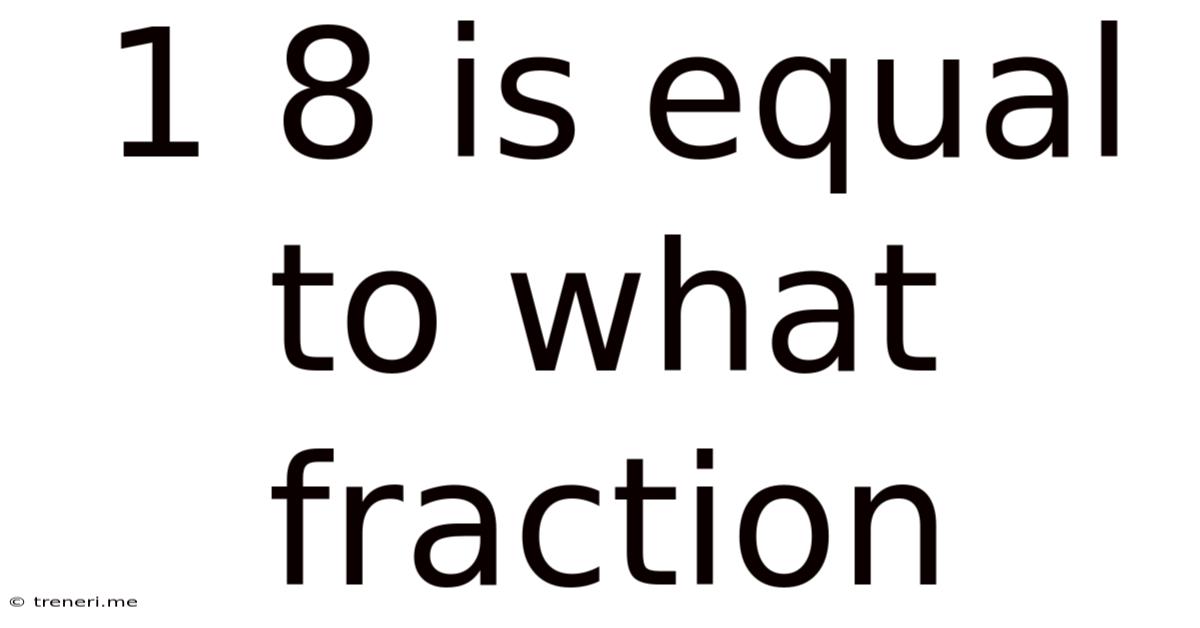
Table of Contents
1 ÷ 8 is Equal to What Fraction? A Comprehensive Guide
Understanding fractions is fundamental to mathematics, and knowing how to represent division problems as fractions is a crucial skill. This comprehensive guide will explore the simple yet important question: what fraction is equal to 1 divided by 8 (1 ÷ 8)? We'll delve into the concept, provide various approaches to solving it, and offer practical applications to solidify your understanding.
Understanding Fractions and Division
Before diving into the specific problem, let's refresh our understanding of fractions and their relationship with division. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts the whole is divided into.
Division, on the other hand, is the process of splitting a quantity into equal groups. Crucially, division and fractions are intrinsically linked. When we divide a number (dividend) by another number (divisor), the result can be expressed as a fraction where the dividend is the numerator, and the divisor is the denominator.
Solving 1 ÷ 8: The Direct Approach
The most straightforward approach to finding the fraction equal to 1 ÷ 8 is to directly represent the division as a fraction. Since 1 is the dividend and 8 is the divisor, the fraction is:
1/8
This fraction is already in its simplest form; we cannot further reduce the numerator and denominator by dividing them both by a common factor other than 1. Therefore, 1 divided by 8 is equal to one-eighth.
Visualizing 1 ÷ 8
Visualizing fractions can significantly enhance understanding, particularly for beginners. Imagine a pizza cut into eight equal slices. If you take one slice, you have 1 out of 8 slices, representing the fraction 1/8. This visual representation reinforces the concept that 1/8 represents one part of a whole that's divided into eight equal parts.
Equivalent Fractions: Exploring Alternatives
While 1/8 is the simplest and most common representation of 1 ÷ 8, it's important to understand that equivalent fractions exist. Equivalent fractions represent the same value but have different numerators and denominators. We can create equivalent fractions by multiplying both the numerator and denominator of 1/8 by the same number.
For example:
- Multiplying both by 2: (1 x 2) / (8 x 2) = 2/16
- Multiplying both by 3: (1 x 3) / (8 x 3) = 3/24
- Multiplying both by 10: (1 x 10) / (8 x 10) = 10/80
All these fractions – 2/16, 3/24, 10/80, and so on – are equivalent to 1/8. They represent the same portion of a whole. However, 1/8 is the simplest form because its numerator and denominator share no common factors other than 1.
Decimal Representation: Converting to Decimal
Fractions can also be represented as decimals. To convert 1/8 to a decimal, we perform the division: 1 ÷ 8 = 0.125. This decimal represents the same value as the fraction 1/8. Understanding both fractional and decimal representations is vital for various mathematical operations and applications.
Applications of 1/8 in Real-Life Scenarios
The fraction 1/8 appears frequently in various real-world situations. Here are a few examples:
- Cooking and Baking: Recipes often call for fractional amounts of ingredients. A recipe might require 1/8 teaspoon of salt or 1/8 cup of sugar.
- Measurement: Measurements in inches, feet, and other units sometimes involve fractions. You might measure a piece of wood as being 1/8 of an inch thick.
- Sharing and Division: Imagine sharing a pizza among eight friends. Each friend would receive 1/8 of the pizza.
- Probability: In probability calculations, 1/8 might represent the probability of a specific event occurring. For example, if there are eight equally likely outcomes, the probability of a particular outcome is 1/8.
Advanced Concepts: Working with 1/8 in More Complex Calculations
Understanding 1/8 lays the foundation for more advanced mathematical concepts. Here are a few examples:
-
Adding and Subtracting Fractions: To add or subtract fractions, they must have a common denominator. If you needed to add 1/8 and 3/8, you would simply add the numerators (1 + 3 = 4), keeping the denominator the same: 4/8 (which simplifies to 1/2).
-
Multiplying Fractions: Multiplying fractions involves multiplying the numerators together and the denominators together. For example, 1/8 multiplied by 2/3 would be (1 x 2) / (8 x 3) = 2/24 (which simplifies to 1/12).
-
Dividing Fractions: Dividing fractions involves inverting (flipping) the second fraction and then multiplying. For example, 1/8 divided by 1/2 would be 1/8 x 2/1 = 2/8 (which simplifies to 1/4).
-
Fractions in Algebra: Fractions appear extensively in algebraic expressions and equations. Solving for variables often involves manipulating fractions.
Troubleshooting Common Mistakes
When working with fractions, certain mistakes are common:
-
Incorrect Simplification: Always simplify fractions to their lowest terms by dividing the numerator and denominator by their greatest common divisor.
-
Adding or Subtracting Incorrectly: Remember to find a common denominator before adding or subtracting fractions.
-
Multiplying or Dividing Incorrectly: Carefully apply the rules for multiplying and dividing fractions.
-
Misunderstanding Equivalent Fractions: Remember that equivalent fractions have the same value even though they appear different.
Conclusion: Mastering 1/8 and Beyond
Understanding that 1 ÷ 8 equals 1/8 is a fundamental step in mastering fractions. This seemingly simple concept forms the basis for more complex mathematical operations and real-world applications. By practicing various approaches—visualizations, decimal conversions, and working through examples—you can strengthen your understanding and confidently apply your knowledge in various contexts. Remember the importance of simplification and the concept of equivalent fractions. Through consistent practice and attention to detail, you can confidently navigate the world of fractions and beyond. The seemingly simple problem of 1 ÷ 8 provides a stepping stone to a deeper appreciation of mathematics and its role in everyday life.
Latest Posts
Latest Posts
-
88 83 Is What Percent Of 21
May 15, 2025
-
Cuantas Semanas Tiene Un Ano De 365 Dias
May 15, 2025
-
How Many Ounce In A Kilogram
May 15, 2025
-
How Many Cubic Feet Is 55 Quarts Of Potting Soil
May 15, 2025
-
What Is The Gcf For 18 And 32
May 15, 2025
Related Post
Thank you for visiting our website which covers about 1 8 Is Equal To What Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.