1 In 80 As A Percentage
Treneri
May 13, 2025 · 5 min read
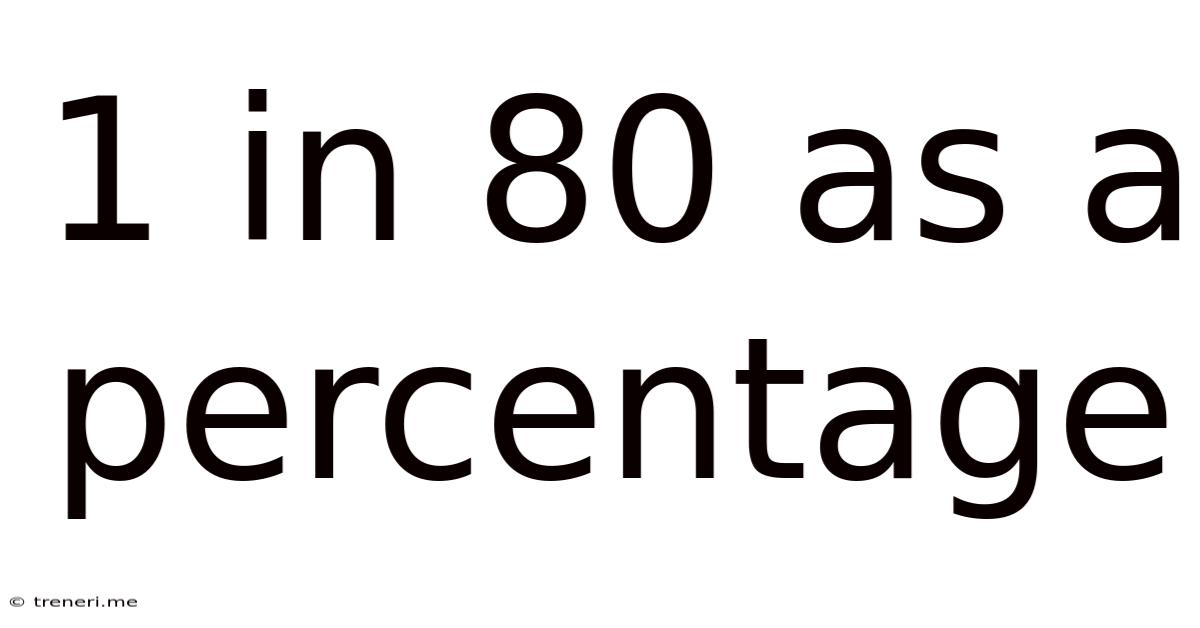
Table of Contents
1 in 80 as a Percentage: A Comprehensive Guide
Understanding fractions, decimals, and percentages is crucial in various aspects of life, from everyday calculations to complex statistical analyses. Converting fractions like "1 in 80" into percentages allows for easier comparison and comprehension, especially when dealing with probabilities, risk assessments, or data representation. This comprehensive guide will walk you through the process of converting 1 in 80 to a percentage, exploring different methods and providing practical examples to solidify your understanding.
Understanding the Concept: From Fraction to Percentage
The phrase "1 in 80" represents a fraction where 1 is the numerator (the part) and 80 is the denominator (the whole). To convert this fraction to a percentage, we need to express it as a proportion of 100. Percentages represent parts per hundred, making them ideal for comparing different proportions easily.
The fundamental conversion process involves two key steps:
- Converting the fraction to a decimal: Divide the numerator (1) by the denominator (80).
- Converting the decimal to a percentage: Multiply the decimal by 100 and add the percent symbol (%).
Calculating 1 in 80 as a Percentage: Step-by-Step Guide
Let's break down the conversion process:
-
Divide the numerator by the denominator:
1 ÷ 80 = 0.0125
-
Multiply the decimal by 100:
0.0125 x 100 = 1.25
-
Add the percent symbol:
1.25%
Therefore, 1 in 80 is equal to 1.25%. This means that for every 80 instances, you can expect the event described by the fraction "1 in 80" to occur approximately 1.25 times.
Alternative Methods for Conversion
While the above method is straightforward, let's explore alternative approaches to reach the same result:
Method 2: Using Proportions
We can set up a proportion to solve for the percentage:
- 1/80 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', cross-multiply:
- 80x = 100
- x = 100/80
- x = 1.25
Therefore, x = 1.25%, confirming our previous result.
Method 3: Utilizing a Calculator
Most calculators have built-in functions to handle fraction-to-percentage conversions. Simply input the fraction 1/80 and convert it to a percentage using the calculator's features. This method offers speed and accuracy, especially when dealing with more complex fractions.
Practical Applications of 1.25%
Understanding that 1 in 80 equates to 1.25% is useful in numerous real-world scenarios:
-
Risk Assessment: If there's a 1 in 80 chance of a specific event occurring (e.g., a machine malfunction), knowing this is a 1.25% risk helps in decision-making and risk mitigation strategies.
-
Probability and Statistics: In statistical analysis, understanding this conversion is fundamental for interpreting probabilities and drawing meaningful conclusions from data. For instance, if a study shows that 1 in 80 people exhibit a particular trait, expressing it as 1.25% makes the finding easier to understand and visualize.
-
Data Representation: When presenting data visually (e.g., charts and graphs), representing fractions as percentages provides a more clear and readily understandable format for the audience.
-
Quality Control: In manufacturing, if 1 out of every 80 products is defective, the 1.25% defect rate provides a quantifiable metric for evaluating the production process's efficiency.
Expanding on the Concept: Variations and Extensions
The principle of converting fractions to percentages extends beyond simple scenarios like "1 in 80." Let's consider some variations:
More Complex Fractions: "x in y" as a Percentage
The process remains consistent for any fraction "x in y," where 'x' is the numerator and 'y' is the denominator:
- Divide x by y.
- Multiply the resulting decimal by 100.
- Add the percentage symbol.
Dealing with Larger Numbers: Improving Accuracy
When dealing with larger numbers, rounding may be necessary to maintain clarity and practicality. However, it's crucial to be mindful of the level of precision required in specific contexts. For instance, in scientific research, higher precision might be crucial, while in everyday applications, rounding to one or two decimal places is often sufficient.
Understanding the Significance of Precision
The precision of the percentage (1.25%) is directly related to the accuracy of the original data and the purpose of the analysis. In some cases, rounding to a whole number percentage (1%) might suffice, providing a simpler representation. However, in other instances, maintaining the precision of 1.25% is necessary to reflect the exact proportion.
Beyond the Calculation: Interpreting the Percentage
Simply calculating the percentage is only half the battle. Understanding the implications of that percentage within the context of the problem is vital. For example, a 1.25% chance of failure might be acceptable in some contexts (e.g., a low-stakes game), but unacceptable in others (e.g., a critical piece of medical equipment). The percentage provides a quantitative measure, but the interpretation must consider the qualitative aspects of the situation.
Conclusion: Mastering Fraction-to-Percentage Conversions
Converting "1 in 80" to 1.25% is a straightforward process with broad applicability. Mastering this conversion enhances your ability to understand and interpret data, assess risks, and make informed decisions in various aspects of life, from everyday calculations to complex statistical analysis. By understanding the underlying principles and applying the methods outlined above, you can confidently convert fractions to percentages, ensuring accurate representation and clear communication of proportions. Remember to always consider the context and the required level of precision when interpreting the resulting percentage. This understanding empowers you to make more informed decisions based on the data at hand.
Latest Posts
Latest Posts
-
4 To The Power Of 1 5
May 14, 2025
-
How To Get Mass Flow Rate From Volumetric Flow Rate
May 14, 2025
-
How Many Miles Is 115 Km
May 14, 2025
-
How Far Along Is My Cat Pregnant
May 14, 2025
-
How Many Weeks Is 97 Days
May 14, 2025
Related Post
Thank you for visiting our website which covers about 1 In 80 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.