11 Is What Percent Of 60
Treneri
May 15, 2025 · 5 min read
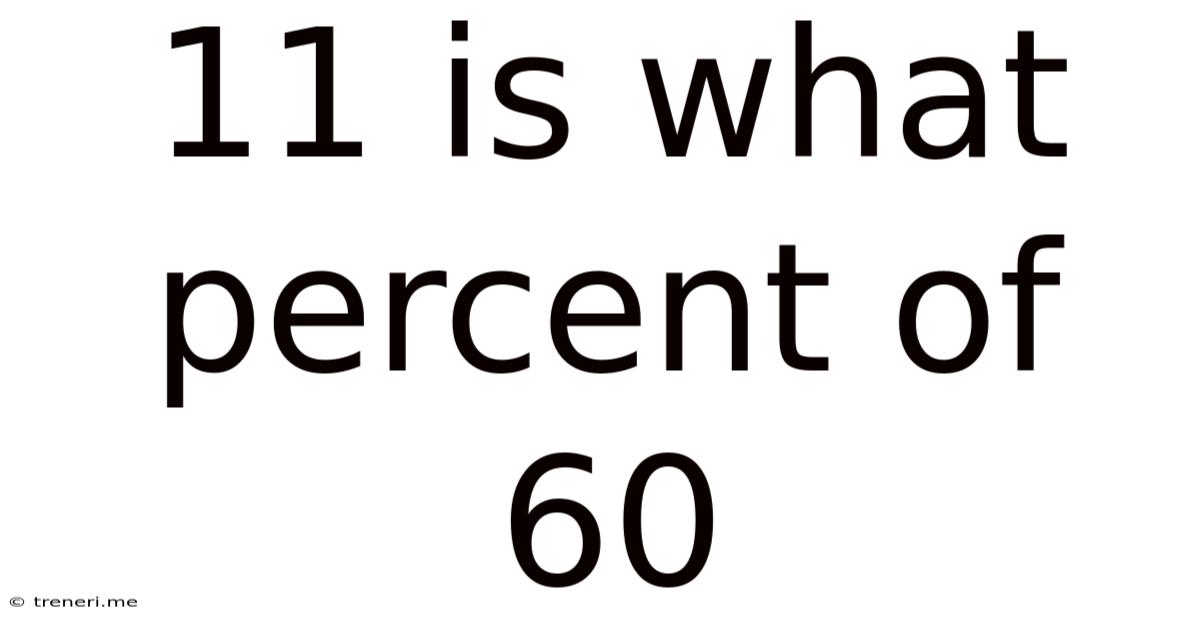
Table of Contents
11 is What Percent of 60? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday finances to complex scientific analyses. This article delves deep into the calculation of "11 is what percent of 60?", providing not just the answer but a thorough explanation of the process, various methods to solve it, and real-world applications. We'll also explore related percentage concepts and offer practical tips for mastering percentage calculations.
Understanding Percentages: The Basics
Before tackling the specific problem, let's establish a strong foundation in percentage concepts. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" signifies "per cent," meaning "out of one hundred." For example, 50% represents 50 out of 100, or 50/100, which simplifies to 1/2.
The fundamental formula for calculating percentages is:
(Part / Whole) * 100% = Percentage
Where:
- Part: The specific amount you are considering (in our case, 11).
- Whole: The total amount you are referencing (in our case, 60).
- Percentage: The resulting value expressed as a percentage.
Calculating "11 is What Percent of 60?"
Now, let's apply this formula to our problem: "11 is what percent of 60?"
-
Identify the parts: The part is 11, and the whole is 60.
-
Apply the formula: (11 / 60) * 100% = Percentage
-
Calculate the fraction: 11 divided by 60 equals approximately 0.1833
-
Multiply by 100: 0.1833 * 100% = 18.33%
Therefore, 11 is approximately 18.33% of 60.
Alternative Methods for Calculating Percentages
While the fundamental formula is efficient, alternative methods can be equally useful, especially depending on the complexity of the problem and your preferred approach.
Method 2: Using Proportions
Proportions offer a visual and intuitive way to solve percentage problems. We set up a proportion:
11/60 = x/100
Where 'x' represents the unknown percentage. Cross-multiplying gives:
60x = 1100
Solving for 'x':
x = 1100 / 60 = 18.33%
This method clearly shows the relationship between the parts and the whole.
Method 3: Using a Calculator
Most calculators have a percentage function (%) that simplifies the process significantly. Simply enter 11 ÷ 60 =, then multiply by 100. The calculator will directly provide the percentage value. This is the quickest method for most calculations.
Real-World Applications of Percentage Calculations
Percentage calculations are invaluable in a wide range of scenarios, including:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage computations. Understanding percentages is crucial for managing personal finances effectively. For instance, determining the percentage increase or decrease in your savings account balance, or calculating the percentage of your income allocated to different expenses.
-
Science: In scientific studies, percentages are frequently used to represent data, such as the percentage of a population with a specific characteristic or the percentage change in a measured variable over time.
-
Business: Businesses use percentages to analyze sales figures, track market share, assess profitability, and make informed decisions regarding pricing, marketing, and investment. For example, calculating the percentage of sales attributed to a specific product line or analyzing the percentage change in customer satisfaction scores.
-
Education: Grades are often expressed as percentages, reflecting a student's performance on assessments and assignments. Understanding percentages is vital for students to track their progress and evaluate their academic standing.
-
Everyday Life: We encounter percentages in everyday situations, such as calculating tips at restaurants, understanding sale discounts, figuring out the nutritional content of food, or interpreting statistical information in news reports.
Expanding on Percentage Concepts: Related Calculations
Understanding percentages allows you to tackle related calculations, such as:
-
Finding the whole: If you know the percentage and the part, you can calculate the whole. For example, if 25% of a number is 10, you can find the whole number by setting up the equation: 0.25 * x = 10, where x is the whole number.
-
Finding the part: If you know the percentage and the whole, you can easily determine the part. For instance, finding 30% of 200 involves calculating 0.30 * 200.
-
Percentage change: This measures the relative change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100%. This is useful for tracking growth or decline in various aspects of life, from the size of your investments to the number of followers on social media.
-
Percentage increase/decrease: These calculations demonstrate the magnitude of a change as a percentage of the original value. A percentage increase shows growth, while a percentage decrease indicates shrinkage. For example, if a product initially priced at $100 is now $120, the percentage increase is 20%.
Tips for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations. Try working through different types of problems to solidify your understanding.
-
Understand the concepts: Don't just memorize formulas; strive to understand the underlying concepts of percentages, ratios, and proportions.
-
Use different methods: Experiment with different approaches to solving percentage problems to find the method that works best for you.
-
Utilize online resources: There are numerous online resources and tools that can help you practice and improve your skills in percentage calculations.
-
Break down complex problems: If faced with a complex percentage problem, break it down into smaller, manageable steps.
Conclusion: Mastering the Percentage Power
This comprehensive guide has explored the calculation of "11 is what percent of 60?" in detail, providing not only the answer (approximately 18.33%) but also a deep dive into the underlying principles and methods involved. We've examined different approaches, highlighted real-world applications, and explored related percentage concepts. By consistently applying these techniques and practicing regularly, you can master percentage calculations and utilize this fundamental skill across various aspects of your life, both personal and professional. Remember, consistent practice is key to building fluency and confidence in handling percentages effectively.
Latest Posts
Latest Posts
-
What Is The Least Common Multiple Of 11 And 5
May 15, 2025
-
How Does Eu Shoe Size Work
May 15, 2025
-
What Is The Value Of X To The Nearest Hundredth
May 15, 2025
-
How Many Pints Equal 1 2 Gallon
May 15, 2025
-
Round 43 To The Nearest Hundred
May 15, 2025
Related Post
Thank you for visiting our website which covers about 11 Is What Percent Of 60 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.