12 Is What Percent Of 400
Treneri
May 11, 2025 · 5 min read
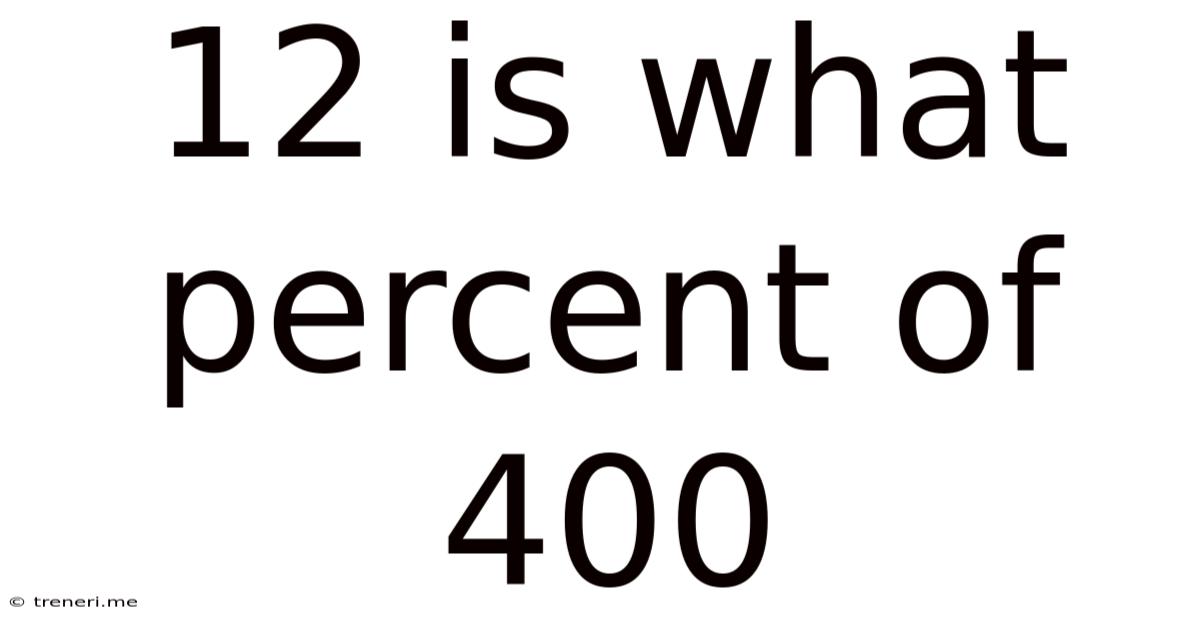
Table of Contents
12 is What Percent of 400? A Deep Dive into Percentage Calculations
Finding percentages is a fundamental skill in math with widespread applications in everyday life, from calculating discounts and taxes to understanding statistics and financial reports. This article will not only answer the question "12 is what percent of 400?" but also provide a comprehensive guide to understanding percentage calculations, offering various methods and exploring practical examples.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of 100" (per centum in Latin). For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Understanding this fundamental concept is crucial for solving percentage problems.
Method 1: Using the Percentage Formula
The most straightforward method to find what percent 12 is of 400 is using the basic percentage formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 12 (the number we want to express as a percentage)
- Whole: 400 (the total number)
Substituting these values into the formula:
(12 / 400) x 100% = 3%
Therefore, 12 is 3% of 400.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can set up a proportion to solve for the unknown percentage (x):
12/400 = x/100
To solve for x, we cross-multiply:
12 x 100 = 400x
1200 = 400x
x = 1200 / 400
x = 3
Therefore, x = 3%. This method reinforces the understanding of the relationship between fractions and percentages.
Method 3: Using Decimal Conversion
This method involves converting the fraction into a decimal and then multiplying by 100%.
First, express the ratio as a decimal:
12 / 400 = 0.03
Then, multiply the decimal by 100% to obtain the percentage:
0.03 x 100% = 3%
This method highlights the connection between decimals and percentages and can be particularly useful when working with calculators.
Practical Applications: Real-World Examples
Understanding percentage calculations is essential in various real-world scenarios. Here are a few examples:
-
Discounts: A store offers a 20% discount on an item originally priced at $100. To calculate the discount amount, you'd find 20% of $100: (20/100) x $100 = $20. The final price would be $100 - $20 = $80.
-
Taxes: If the sales tax is 6%, and you purchase an item for $50, the tax amount would be (6/100) x $50 = $3. The total cost, including tax, would be $50 + $3 = $53.
-
Interest Rates: If you deposit $1000 into a savings account with a 2% annual interest rate, you'll earn (2/100) x $1000 = $20 in interest after one year.
-
Grade Calculation: If you scored 45 out of 50 on a test, your percentage score is (45/50) x 100% = 90%.
-
Statistical Analysis: Percentages are widely used in data representation and analysis to express proportions and trends. For example, expressing the percentage of people who prefer a certain brand or the percentage of respondents who agree with a particular statement in a survey.
-
Financial Statements: Financial reports extensively use percentages to show ratios like profit margins, debt-to-equity ratios, and return on investment (ROI), providing critical insights into a company's financial health.
Advanced Percentage Calculations: Finding the Whole or the Part
While the question "12 is what percent of 400?" focuses on finding the percentage, percentage calculations can also involve finding the whole or the part.
Finding the Whole: If you know the percentage and the part, you can calculate the whole. For instance:
- 15 is 25% of what number?
We can set up the equation:
15/x = 25/100
Cross-multiplying and solving for x:
1500 = 25x
x = 1500 / 25
x = 60
Therefore, 15 is 25% of 60.
Finding the Part: If you know the percentage and the whole, you can calculate the part. For example:
- What is 10% of 200?
This is a straightforward calculation:
(10/100) x 200 = 20
Therefore, 10% of 200 is 20.
Tips and Tricks for Efficient Percentage Calculations
-
Use a Calculator: For more complex calculations, a calculator can significantly simplify the process. Many calculators have a percentage function (%) that automates the calculation.
-
Mental Math: For simpler percentages like 10%, 25%, 50%, and 75%, you can often perform mental calculations. For example, 10% of a number is simply moving the decimal point one place to the left.
-
Working with Fractions: Understanding the relationship between fractions and percentages is beneficial. Converting percentages to fractions can sometimes simplify calculations.
-
Practice Regularly: The key to mastering percentage calculations is consistent practice. Work through various problems and real-world examples to build confidence and proficiency.
Conclusion: Mastering Percentages for Everyday Success
Understanding percentages is a vital skill with far-reaching applications in many aspects of life. By mastering the different methods for calculating percentages, and by practicing regularly, you'll be well-equipped to handle a wide range of percentage-related problems. Remember, the fundamental formula (Part / Whole) x 100% = Percentage is your key to unlocking the world of percentages, whether you're calculating discounts, taxes, interest rates, or analyzing data. The ability to quickly and accurately calculate percentages will significantly improve your problem-solving skills and empower you to make informed decisions in various situations. So, keep practicing, and you'll soon find that percentages are less daunting than they first appear!
Latest Posts
Latest Posts
-
How Much Square Footage Per Chicken
May 11, 2025
-
3 10 Divided By 2 5
May 11, 2025
-
One Astronomical Unit Equals Blank Million Miles
May 11, 2025
-
Cuantos Dias Pasaron Desde 1 De Enero Hasta Hoy
May 11, 2025
-
1 Xicara De Farinha Quantas Gramas
May 11, 2025
Related Post
Thank you for visiting our website which covers about 12 Is What Percent Of 400 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.