2311 Divided By 20 With Remainder
Treneri
May 11, 2025 · 4 min read
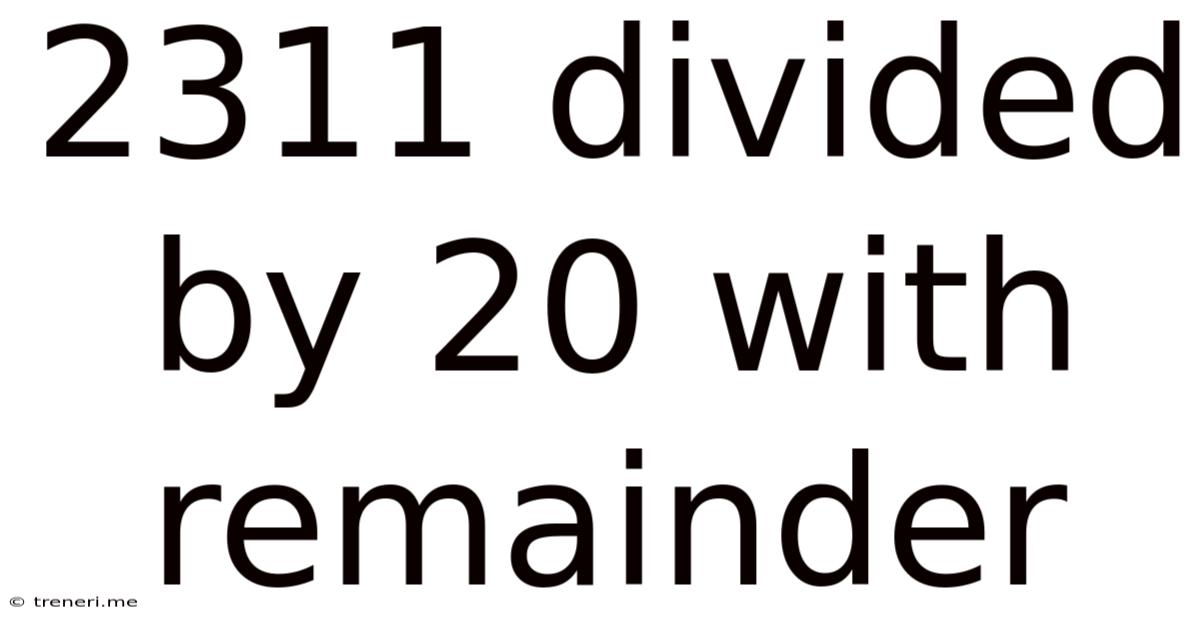
Table of Contents
2311 Divided by 20: A Deep Dive into Division with Remainders
This article explores the seemingly simple problem of dividing 2311 by 20, but delves much deeper than just finding the answer. We'll unpack the process, explore different methods of solving it, discuss the significance of the remainder, and even examine its applications in various fields. Understanding division with remainders is fundamental to mathematics and has practical implications across numerous disciplines.
Understanding Division and Remainders
Division, at its core, is the process of splitting a quantity into equal parts. When we divide 2311 by 20, we're essentially asking: "How many times does 20 fit completely into 2311, and what's left over?"
The number being divided (2311) is called the dividend. The number we're dividing by (20) is the divisor. The result of the division (the number of times the divisor fits into the dividend) is the quotient. And finally, the amount left over after the division is the remainder.
In mathematical notation, we can represent division with a remainder as follows:
Dividend = (Divisor × Quotient) + Remainder
In our case: 2311 = (20 × Quotient) + Remainder
Calculating 2311 Divided by 20
Let's find the quotient and remainder using several methods:
Method 1: Long Division
The traditional long division method is a reliable way to calculate the quotient and remainder.
115
20 | 2311
-20
---
31
-20
---
111
-100
---
11
Following the long division process, we find that 20 goes into 2311 115 times with a remainder of 11.
Method 2: Repeated Subtraction
This method involves repeatedly subtracting the divisor (20) from the dividend (2311) until the result is less than the divisor. The number of subtractions is the quotient, and the final result is the remainder. While less efficient for larger numbers, this method helps visualize the concept of division.
(This method is best demonstrated visually, but the principle can be understood by repeatedly subtracting 20 from 2311 until a number less than 20 remains. The number of times 20 is subtracted is the quotient, and the remaining number is the remainder.)
This process would result in the same answer: a quotient of 115 and a remainder of 11.
Method 3: Using a Calculator
Most calculators will handle this directly. Simply input 2311 ÷ 20. Many calculators will show the answer as 115.55. This decimal part represents the remainder as a fraction of the divisor. To find the integer remainder, multiply the decimal part (.55) by the divisor (20): 0.55 * 20 = 11.
The Significance of the Remainder
The remainder isn't just a leftover; it's a crucial piece of information. It tells us that 2311 cannot be perfectly divided into groups of 20. This has practical implications in many real-world situations:
-
Distribution: Imagine dividing 2311 items into boxes that hold 20 items each. You would fill 115 boxes completely and have 11 items left over.
-
Scheduling: If a task takes 20 minutes, and you have 2311 minutes available, you can complete the task 115 times, with 11 minutes remaining.
-
Modular Arithmetic: In computer science and cryptography, the remainder is essential in modular arithmetic, which forms the basis of many security protocols. The remainder when dividing by a number (the modulus) is used to represent numbers in a specific range.
-
Measurement: When measuring lengths or weights, the remainder often represents the excess beyond a whole unit.
Applications in Various Fields
The concept of division with remainders is fundamental in several fields:
-
Computer Science: Binary representation of numbers, hashing algorithms, and error detection codes all rely heavily on modular arithmetic and the concept of remainders.
-
Engineering: Calculations involving gear ratios, material cutting, and resource allocation often use division with remainders to account for incomplete divisions.
-
Finance: Dividing profits amongst shareholders, calculating interest payments, and distributing assets frequently involve remainders.
-
Physics: Many physical phenomena involve cyclical patterns, where remainders are crucial in understanding periodicity and related concepts.
Expanding on the Concept: Different Divisors and Dividends
While we've focused on 2311 divided by 20, the principles remain the same for other dividend-divisor pairs. The method of calculation will be the same, whether you're working with larger or smaller numbers, or even negative numbers. Understanding how the remainder behaves with different divisors is a crucial part of grasping the broader concept of division.
Consider these examples:
- 2311 divided by 10: The quotient would be 231 with a remainder of 1.
- 2311 divided by 5: The quotient would be 462 with a remainder of 1.
- 2311 divided by 100: The quotient would be 23 with a remainder of 11.
Notice how changing the divisor significantly impacts both the quotient and the remainder.
Conclusion: Mastering Division with Remainders
The seemingly simple calculation of 2311 divided by 20 reveals a rich mathematical concept with broad applications. Beyond the straightforward answer of a quotient of 115 and a remainder of 11, we've explored different methods of calculating this division, examined the significance of the remainder, and highlighted its relevance in various fields. A firm grasp of division with remainders is crucial for anyone pursuing studies or careers involving mathematics, computer science, engineering, or any field requiring precise calculations and the understanding of incomplete divisions. The remainder, far from being a trivial leftover, is a vital piece of information that shapes our understanding of numerical relationships and their practical applications in the real world.
Latest Posts
Latest Posts
-
Sales Tax On Car In Missouri
May 12, 2025
-
What Is The Greatest Common Factor Of 72 And 90
May 12, 2025
-
How Many Months In 70 Years
May 12, 2025
-
How Many Square Feet In 14x14
May 12, 2025
-
How Long Is 84 Months In Years
May 12, 2025
Related Post
Thank you for visiting our website which covers about 2311 Divided By 20 With Remainder . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.