3 Out Of 18 As A Percentage
Treneri
May 14, 2025 · 4 min read
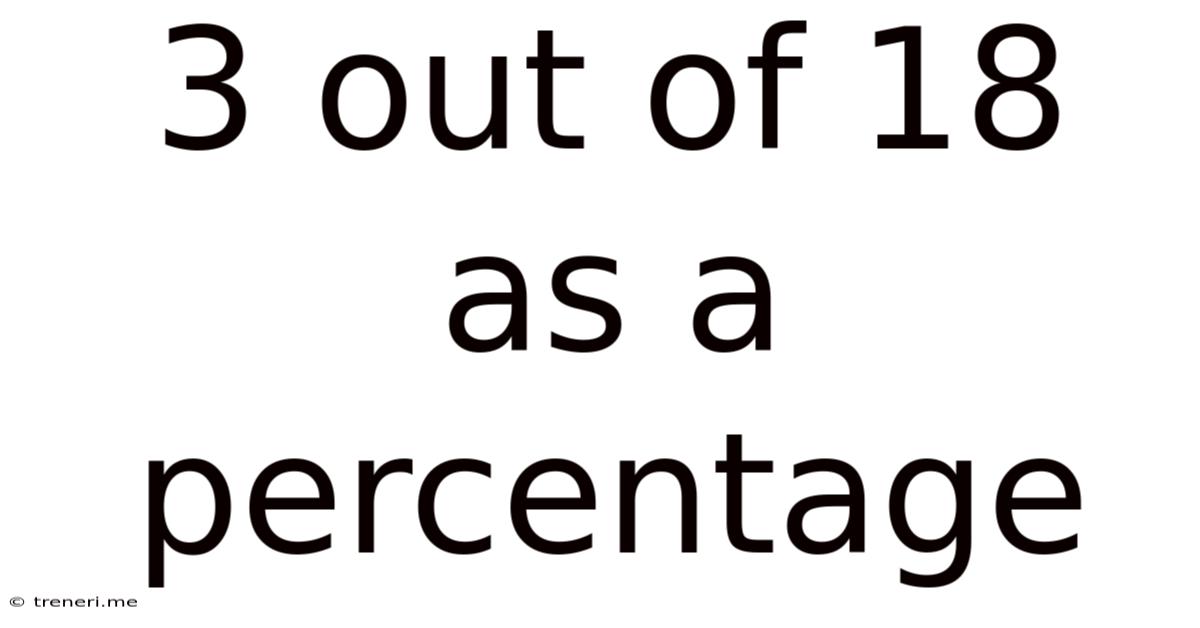
Table of Contents
3 Out of 18 as a Percentage: A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday finances to complex scientific calculations. This comprehensive guide will delve into how to calculate "3 out of 18 as a percentage," providing various methods, clarifying common misconceptions, and exploring practical applications. We'll also look at the broader context of percentage calculations and how to approach similar problems efficiently.
Understanding the Fundamentals of Percentages
Before diving into the specific calculation, let's refresh our understanding of percentages. A percentage represents a fraction of 100. The term "percent" literally means "per hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Key Terms:
- Numerator: The top number in a fraction (in this case, 3). It represents the part.
- Denominator: The bottom number in a fraction (in this case, 18). It represents the whole.
- Percentage: The fraction expressed as a value out of 100.
Method 1: Using the Fraction Method
The most straightforward approach to calculating "3 out of 18 as a percentage" involves converting the given numbers into a fraction and then converting that fraction into a percentage.
Steps:
-
Form a Fraction: Express "3 out of 18" as a fraction: 3/18.
-
Simplify the Fraction (Optional): Simplifying the fraction makes the subsequent calculation easier. We can divide both the numerator and the denominator by their greatest common divisor (GCD), which is 3 in this case. This simplifies the fraction to 1/6.
-
Convert to Decimal: Divide the numerator by the denominator: 1 ÷ 6 ≈ 0.1667.
-
Convert to Percentage: Multiply the decimal by 100: 0.1667 × 100 = 16.67%.
Therefore, 3 out of 18 is approximately 16.67%.
Method 2: Using the Proportion Method
This method utilizes proportions to directly find the percentage. We set up a proportion where we know one ratio (3/18) and want to find an equivalent ratio with a denominator of 100.
Steps:
-
Set up a Proportion: We can set up the proportion as follows:
3/18 = x/100
-
Cross-Multiply: Cross-multiply to solve for x:
18x = 300
-
Solve for x: Divide both sides by 18:
x = 300/18 ≈ 16.67
Therefore, x ≈ 16.67, meaning 3 out of 18 is approximately 16.67%.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process significantly. Simply enter 3 ÷ 18 and then multiply the result by 100. The calculator will directly display the percentage. This method is particularly useful for more complex calculations.
Understanding the Result: 16.67%
The result, 16.67%, signifies that 3 represents approximately 16.67% of the total value of 18. This percentage can be interpreted in various contexts depending on the scenario. For example, if 3 out of 18 students passed an exam, it indicates that approximately 16.67% of the students were successful.
Practical Applications of Percentage Calculations
Percentage calculations are ubiquitous in daily life and various professional settings. Here are some examples:
- Finance: Calculating interest rates, discounts, tax rates, profit margins, and investment returns.
- Statistics: Analyzing data, determining probabilities, and presenting results in a clear and concise manner.
- Science: Expressing concentrations, efficiencies, and error margins in experiments.
- Retail: Calculating discounts, sales tax, and markups on products.
- Education: Assessing student performance, analyzing test scores, and tracking progress.
Common Mistakes to Avoid
While percentage calculations are relatively straightforward, common mistakes can lead to inaccurate results. Here are some points to keep in mind:
- Incorrect Fraction Formation: Ensure the correct values are placed in the numerator and the denominator. The part goes on top, and the whole goes on the bottom.
- Rounding Errors: Rounding off intermediate results too early can lead to significant inaccuracies in the final percentage. It is best to retain as many decimal places as possible until the final result.
- Misinterpreting the Percentage: Understanding the context of the percentage is crucial for accurate interpretation. A 16.67% success rate might be considered low in some contexts and high in others.
Expanding on Percentage Calculations: More Complex Scenarios
While this article focuses on a simple calculation, the principles can be applied to more complex scenarios. For example:
- Calculating percentage increase or decrease: This involves comparing two values and determining the percentage change.
- Calculating percentages of percentages: This involves applying multiple percentage calculations sequentially.
- Working with percentages in data analysis: Using percentages to interpret and present data from larger datasets.
Conclusion: Mastering Percentage Calculations
The ability to calculate percentages is a valuable skill with applications in many areas of life. By understanding the fundamental concepts and applying the methods described in this guide, you can accurately and confidently calculate percentages, even in more complex scenarios. Remember to pay close attention to detail, avoid common mistakes, and always consider the context of your results for accurate interpretation. Practice is key to mastering percentage calculations, so try applying these methods to different problems and scenarios to further enhance your understanding and build your confidence. The more you practice, the more intuitive and efficient these calculations will become.
Latest Posts
Latest Posts
-
How Much Days Until June 1
May 14, 2025
-
How Many Quarts Are In 28 Cups
May 14, 2025
-
How To Calculate Z Score On Calculator
May 14, 2025
-
12x20 Is How Many Square Feet
May 14, 2025
-
What Grade Is 11 Out Of 20
May 14, 2025
Related Post
Thank you for visiting our website which covers about 3 Out Of 18 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.