3 To The Third Power Equals
Treneri
May 13, 2025 · 5 min read
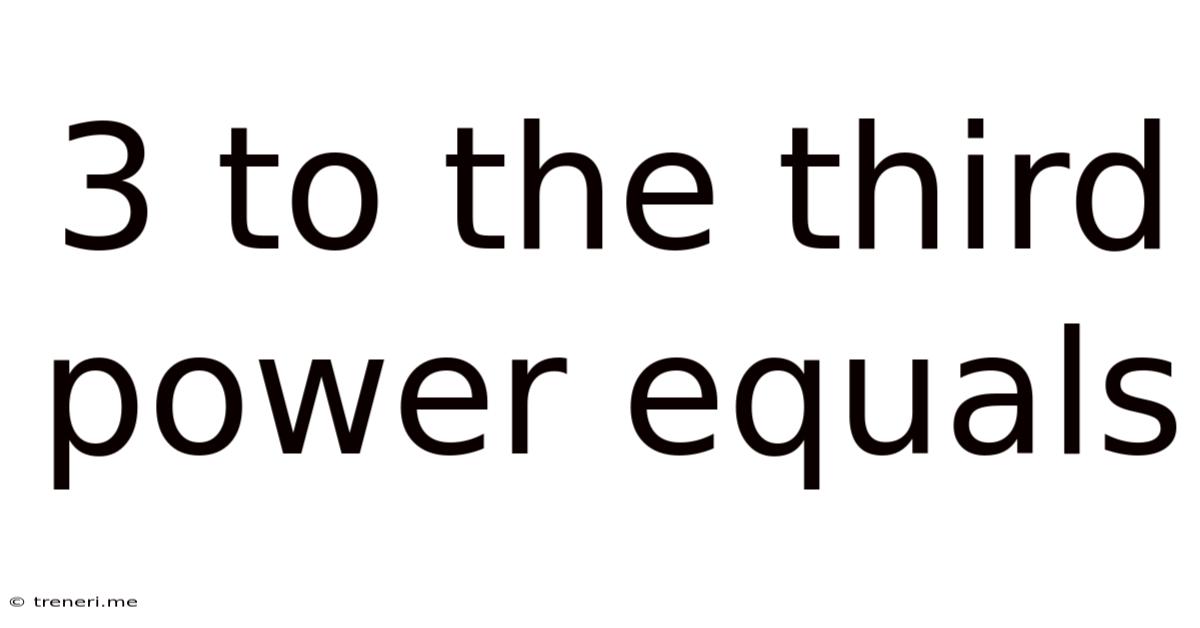
Table of Contents
3 to the Third Power Equals: Unpacking the Concept of Cubing
The seemingly simple statement, "3 to the third power equals," opens a door to a vast world of mathematical concepts. Understanding this seemingly basic calculation unlocks a deeper comprehension of exponents, their applications in various fields, and their significance in higher-level mathematics. This article will delve into the meaning of "3 to the third power," explore its practical applications, and examine related mathematical concepts.
Understanding Exponents and Powers
Before we delve into the specific calculation of 3 to the third power, it's crucial to understand the fundamental concept of exponents. An exponent, also known as a power or index, is a small number written slightly above and to the right of a base number. It indicates how many times the base number is multiplied by itself.
For example, in the expression 3³, the number 3 is the base, and the number 3 (the exponent) signifies that the base (3) should be multiplied by itself three times. Therefore, 3³ is equivalent to 3 * 3 * 3.
The Language of Exponents
Understanding the terminology is vital. We often express exponents using specific language:
- Base: The number being multiplied (in 3³, the base is 3).
- Exponent/Power/Index: The number indicating how many times the base is multiplied by itself (in 3³, the exponent is 3).
- Cubed: When an exponent is 3, we often say the number is "cubed." This refers to the geometric concept of a cube's volume, which is calculated by multiplying its length, width, and height (all three dimensions). This visual connection helps solidify understanding.
Calculating 3 to the Third Power
Now, let's tackle the core question: What does 3 to the third power equal? As explained earlier, 3³ means 3 * 3 * 3. Performing this simple multiplication:
3 * 3 = 9 9 * 3 = 27
Therefore, 3 to the third power equals 27.
Different Representations
While we've established that 3³ = 27, it's useful to understand that this mathematical concept can be expressed in various ways, all representing the same value:
- 3 to the power of 3: This is a more descriptive way of stating the problem.
- 3 cubed: This concise phrase highlights the exponent's value of 3.
- 27: This is the final numerical result of the calculation.
Applications of Cubing and Exponents
The concept of cubing, and exponents in general, isn't confined to theoretical mathematics; it has widespread practical applications across numerous fields:
1. Geometry and Volume Calculations
As mentioned earlier, the term "cubed" directly relates to calculating the volume of a cube. If a cube has sides of length 3 units, its volume is 3³ cubic units, or 27 cubic units. This principle extends to other three-dimensional shapes, where cubic functions frequently appear in volume formulas. Understanding exponents is essential for accurate volume calculations.
2. Physics and Engineering
Exponents are pervasive in physics and engineering, appearing in formulas related to:
- Motion: Calculations involving acceleration, velocity, and displacement often utilize exponents.
- Electricity: Ohm's Law (V = IR) involves exponents in more complex circuit analysis.
- Fluid dynamics: Formulas describing fluid flow and pressure frequently involve exponents.
- Optics: Calculations related to light intensity and wave propagation often involve exponents.
3. Computer Science and Programming
Exponents play a significant role in computer science, particularly in:
- Big O Notation: Used to describe the efficiency of algorithms, big O notation employs exponents to express how an algorithm's runtime scales with input size.
- Data Structures: The performance of certain data structures, such as trees and graphs, is analyzed using exponential functions.
- Cryptography: Many cryptographic algorithms rely heavily on exponentiation for encryption and decryption.
4. Finance and Economics
Exponential functions are crucial in finance and economics, primarily for:
- Compound Interest: Calculating compound interest involves exponential growth, where the interest earned is added to the principal, generating even more interest over time.
- Investment Growth: Modeling the growth of investments, particularly over longer periods, typically utilizes exponential functions.
- Economic Models: Numerous economic models utilize exponential functions to represent growth rates, decay rates, and other dynamic processes.
5. Biology and Medicine
Exponential growth and decay are observed in biological systems:
- Population Growth: Modeling population growth in ideal conditions often uses exponential functions.
- Radioactive Decay: The decay rate of radioactive isotopes is described by exponential functions, crucial for medical imaging (PET scans) and radiation therapy.
- Drug Metabolism: The rate at which the body metabolizes drugs can be modeled using exponential functions.
Expanding on Exponents: Beyond Cubing
While this article focuses on 3³, it's important to contextualize this within the broader realm of exponents. The rules governing exponents are crucial for mastering more complex mathematical concepts:
1. Product Rule: aᵐ * aⁿ = aᵐ⁺ⁿ
When multiplying two terms with the same base, you add the exponents. For example: 3² * 3⁴ = 3⁶ = 729
2. Quotient Rule: aᵐ / aⁿ = aᵐ⁻ⁿ
When dividing two terms with the same base, you subtract the exponents. For example: 3⁵ / 3² = 3³ = 27
3. Power Rule: (aᵐ)ⁿ = aᵐⁿ
When raising a term with an exponent to another power, you multiply the exponents. For example: (3²)³ = 3⁶ = 729
4. Zero Exponent: a⁰ = 1
Any non-zero number raised to the power of zero equals 1. For example: 3⁰ = 1
5. Negative Exponents: a⁻ⁿ = 1/aⁿ
A negative exponent indicates the reciprocal of the base raised to the positive exponent. For example: 3⁻² = 1/3² = 1/9
Understanding these rules allows for the manipulation and simplification of exponential expressions, crucial for solving complex mathematical problems across various disciplines.
Conclusion: The Significance of 3 Cubed and Beyond
"3 to the third power equals 27" is more than just a simple calculation; it's a gateway to understanding exponents, their properties, and their widespread applications. From calculating volumes to modeling complex phenomena, exponents are fundamental mathematical tools that underpin numerous fields of study and practical applications. By mastering the concepts discussed here, you equip yourself with powerful tools for problem-solving and a deeper appreciation of the interconnectedness of mathematics and the real world. Further exploration of exponential functions, logarithmic functions, and their interrelationships will only deepen this understanding and open doors to even more advanced mathematical concepts.
Latest Posts
Latest Posts
-
What Is 60 Percent Of 100
May 13, 2025
-
How Many Oz Is 2 5 Lbs
May 13, 2025
-
Area Of A Circle With A Diameter Of 12
May 13, 2025
-
How To Do Diamond Math Problems
May 13, 2025
-
What Is The Greatest Common Factor Of 72 And 36
May 13, 2025
Related Post
Thank you for visiting our website which covers about 3 To The Third Power Equals . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.