45 7 As A Mixed Number
Treneri
May 14, 2025 · 4 min read
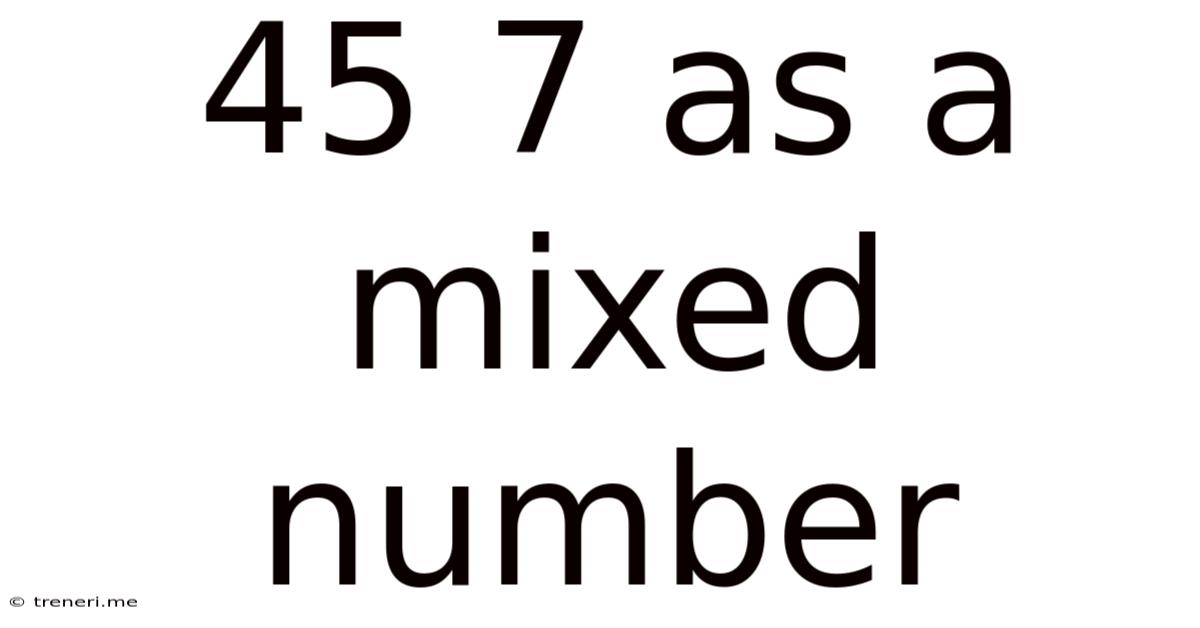
Table of Contents
45/7 as a Mixed Number: A Comprehensive Guide
Converting improper fractions, like 45/7, into mixed numbers is a fundamental skill in arithmetic. This comprehensive guide will not only show you how to convert 45/7 to a mixed number but also delve into the underlying concepts, provide alternative methods, and explore real-world applications. We'll also cover some common mistakes to avoid and offer practice problems to solidify your understanding.
Understanding Improper Fractions and Mixed Numbers
Before we dive into the conversion, let's clarify the definitions:
-
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). 45/7 is an example of an improper fraction because 45 > 7.
-
Mixed Number: A mixed number consists of a whole number and a proper fraction. A proper fraction is a fraction where the numerator is less than the denominator. For example, 6 ¾ is a mixed number.
The conversion process essentially involves determining how many times the denominator goes into the numerator and expressing the remainder as a fraction.
Converting 45/7 to a Mixed Number: The Standard Method
The most common method for converting an improper fraction to a mixed number involves division:
-
Divide the numerator by the denominator: Divide 45 by 7. This gives us a quotient (the whole number part) and a remainder.
45 ÷ 7 = 6 with a remainder of 3
-
Write the quotient as the whole number: The quotient, 6, becomes the whole number part of our mixed number.
-
Write the remainder as the numerator of the fraction: The remainder, 3, becomes the numerator of the fraction.
-
Keep the original denominator: The denominator remains the same, 7.
Therefore, 45/7 as a mixed number is 6 ³⁄₇.
Alternative Methods: Visualizing the Conversion
While the division method is efficient, visualizing the process can enhance understanding, especially for beginners. Consider these approaches:
-
Using Fraction Circles or Bars: Imagine seven equal parts making a whole. To represent 45/7, you'd need six full sets of seven parts (6 x 7 = 42) and three additional parts. This visually represents 6 wholes and 3/7, leading to the mixed number 6 ³⁄₇.
-
Repeated Subtraction: Subtract the denominator (7) from the numerator (45) repeatedly until you reach a number less than the denominator. Count how many times you subtracted (this is your whole number) and the remaining number is your new numerator.
45 - 7 = 38 38 - 7 = 31 31 - 7 = 24 24 - 7 = 17 17 - 7 = 10 10 - 7 = 3
You subtracted 7 six times, so the whole number is 6. The remainder is 3, giving you the mixed number 6 ³⁄₇. This method is less efficient for larger numbers but helpful for conceptual understanding.
Common Mistakes to Avoid
Several common errors can occur during the conversion process:
-
Incorrect Division: Ensure you perform the division correctly. A simple mistake in division will lead to an incorrect mixed number. Double-check your work!
-
Forgetting the Remainder: The remainder is crucial. Ignoring it will result in an incomplete and inaccurate mixed number.
-
Incorrect Placement of Numbers: Make sure the whole number, numerator, and denominator are placed correctly in the mixed number format.
-
Not Simplifying the Fraction: Always simplify the fractional part of the mixed number if possible. For instance, if you had obtained 6 ⁶⁄₁₄, you would simplify the fraction to 6 ³⁄₇.
Real-World Applications of Mixed Numbers
Mixed numbers are prevalent in various real-world situations:
-
Measurement: Measuring ingredients in cooking (e.g., 2 ½ cups of flour), lengths (e.g., 3 ¾ inches of wood), or weights (e.g., 5 ²/₃ pounds of sugar).
-
Time: Representing durations (e.g., 1 ¼ hours, 2 ⅔ minutes).
-
Finance: Dealing with fractional shares of stocks or representing portions of a budget.
-
Construction and Engineering: Precise measurements in building projects and design specifications.
-
Data Analysis: Presenting data in a more easily understandable format involving fractions and whole numbers.
Practice Problems
To reinforce your understanding, try converting these improper fractions to mixed numbers:
- 23/5
- 37/8
- 51/6
- 92/11
- 105/12
Solutions:
- 4 ¾
- 4 ⁵⁄₈
- 8 ½
- 8 ⁴⁄₁₁
- 8 ⁹⁄₁₂ (which simplifies to 8 ¾)
Conclusion: Mastering Mixed Number Conversions
Converting improper fractions like 45/7 to mixed numbers is a crucial skill with widespread applications. By understanding the underlying concepts and employing the methods described, you'll gain confidence and accuracy in performing these conversions. Remember to check your work for common errors and to always simplify the fractional part of your mixed number when possible. Practice makes perfect, so utilize the provided practice problems and seek out additional exercises to solidify your mastery. The ability to seamlessly navigate between improper fractions and mixed numbers will significantly enhance your mathematical proficiency and ability to solve real-world problems.
Latest Posts
Latest Posts
-
1 Cucharada De Leche En Polvo En Gramos
May 14, 2025
-
Write 720 080 In Expanded Form With Exponents
May 14, 2025
-
Cuanto Es Un Mm En Pulgadas
May 14, 2025
-
How To Calculate Current With Power And Voltage
May 14, 2025
-
How Many Lbs Of Sand For Sandbox
May 14, 2025
Related Post
Thank you for visiting our website which covers about 45 7 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.