5 8 As A Mixed Number
Treneri
May 14, 2025 · 5 min read
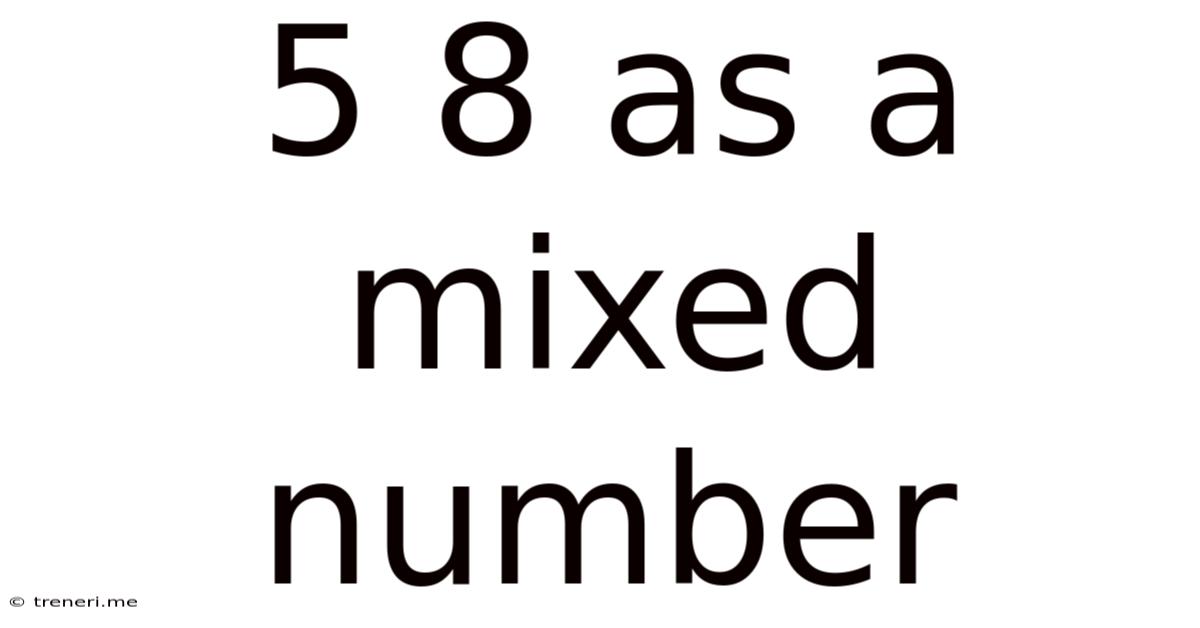
Table of Contents
5/8 as a Mixed Number: A Comprehensive Guide
Understanding fractions is fundamental to mathematics, and converting improper fractions (like 5/8) to mixed numbers is a crucial skill. This comprehensive guide will not only show you how to convert 5/8 to a mixed number but will also delve into the underlying concepts, provide various methods for conversion, and explore the practical applications of this knowledge. We'll even tackle some frequently asked questions to ensure you have a complete grasp of the topic.
What is a Mixed Number?
A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1 ½, 3 ¼, and 2 ⅔ are all mixed numbers. They represent a quantity that is more than one whole unit but less than the next whole number.
Why Convert Improper Fractions to Mixed Numbers?
Improper fractions, where the numerator is greater than or equal to the denominator (like 5/8 is not an improper fraction, but 8/5 is), can be difficult to visualize and work with in certain contexts. Converting them to mixed numbers often makes them easier to understand and use in calculations, especially when dealing with real-world problems involving measurement or quantities.
Why 5/8 Isn't an Improper Fraction and Therefore Doesn't Need Conversion
It's crucial to understand that the fraction 5/8 is not an improper fraction. The numerator (5) is smaller than the denominator (8). Therefore, 5/8 is already a proper fraction, and it cannot be expressed as a mixed number. A mixed number represents a value greater than 1; 5/8 represents a value less than 1. Attempting to convert it would result in an incorrect and meaningless answer.
Understanding Fractions: A Deeper Dive
Before moving on, let's reinforce our understanding of fractions. A fraction represents a part of a whole. The denominator shows the number of equal parts the whole is divided into, and the numerator indicates how many of those parts we're considering.
For example, in the fraction 5/8:
- Denominator (8): Imagine a pie cut into 8 equal slices.
- Numerator (5): You have 5 of those 8 slices.
Working with Proper Fractions Like 5/8
Since 5/8 is a proper fraction, it represents a quantity less than one whole. We can visualize this as having 5 out of 8 equal parts of a whole. This fraction is already in its simplest form, meaning there's no common factor (other than 1) that can divide both the numerator and the denominator. Therefore, no further simplification is possible.
Illustrative Examples of Improper Fraction to Mixed Number Conversion
To further clarify the concept of converting improper fractions to mixed numbers (which, again, is not applicable to 5/8), let's look at some examples:
Example 1: Convert 7/4 to a mixed number.
- Divide the numerator by the denominator: 7 ÷ 4 = 1 with a remainder of 3.
- The whole number part of the mixed number is the quotient: 1
- The fraction part of the mixed number has the remainder as the numerator and the original denominator as the denominator: 3/4
- The mixed number is: 1 ¾
Example 2: Convert 11/3 to a mixed number.
- Divide the numerator by the denominator: 11 ÷ 3 = 3 with a remainder of 2.
- The whole number part: 3
- The fraction part: 2/3
- The mixed number is: 3 ⅔
Practical Applications of Fraction Conversion
Converting fractions (improper fractions to mixed numbers) is crucial in various real-world situations:
- Cooking and Baking: Recipes often call for fractional amounts of ingredients. Converting improper fractions to mixed numbers can make measuring these amounts easier.
- Construction and Engineering: Precise measurements are critical in these fields. Converting fractions helps in accurate calculations and avoids errors.
- Sewing and Tailoring: Patterns and measurements often involve fractions. Converting fractions ensures precise cutting and fitting.
- Data Analysis: Representing data using fractions can be simplified by converting them to mixed numbers for better understanding and presentation.
Frequently Asked Questions (FAQs)
Q1: Can all fractions be converted to mixed numbers?
A1: No. Only improper fractions (where the numerator is greater than or equal to the denominator) can be converted to mixed numbers. Proper fractions, like 5/8, remain as they are.
Q2: What if the fraction is already in its simplest form?
A2: Even if the fraction is in its simplest form, if it's an improper fraction, it can still be converted to a mixed number. The simplification step is done before the conversion to a mixed number.
Q3: How do I convert a mixed number back to an improper fraction?
A3: To convert a mixed number to an improper fraction, multiply the whole number by the denominator, add the numerator, and keep the same denominator. For example, 2 ¾ becomes (2 * 4 + 3) / 4 = 11/4.
Q4: Why is it important to understand fraction conversion?
A4: Understanding fraction conversion is essential for accurate calculations and problem-solving in various fields, improving comprehension of mathematical concepts and facilitating real-world applications.
Conclusion
While the initial question asked about expressing 5/8 as a mixed number, it highlights the importance of understanding the difference between proper and improper fractions. 5/8, being a proper fraction, cannot be expressed as a mixed number. This article provided a comprehensive overview of fractions, explained the process of converting improper fractions to mixed numbers with illustrative examples, and explored the practical applications of this crucial mathematical skill. Remember, a solid grasp of fractions is a cornerstone of mathematical proficiency and problem-solving.
Latest Posts
Related Post
Thank you for visiting our website which covers about 5 8 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.