6 Out Of 24 As A Percentage
Treneri
May 12, 2025 · 5 min read
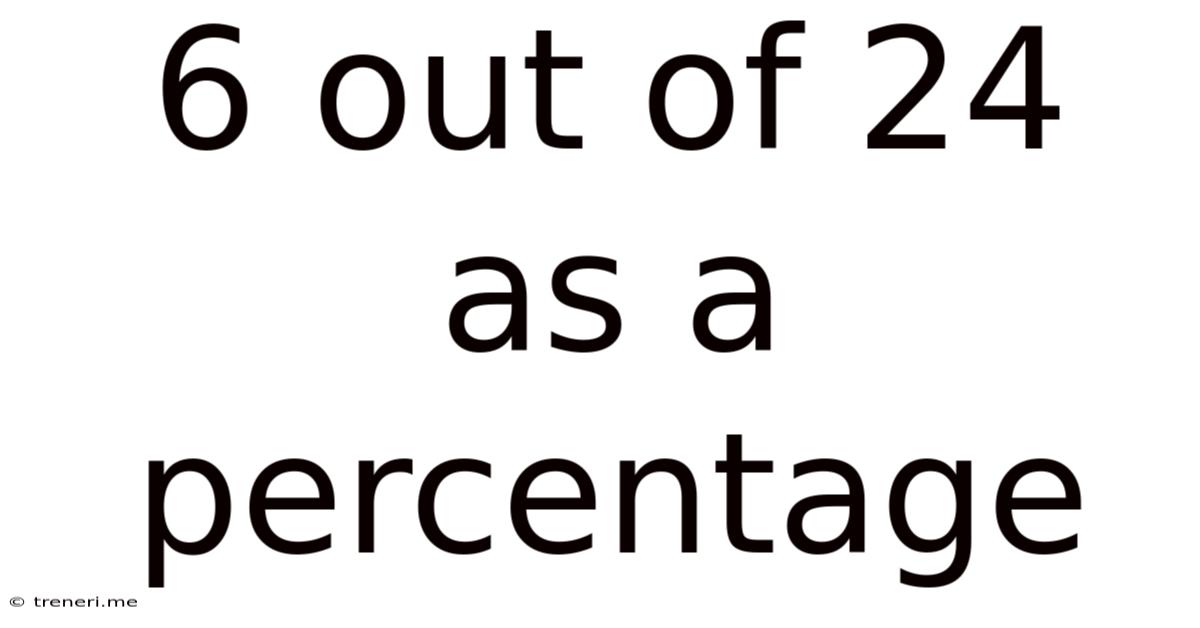
Table of Contents
6 Out of 24 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from everyday finances to complex scientific analyses. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and excelling in various academic and professional settings. This comprehensive guide will delve into the method of calculating "6 out of 24 as a percentage," offering various approaches, explaining the underlying concepts, and providing practical examples. We will also explore the broader applications of percentage calculations and address common misconceptions.
Understanding Percentages
Before jumping into the calculation, let's establish a solid understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" signifies "per cent," meaning "out of one hundred." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Percentages provide a standardized way to compare proportions and ratios, making them incredibly useful for data analysis and representation.
Method 1: Using the Fraction Method
The most straightforward method to calculate "6 out of 24 as a percentage" is to express the given information as a fraction and then convert it into a percentage.
Step 1: Express as a Fraction
The phrase "6 out of 24" can be directly written as the fraction 6/24.
Step 2: Simplify the Fraction
To simplify the fraction, we find the greatest common divisor (GCD) of the numerator (6) and the denominator (24). The GCD of 6 and 24 is 6. Dividing both the numerator and denominator by 6, we get:
6/24 = 1/4
Step 3: Convert the Fraction to a Decimal
To convert the fraction 1/4 into a decimal, we divide the numerator (1) by the denominator (4):
1 ÷ 4 = 0.25
Step 4: Convert the Decimal to a Percentage
To convert the decimal 0.25 into a percentage, we multiply it by 100 and add the "%" symbol:
0.25 × 100 = 25%
Therefore, 6 out of 24 is equal to 25%.
Method 2: Using the Proportion Method
Another approach involves setting up a proportion. This method is particularly useful when dealing with more complex scenarios or when visualizing the relationship between the parts and the whole.
Step 1: Set up the Proportion
We can set up a proportion as follows:
6/24 = x/100
Where 'x' represents the percentage we want to find.
Step 2: Cross-Multiply
Cross-multiplying the proportion, we get:
24x = 600
Step 3: Solve for x
Dividing both sides by 24, we get:
x = 600/24 = 25
Therefore, x = 25%, confirming our result from the previous method.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process. Simply enter 6 ÷ 24 and then multiply the result by 100. The calculator will directly display the answer as 25%. This method is quick and efficient, especially for more intricate calculations.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is vital in numerous real-world scenarios. Here are some examples:
- Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations.
- Statistics: Percentages are fundamental in representing data, conducting statistical analyses, and interpreting survey results. Understanding percentages is crucial for drawing accurate conclusions from data.
- Science: Many scientific concepts and experiments involve calculating percentages, such as expressing concentrations, yields, and error margins.
- Everyday Life: We encounter percentages daily in various contexts, such as calculating tips, understanding sales promotions, and interpreting nutritional information on food labels.
Common Misconceptions about Percentages
Several common misconceptions can lead to errors in percentage calculations. It's crucial to address these to ensure accuracy:
- Confusing Percentage Change with Absolute Change: A 10% increase on a larger number is significantly greater than a 10% increase on a smaller number. Understanding the difference between absolute and relative change is crucial for accurate interpretation.
- Incorrectly Calculating Percentage Increase/Decrease: When calculating a percentage increase or decrease, the base value must be clearly identified. Errors often arise when using the wrong base value for calculation.
- Misinterpreting Percentages in Context: The context in which a percentage is presented is vital for proper interpretation. A percentage alone does not provide a complete picture without additional information.
Expanding on the Concept: Beyond 6 out of 24
While this guide focuses on "6 out of 24," the principles discussed are applicable to any fraction-to-percentage conversion. Let's explore some related examples to solidify your understanding:
- 12 out of 24: This simplifies to 12/24 = 1/2 = 0.5 = 50%
- 3 out of 24: This simplifies to 3/24 = 1/8 = 0.125 = 12.5%
- 18 out of 24: This simplifies to 18/24 = 3/4 = 0.75 = 75%
These examples illustrate how the same fundamental principles can be applied across various fractions. The key is to simplify the fraction and then convert it to a decimal before multiplying by 100 to obtain the percentage.
Conclusion: Mastering Percentage Calculations
Calculating "6 out of 24 as a percentage" – and other similar calculations – is a fundamental skill with broad applications. By understanding the different methods – using fractions, proportions, or calculators – you can confidently tackle percentage calculations in any context. Remember to avoid common misconceptions and always consider the context in which the percentage is presented for accurate interpretation. Mastering percentage calculations empowers you to analyze data effectively, make informed decisions, and excel in various fields. With practice, these calculations will become second nature, enhancing your numerical literacy and problem-solving abilities.
Latest Posts
Latest Posts
-
1991 To 2023 How Many Years
May 13, 2025
-
How To Calculate The Process Capability Index
May 13, 2025
-
How Many Grams Is 29 Ounces
May 13, 2025
-
How Much Oil Is One Stick Of Butter
May 13, 2025
-
Calculate Time With Acceleration And Distance
May 13, 2025
Related Post
Thank you for visiting our website which covers about 6 Out Of 24 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.