8 1/3 As A Mixed Number
Treneri
May 10, 2025 · 6 min read
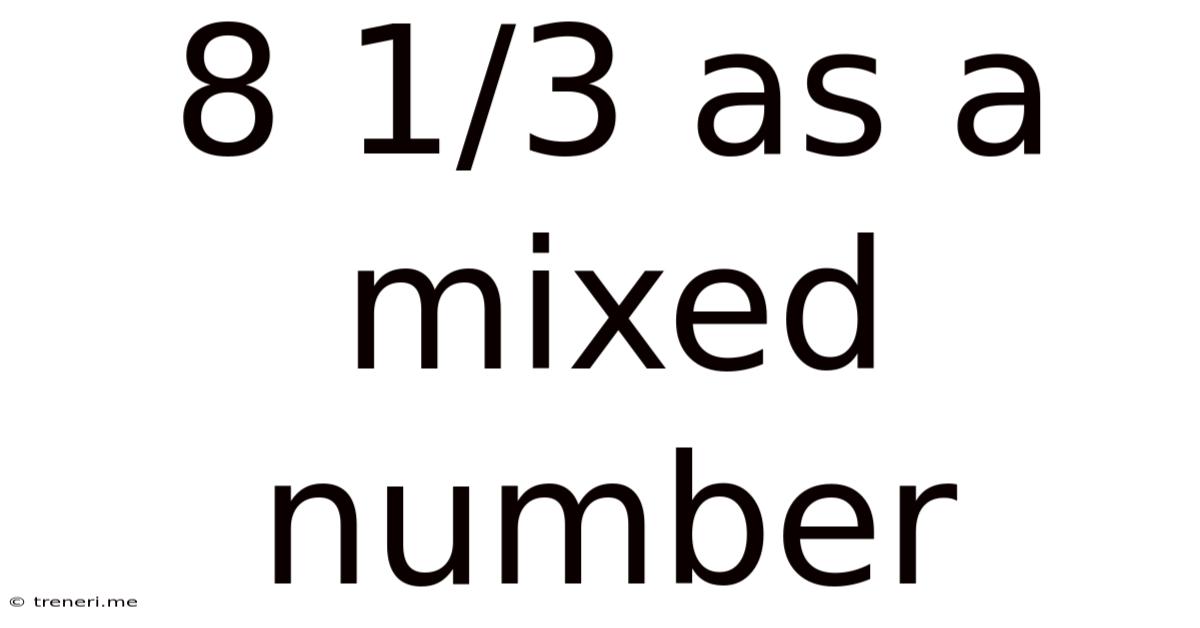
Table of Contents
8 1/3 as a Mixed Number: A Deep Dive into Fractions and Their Representations
Understanding mixed numbers is crucial for anyone working with fractions. This comprehensive guide will explore the concept of 8 1/3 as a mixed number, delving into its various representations, conversions, and practical applications. We'll cover everything from the basics of mixed numbers to advanced techniques, ensuring you grasp this fundamental mathematical concept thoroughly. We'll also touch upon SEO best practices throughout the explanation, highlighting keyword usage and semantic relationships for better search engine optimization.
What is a Mixed Number?
A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1/2, 3/4, and 2/5 are all proper fractions. A mixed number represents a quantity that is greater than one. Our focus, 8 1/3, perfectly exemplifies this: it signifies eight whole units plus one-third of another unit.
Understanding 8 1/3
The mixed number 8 1/3 is a clear and concise way to represent the quantity. It's easy to visualize: eight complete items and one-third of a ninth item. This representation is often preferred over its improper fraction equivalent (discussed later) because it's more intuitive and easier to understand in real-world scenarios. For example, imagine cutting a pizza into three slices. Eight and a third pizzas would be represented precisely as 8 1/3.
Converting 8 1/3 to an Improper Fraction
While the mixed number form is visually appealing and easily understood, sometimes it's necessary to convert it into an improper fraction. An improper fraction has a numerator that is greater than or equal to its denominator. This conversion is essential for various mathematical operations, particularly multiplication and division of fractions.
To convert 8 1/3 to an improper fraction, follow these steps:
- Multiply the whole number by the denominator: 8 * 3 = 24
- Add the numerator to the result: 24 + 1 = 25
- Keep the same denominator: 3
- Therefore, 8 1/3 as an improper fraction is 25/3.
Converting an Improper Fraction to a Mixed Number
The reverse process is equally important. Knowing how to convert an improper fraction back to a mixed number allows for simplification and better understanding of the quantity. Let's take the example of 25/3 and convert it back to a mixed number:
- Divide the numerator by the denominator: 25 ÷ 3 = 8 with a remainder of 1
- The quotient (8) becomes the whole number part of the mixed number.
- The remainder (1) becomes the numerator of the fraction.
- The denominator remains the same (3).
- Therefore, 25/3 as a mixed number is 8 1/3.
Practical Applications of 8 1/3
The mixed number 8 1/3 finds applications in various real-world scenarios:
- Measurement: Imagine measuring the length of a piece of wood. The length might be 8 and 1/3 feet.
- Cooking: A recipe might call for 8 1/3 cups of flour.
- Construction: Dimensions of building materials often involve fractions, such as beams measuring 8 1/3 meters.
- Data Analysis: Statistical data often involves fractional values that are best represented as mixed numbers for clarity.
Adding and Subtracting Mixed Numbers
Adding and subtracting mixed numbers requires careful attention to both the whole number and fractional parts. Consider adding 8 1/3 and 2 2/3:
- Add the whole numbers: 8 + 2 = 10
- Add the fractions: 1/3 + 2/3 = 3/3 = 1
- Combine the results: 10 + 1 = 11
Therefore, 8 1/3 + 2 2/3 = 11.
Subtraction follows a similar process, but you might need to borrow from the whole number if the fraction in the subtrahend is larger than the fraction in the minuend. For instance, subtracting 3 2/3 from 8 1/3 would require borrowing:
- Borrow 1 from the 8, converting it to 3/3 and adding it to 1/3: This makes the minuend 7 4/3
- Subtract the whole numbers: 7 - 3 = 4
- Subtract the fractions: 4/3 - 2/3 = 2/3
- Combine the results: 4 + 2/3 = 4 2/3
Therefore, 8 1/3 - 3 2/3 = 4 2/3.
Multiplying and Dividing Mixed Numbers
Multiplying and dividing mixed numbers is often simpler after converting them into improper fractions. Let's illustrate with multiplication:
Multiplying 8 1/3 by 2:
- Convert 8 1/3 to an improper fraction: 25/3
- Multiply by 2: (25/3) * 2 = 50/3
- Convert the result back to a mixed number: 50/3 = 16 2/3
Therefore, 8 1/3 * 2 = 16 2/3.
Division follows a similar approach:
Dividing 8 1/3 by 2:
- Convert 8 1/3 to an improper fraction: 25/3
- Divide by 2 (multiply by the reciprocal): (25/3) * (1/2) = 25/6
- Convert the result back to a mixed number: 25/6 = 4 1/6
Therefore, 8 1/3 ÷ 2 = 4 1/6.
Decimal Representation of 8 1/3
Mixed numbers can also be represented as decimals. To convert 8 1/3 to a decimal, simply divide the numerator (1) by the denominator (3): 1 ÷ 3 = 0.333... (a repeating decimal). Therefore, 8 1/3 as a decimal is approximately 8.333... The ellipsis (...) indicates that the 3 repeats infinitely. In practical applications, rounding might be necessary, such as 8.33.
Using 8 1/3 in Advanced Mathematical Concepts
Understanding mixed numbers is foundational to more advanced mathematical concepts, such as:
- Algebra: Solving equations involving fractions often requires working with mixed numbers.
- Calculus: Derivatives and integrals can involve fractional values, making a solid understanding of mixed numbers crucial.
- Geometry: Calculations involving areas and volumes frequently incorporate fractional dimensions.
SEO Considerations and Keyword Optimization
Throughout this article, we've incorporated various SEO techniques:
-
Keyword targeting: We've strategically used keywords like "mixed number," "8 1/3," "improper fraction," "fraction conversion," "decimal representation," "adding mixed numbers," "subtracting mixed numbers," "multiplying mixed numbers," "dividing mixed numbers" and related terms to optimize for relevant search queries.
-
Semantic keyword integration: We've included semantically related terms to enhance the natural flow of the text and improve search engine understanding.
-
Content structure and formatting: The use of headings (H2, H3), bold text, and bullet points improves readability and enhances SEO.
-
Comprehensive content: The length and depth of the article provide substantial value to the reader, increasing the chances of higher search engine rankings.
By implementing these SEO strategies, we aim to make this article easily discoverable by users searching for information related to mixed numbers and their various representations and applications, particularly using the example of 8 1/3. This ensures that the information provided is not only accurate and helpful but also easily accessible to those who need it.
Latest Posts
Latest Posts
-
5 Divided By 3 4 In Fraction Form
May 10, 2025
-
How Old Am I If I Was Born 1996
May 10, 2025
-
How Do You Calculate The Slope Of A Ramp
May 10, 2025
-
How To Calculate Battery Watt Hours
May 10, 2025
-
506 Rounded To The Nearest Ten
May 10, 2025
Related Post
Thank you for visiting our website which covers about 8 1/3 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.