99 Divided By 12 With Remainder
Treneri
May 15, 2025 · 4 min read
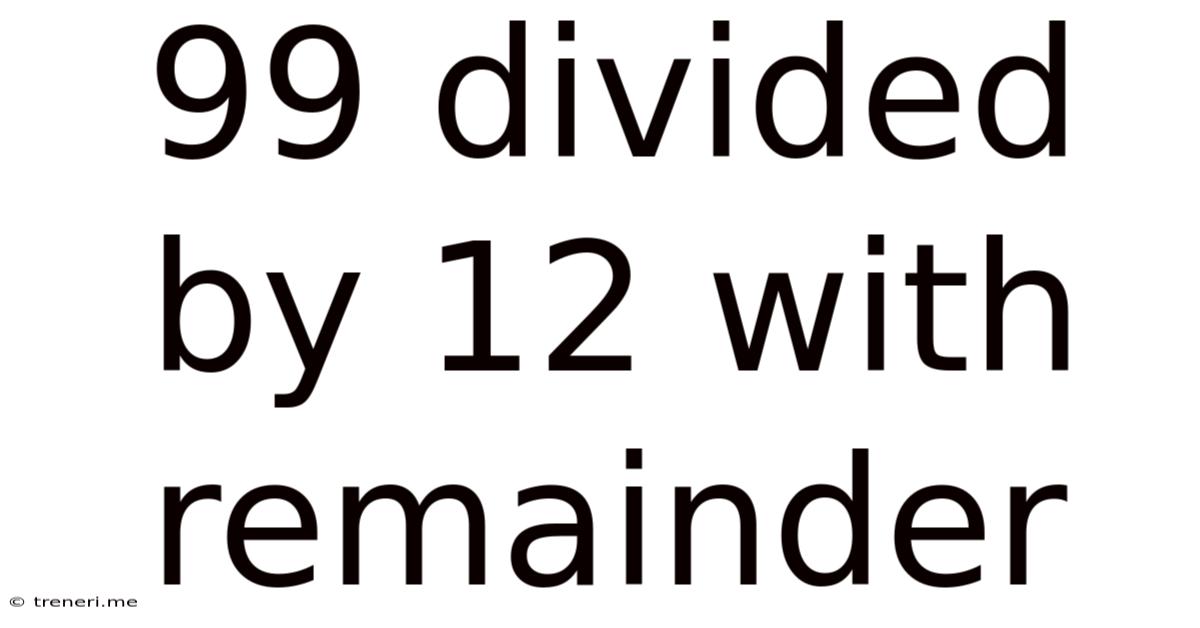
Table of Contents
99 Divided by 12: A Deep Dive into Division with Remainders
The seemingly simple question, "What is 99 divided by 12?", opens a door to a fascinating exploration of division, remainders, and their applications in various fields. While a quick calculation might provide the answer, understanding the underlying principles and the significance of the remainder offers valuable insights into mathematics and its practical uses. This article will delve into this seemingly simple problem, exploring different methods of solving it and highlighting the importance of remainders in real-world scenarios.
Understanding Division and Remainders
Division is a fundamental arithmetic operation that involves splitting a quantity into equal parts. When we divide 99 by 12, we're essentially asking how many times 12 fits completely into 99. However, 12 doesn't divide evenly into 99; there will be a leftover amount, which we call the remainder. This can be represented mathematically as:
Dividend = (Divisor × Quotient) + Remainder
In our case:
- Dividend: 99 (the number being divided)
- Divisor: 12 (the number we're dividing by)
- Quotient: The result of the division (how many times 12 goes into 99)
- Remainder: The amount left over after dividing as evenly as possible.
Methods for Calculating 99 Divided by 12
There are several ways to calculate 99 divided by 12 and find the remainder:
1. Long Division
Long division is a classic method that systematically works through the division process.
8
12 | 99
-96
3
Following the long division steps, we find that 12 goes into 99 eight times (the quotient). Subtracting 12 multiplied by 8 (96) from 99 leaves a remainder of 3.
2. Repeated Subtraction
Repeated subtraction is a more intuitive method, especially for visualizing the division process. We repeatedly subtract the divisor (12) from the dividend (99) until we reach a number smaller than the divisor.
99 - 12 = 87 87 - 12 = 75 75 - 12 = 63 63 - 12 = 51 51 - 12 = 39 39 - 12 = 27 27 - 12 = 15 15 - 12 = 3
We subtracted 12 eight times before reaching a remainder of 3.
3. Using a Calculator
Modern calculators provide a quick and efficient way to perform division. While a calculator might directly give the decimal result (8.25), we can easily obtain the remainder by performing the following calculation:
(99 / 12) = 8.25
Then:
99 - (8 * 12) = 3
This confirms our previous results.
The Significance of the Remainder
The remainder (3, in this case) is not just a leftover; it holds significant mathematical and practical meaning.
1. Modular Arithmetic
Remainders are crucial in modular arithmetic, a branch of number theory with wide applications in cryptography, computer science, and other areas. Modular arithmetic deals with the remainders after division by a specific number (the modulus). In our example, 99 modulo 12 (written as 99 mod 12) is 3.
2. Real-World Applications
Imagine you have 99 apples, and you want to divide them equally among 12 friends. Each friend would receive 8 apples, and you'd have 3 apples remaining. The remainder represents the leftover apples.
Other examples include:
- Scheduling: Determining the day of the week after a certain number of days.
- Cycling: Identifying patterns that repeat after a certain number of iterations.
- Data Structures: Hashing algorithms often use remainders to distribute data evenly.
- Inventory Management: Tracking inventory levels and predicting restocking needs.
Expanding on Division with Remainders
Let's explore further concepts related to division with remainders:
1. Different Divisors
Let's consider dividing 99 by other divisors:
- 99 divided by 5: Quotient = 19, Remainder = 4
- 99 divided by 7: Quotient = 14, Remainder = 1
- 99 divided by 11: Quotient = 9, Remainder = 0 (This is an example of even division, where the remainder is 0)
2. Larger Numbers
The principles of division with remainders remain consistent even with significantly larger numbers. For instance, dividing 12345 by 25 will yield a quotient and a remainder, calculated using the same methods outlined above.
3. Negative Numbers
Division involving negative numbers follows similar rules. The sign of the remainder depends on the signs of the dividend and divisor. This requires careful attention to the rules of signed number arithmetic.
Conclusion: The Importance of Understanding Remainders
The seemingly simple problem of 99 divided by 12 reveals a deeper mathematical concept that extends far beyond basic arithmetic. Understanding division with remainders is crucial not only for mastering mathematical principles but also for practical applications in various fields. From modular arithmetic to real-world scenarios involving distribution, scheduling, and data management, the remainder plays a significant role. This article has explored multiple methods for calculating the remainder, discussed its significance, and highlighted its practical implications. By grasping these concepts, you gain a more profound understanding of mathematics and its applicability in everyday life. Mastering division with remainders empowers you to solve a wider range of problems and appreciate the elegance and practicality of this fundamental arithmetic operation.
Latest Posts
Latest Posts
-
One Million Is Equal To How Many Thousands
May 15, 2025
-
What Is The Reciprocal Of 17
May 15, 2025
-
The Two Triangles Below Are Similar Find The Missing Lengths
May 15, 2025
-
60 Days From June 23 2024
May 15, 2025
-
How Many Days Do I Work In A Year
May 15, 2025
Related Post
Thank you for visiting our website which covers about 99 Divided By 12 With Remainder . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.