Area Of A Circle With A Radius Of 4
Treneri
Apr 06, 2025 · 5 min read
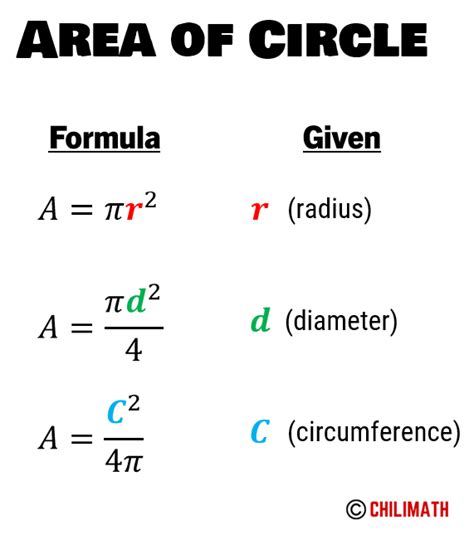
Table of Contents
Calculating the Area of a Circle with a Radius of 4: A Comprehensive Guide
The seemingly simple question of finding the area of a circle with a radius of 4 units opens the door to a fascinating exploration of geometry, mathematical formulas, and their practical applications. This in-depth guide will not only provide the answer but will delve into the underlying concepts, explore variations of the problem, and offer practical examples to solidify your understanding.
Understanding the Formula: Pi, Radius, and Area
The area of any circle is calculated using a fundamental formula deeply rooted in mathematics:
Area = πr²
Where:
- A represents the area of the circle.
- π (pi) is a mathematical constant, approximately equal to 3.14159. It represents the ratio of a circle's circumference to its diameter. Pi is an irrational number, meaning its decimal representation goes on forever without repeating.
- r represents the radius of the circle, which is the distance from the center of the circle to any point on its edge.
In our case, the radius (r) is given as 4 units. This could be 4 centimeters, 4 meters, 4 inches – the unit of measurement will determine the unit of the final area.
Calculating the Area: Step-by-Step Solution
Let's plug the given radius into the formula:
Area = π * (4)²
-
Square the radius: 4² = 16
-
Multiply by pi: 16 * π ≈ 16 * 3.14159 ≈ 50.26544
Therefore, the area of a circle with a radius of 4 units is approximately 50.27 square units.
Beyond the Basics: Exploring Variations and Related Concepts
While the calculation itself is straightforward, let's explore some related concepts and variations that deepen our understanding.
1. Different Values of Pi: Precision and Accuracy
The value of pi we used (3.14159) is an approximation. The true value of pi is an infinitely long decimal. The number of decimal places you use will influence the precision of your answer. For most practical purposes, 3.14159 provides sufficient accuracy. However, for highly precise calculations, you might use a more extensive approximation of pi, which can be easily accessed through scientific calculators or programming languages.
2. Working with Different Units: Maintaining Consistency
Remember to maintain consistency in units throughout your calculations. If your radius is given in centimeters, your area will be in square centimeters. Converting units before or after calculation is crucial for accurate results. For example, if the radius is 4 inches, the area is approximately 50.27 square inches. If the radius were 4 meters, the area would be 50.27 square meters, and so on. This consistency is vital in various applications, from engineering and construction to astronomy and physics.
3. Diameter and Area: An Interrelated Relationship
The diameter of a circle (d) is twice its radius (r): d = 2r. We can rewrite the area formula in terms of the diameter:
Area = π(d/2)² = πd²/4
This alternative formula is useful when the diameter is provided instead of the radius.
4. Circular Sectors and Segments: Subdividing the Circle
The formula for the area of a circle can be extended to calculate the area of sectors and segments of a circle. A sector is a region bounded by two radii and an arc. A segment is a region bounded by a chord and an arc. Calculating these areas requires additional information, such as the angle of the sector or the length of the chord. These calculations often involve trigonometry and advanced geometric concepts.
5. Applications in Real Life: From Engineering to Everyday Life
The concept of calculating the area of a circle is fundamental to numerous real-world applications. Here are a few examples:
- Engineering and Construction: Determining the amount of material needed for circular structures, such as pipes, tanks, or foundations.
- Agriculture: Calculating the area of irrigated fields or the coverage area of a sprinkler system.
- Manufacturing: Calculating the area of circular components in machinery or products.
- Urban Planning: Determining the area of parks, roundabouts, or other circular spaces.
- Science: Calculating cross-sectional areas in fluid dynamics or the area of circular orbits in astronomy.
Advanced Concepts: Exploring Related Mathematical Ideas
Beyond the basic calculations, the area of a circle connects to several more advanced mathematical concepts.
1. Calculus and Integration: Defining Area Through Limits
Calculus provides a more sophisticated way to define the area of a circle. The area can be derived using integration techniques, which involve summing up infinitesimal areas to obtain the total area. This method offers a deeper understanding of how the area formula is fundamentally derived.
2. Geometric Transformations: Scaling and Area Changes
If we were to double the radius of the circle (from 4 to 8), the area would not simply double. Instead, it would increase by a factor of four. This is because the area is proportional to the square of the radius. This concept is important in understanding geometric transformations and how scaling affects area.
3. Coordinate Geometry: Representing Circles in a Cartesian Plane
In coordinate geometry, a circle can be represented by an equation of the form (x-a)² + (y-b)² = r², where (a, b) is the center of the circle and r is the radius. This equation allows us to analyze the circle using algebraic methods.
Conclusion: Mastering the Area of a Circle
The area of a circle with a radius of 4 units, while seemingly simple at first glance, opens doors to a rich exploration of mathematical principles and their practical applications. Understanding the formula, its variations, and its connections to broader mathematical concepts is crucial for various fields. Whether you're an aspiring mathematician, engineer, or simply curious about the world around you, grasping the concept of circular area is a valuable asset. This comprehensive guide has provided a solid foundation, empowering you to confidently tackle similar problems and appreciate the beauty and power of mathematical formulas. Remember, continuous practice and exploration will further solidify your understanding and ability to apply these concepts in diverse contexts.
Latest Posts
Latest Posts
-
How Many Days Till September 9th
Apr 09, 2025
-
Cuanto Es 100 Libras En Kilogramos
Apr 09, 2025
-
Cuanto Es 500 Gramos En Onzas
Apr 09, 2025
-
How Many Days Is 51 Hours
Apr 09, 2025
-
How Many Mg Is 7 5 Ml
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Area Of A Circle With A Radius Of 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.