Common Denominator Of 7 And 6
Treneri
Apr 07, 2025 · 5 min read
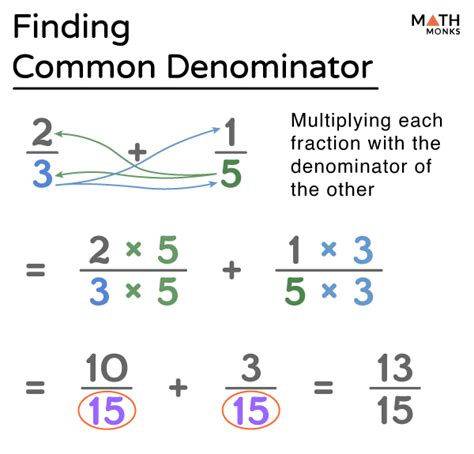
Table of Contents
Finding the Common Denominator of 7 and 6: A Deep Dive into Fractions
Finding the common denominator of two numbers, like 7 and 6, is a fundamental concept in mathematics, crucial for adding, subtracting, and comparing fractions. While seemingly simple at first glance, understanding the underlying principles and exploring different methods can significantly enhance your mathematical proficiency and problem-solving skills. This article will delve deep into the concept, providing various approaches to determine the common denominator of 7 and 6, and expanding on the broader implications of this mathematical operation.
Understanding Fractions and Common Denominators
Before we tackle the specific case of 7 and 6, let's establish a firm grasp of the fundamentals. A fraction represents a part of a whole. It consists of two parts: the numerator (the top number), which indicates the number of parts you have, and the denominator (the bottom number), which indicates the total number of equal parts the whole is divided into.
For example, in the fraction 3/4, the numerator is 3, representing 3 parts, and the denominator is 4, indicating that the whole is divided into 4 equal parts.
When adding or subtracting fractions, they must have the same denominator. This common denominator allows us to directly compare and combine the parts. If the fractions have different denominators, we need to find a common denominator before proceeding with the arithmetic operation.
Methods for Finding the Common Denominator of 7 and 6
Several methods can be used to find the common denominator of 7 and 6. Let's explore the most common and effective approaches:
1. Listing Multiples
This is a straightforward method, especially for smaller numbers. We list the multiples of each number until we find a common multiple.
- Multiples of 7: 7, 14, 21, 28, 35, 42, 49, 56, 63, 70, 77, 84...
- Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48, 54, 60, 66, 72...
Notice that 42 appears in both lists. Therefore, 42 is a common multiple of 7 and 6. In fact, it's the least common multiple (LCM), which is the smallest common multiple. The LCM is the most efficient common denominator to use in calculations.
2. Prime Factorization
This method is more systematic and particularly useful for larger numbers. It involves breaking down each number into its prime factors. Prime factors are numbers divisible only by 1 and themselves (e.g., 2, 3, 5, 7, 11...).
- Prime factorization of 7: 7 (7 is a prime number)
- Prime factorization of 6: 2 x 3
To find the LCM, we take the highest power of each prime factor present in the factorizations:
- We have a 2, a 3, and a 7.
- LCM = 2 x 3 x 7 = 42
Therefore, the LCM of 7 and 6 is 42.
3. Using the Formula: LCM(a, b) = (a x b) / GCD(a, b)
This method utilizes the greatest common divisor (GCD) of the two numbers. The GCD is the largest number that divides both numbers without leaving a remainder.
-
Finding the GCD of 7 and 6: The GCD of 7 and 6 is 1, as they share no common factors other than 1.
-
Applying the formula: LCM(7, 6) = (7 x 6) / GCD(7, 6) = (42) / 1 = 42
This confirms that the LCM, and thus the most efficient common denominator, is 42.
Applying the Common Denominator: Example
Let's illustrate the use of the common denominator with an example. Suppose we need to add the fractions 2/7 and 1/6.
-
Find the common denominator: As we've established, the LCM of 7 and 6 is 42.
-
Convert the fractions:
-
To convert 2/7 to a fraction with a denominator of 42, we multiply both the numerator and denominator by 6: (2 x 6) / (7 x 6) = 12/42
-
To convert 1/6 to a fraction with a denominator of 42, we multiply both the numerator and denominator by 7: (1 x 7) / (6 x 7) = 7/42
-
-
Add the fractions: Now that the fractions have the same denominator, we can add the numerators: 12/42 + 7/42 = 19/42
Therefore, 2/7 + 1/6 = 19/42.
Beyond the Basics: Expanding the Concept
Understanding common denominators extends beyond simple fraction arithmetic. It plays a crucial role in various mathematical areas, including:
1. Algebraic Fractions:
The same principles apply when dealing with algebraic fractions (fractions containing variables). Finding a common denominator is essential for simplifying and manipulating algebraic expressions.
2. Complex Numbers:
Common denominators are also used when performing operations with complex numbers, which involve both real and imaginary parts.
3. Calculus:
In calculus, finding common denominators is crucial for simplifying integrands (expressions being integrated) and for manipulating limits.
Practical Applications in Real Life
While the concept may seem abstract, finding common denominators has many practical applications in everyday life:
-
Baking and Cooking: Recipes often require fractions of ingredients. Understanding common denominators helps in accurately measuring and combining ingredients.
-
Construction and Engineering: Precise measurements are critical in these fields. Common denominators ensure accurate calculations for dimensions and quantities.
-
Finance: Working with percentages and proportions in financial calculations often requires manipulating fractions and finding common denominators for accurate results.
-
Data Analysis: Data analysis frequently involves working with fractions and proportions, making common denominators essential for accurate interpretations.
Conclusion: Mastering the Common Denominator
Finding the common denominator, specifically for numbers like 7 and 6, might appear trivial at first glance. However, a thorough understanding of the underlying principles and the various methods for calculation provides a robust foundation for advanced mathematical concepts and problem-solving. Mastering this fundamental skill empowers you to tackle more complex mathematical challenges confidently and efficiently, making it an invaluable asset in various academic and real-world scenarios. By understanding the methods of listing multiples, prime factorization, and using the GCD, you are equipped to find the common denominator for any pair of numbers, paving the way for a deeper appreciation of fractional arithmetic and its broader applications. Remember that the least common multiple provides the most efficient common denominator for calculations, streamlining the process and minimizing potential errors.
Latest Posts
Latest Posts
-
Perimeter Of Right Angle Triangle Formula
Apr 08, 2025
-
150 G Equals How Many Ounces
Apr 08, 2025
-
Miles A Gallon To Km A Litre
Apr 08, 2025
-
How Much Time Till 8 Am
Apr 08, 2025
-
How To Tell How Old A Hamster Is
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Common Denominator Of 7 And 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.