Common Factors Of 9 And 8
Treneri
May 14, 2025 · 5 min read
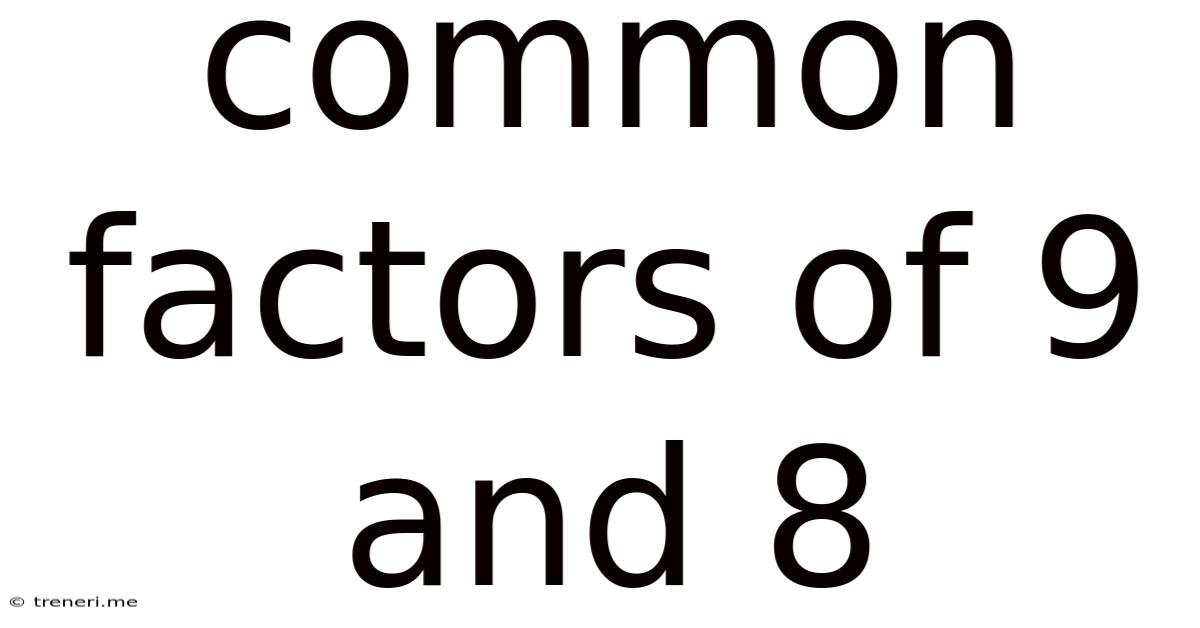
Table of Contents
Unveiling the Secrets: A Deep Dive into the Common Factors of 9 and 8
Finding the common factors of two numbers might seem like a simple mathematical task, but understanding the underlying principles reveals a fascinating glimpse into number theory. This article delves deep into the common factors of 9 and 8, exploring the methods for finding them, their significance in mathematics, and their broader applications. We'll go beyond a simple answer to provide a comprehensive understanding of this concept, suitable for both beginners and those seeking a refresher.
Understanding Factors and Common Factors
Before we tackle the specifics of 9 and 8, let's solidify our understanding of fundamental terms. A factor (or divisor) of a number is a whole number that divides that number evenly without leaving a remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12.
A common factor is a number that is a factor of two or more numbers. For example, the common factors of 12 and 18 are 1, 2, 3, and 6. These numbers divide both 12 and 18 without leaving a remainder.
Finding the Factors of 9
Let's start by identifying all the factors of 9. A systematic approach is crucial here. We can list the pairs of numbers that multiply to 9:
- 1 x 9 = 9
- 3 x 3 = 9
Therefore, the factors of 9 are 1, 3, and 9.
Finding the Factors of 8
Next, let's find all the factors of 8:
- 1 x 8 = 8
- 2 x 4 = 8
Thus, the factors of 8 are 1, 2, 4, and 8.
Identifying the Common Factors of 9 and 8
Now that we have a complete list of factors for both 9 and 8, we can identify the numbers that appear in both lists. Comparing the two sets, we see that only one number is present in both:
- 1
Therefore, the only common factor of 9 and 8 is 1.
Prime Factorization: A Powerful Tool
Prime factorization is a powerful technique for finding common factors. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. Prime factorization involves expressing a number as the product of its prime factors.
Let's apply this to 9 and 8:
- Prime Factorization of 9: 3 x 3 = 3²
- Prime Factorization of 8: 2 x 2 x 2 = 2³
Notice that there are no common prime factors between 9 and 8. This reinforces our earlier finding that their only common factor is 1.
Greatest Common Factor (GCF)
The greatest common factor (GCF), also known as the highest common factor (HCF), is the largest number that divides two or more numbers without leaving a remainder. In the case of 9 and 8, the GCF is 1. Numbers that have a GCF of 1 are called relatively prime or coprime. This means they share no common factors other than 1.
Least Common Multiple (LCM)
While we've focused on common factors, understanding the least common multiple (LCM) provides a complementary perspective. The LCM is the smallest positive number that is a multiple of two or more numbers. Finding the LCM is often useful in solving problems involving fractions or working with rhythmic patterns.
To find the LCM of 9 and 8, we can list the multiples of each number:
- Multiples of 9: 9, 18, 27, 36, 45, 54, 63, 72, ...
- Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, ...
The smallest number that appears in both lists is 72. Therefore, the LCM of 9 and 8 is 72.
The Relationship Between GCF and LCM
There's an interesting relationship between the GCF and LCM of two numbers (a and b):
(a x b) = (GCF(a, b) x LCM(a, b))
Let's verify this for 9 and 8:
(9 x 8) = (1 x 72)
72 = 72
The equation holds true, demonstrating the connection between the GCF and LCM.
Applications of Common Factors
Understanding common factors isn't just an academic exercise; it has practical applications in various fields:
1. Fraction Simplification:
Simplifying fractions involves dividing both the numerator and denominator by their GCF. For example, simplifying the fraction 18/27 requires finding the GCF of 18 and 27, which is 9. Dividing both by 9 results in the simplified fraction 2/3.
2. Geometry and Measurement:
Common factors are crucial in solving problems involving area, volume, and other geometric measurements. For instance, when determining the dimensions of tiles needed to cover a floor, the GCF can help find the largest possible square tile size.
3. Scheduling and Time Management:
Common factors are useful in scheduling events that occur at different intervals. Consider two events, one occurring every 9 days and another every 8 days. Finding the LCM helps determine when both events will coincide.
4. Cryptography:
Number theory, including the concept of factors and prime numbers, plays a critical role in modern cryptography, forming the basis for secure communication and data protection.
5. Modular Arithmetic:
Common factors are integral to modular arithmetic, a branch of number theory with applications in computer science and cryptography. Modular arithmetic focuses on the remainders when integers are divided by a fixed positive integer (the modulus).
Beyond the Basics: Exploring Further Concepts
The exploration of common factors can lead to further mathematical concepts, including:
- Euclidean Algorithm: An efficient method for finding the GCF of two numbers.
- Diophantine Equations: Equations involving integers, often requiring the understanding of GCF and LCM for solutions.
- Number Theory: The branch of mathematics devoted to the properties of integers.
Conclusion: The Significance of Simple Concepts
While the common factors of 9 and 8 might seem elementary, understanding this simple concept opens doors to a deeper understanding of number theory and its widespread applications. From simplifying fractions to securing online transactions, the principles explored here form the foundation of more complex mathematical ideas. This comprehensive analysis has illuminated not only the single common factor shared by 9 and 8 but also highlighted the broader significance of common factors in various mathematical contexts. Mastering these foundational concepts empowers you to approach more advanced mathematical challenges with confidence and skill.
Latest Posts
Latest Posts
-
90 Days From Dec 2 2024
May 14, 2025
-
What Is An Equivalent Fraction To 5 6
May 14, 2025
-
What Is The Greatest Common Factor Of 32 And 56
May 14, 2025
-
Cuanto Es 9 Libras En Kilogramos
May 14, 2025
-
How Many Pounds Is 5 Gallons Of Paint
May 14, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 9 And 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.