Fractions That Are Equivalent To 8/12
Treneri
May 09, 2025 · 5 min read
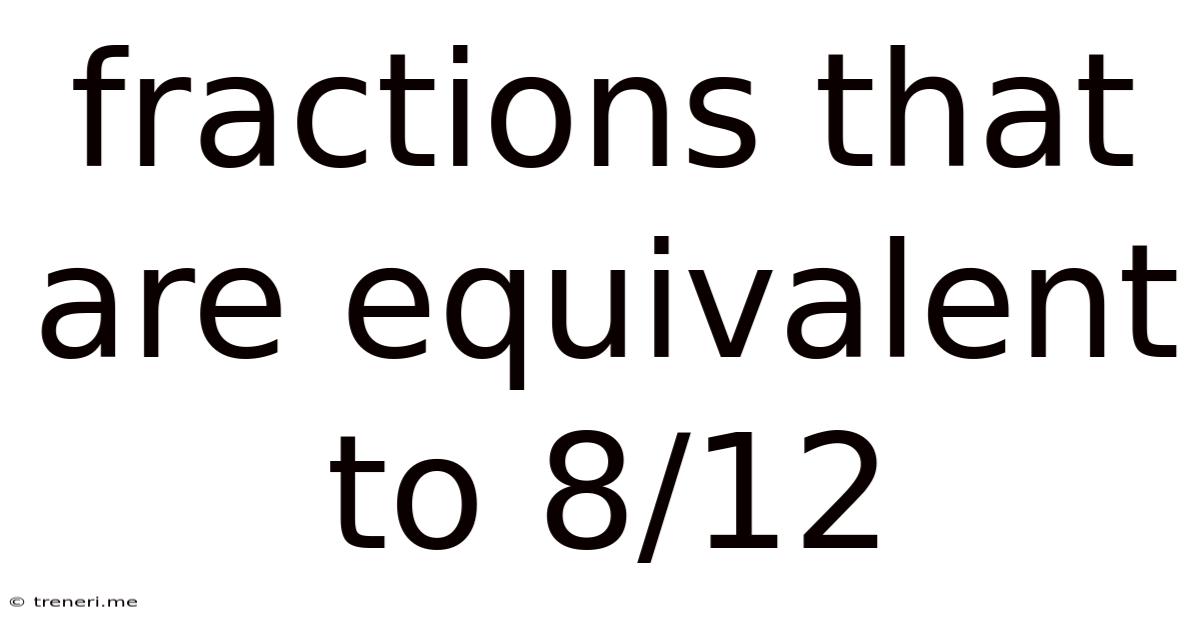
Table of Contents
Fractions Equivalent to 8/12: A Comprehensive Guide
Understanding equivalent fractions is a fundamental concept in mathematics, crucial for various applications from basic arithmetic to advanced calculus. This article delves deep into the world of fractions equivalent to 8/12, exploring different methods to find them, their practical applications, and how to simplify them to their simplest form. We'll also touch upon the importance of understanding equivalent fractions in real-world scenarios.
What are Equivalent Fractions?
Equivalent fractions represent the same proportion or value, even though they appear different. Think of it like slicing a pizza: one-half (1/2) of a pizza is the same amount as two-quarters (2/4) or four-eighths (4/8). These are all equivalent fractions. The key is that the ratio between the numerator (top number) and the denominator (bottom number) remains constant.
Finding Fractions Equivalent to 8/12
There are several ways to find fractions equivalent to 8/12. Let's explore the most common methods:
1. Multiplying the Numerator and Denominator by the Same Number
The simplest method is to multiply both the numerator and the denominator by the same whole number (other than zero). This doesn't change the fraction's value because you're essentially multiplying by 1 (any number divided by itself equals 1).
For example, let's find some equivalent fractions to 8/12:
- Multiply by 2: (8 x 2) / (12 x 2) = 16/24
- Multiply by 3: (8 x 3) / (12 x 3) = 24/36
- Multiply by 4: (8 x 4) / (12 x 4) = 32/48
- Multiply by 5: (8 x 5) / (12 x 5) = 40/60
And so on. You can continue this process indefinitely, generating an infinite number of equivalent fractions.
2. Dividing the Numerator and Denominator by the Same Number
This method is the opposite of the previous one. You divide both the numerator and denominator by the same whole number, provided it divides both evenly. This process is called simplification or reducing a fraction to its lowest terms.
Let's simplify 8/12:
Both 8 and 12 are divisible by 2, 4, and their greatest common divisor (GCD) which is 4.
- Divide by 2: (8 ÷ 2) / (12 ÷ 2) = 4/6
- Divide by 4: (8 ÷ 4) / (12 ÷ 4) = 2/3
2/3 is the simplest form of 8/12. It's important to note that while 4/6 is an equivalent fraction to 8/12, it's not the simplest equivalent fraction.
Understanding the Greatest Common Divisor (GCD)
The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder. Finding the GCD is crucial for simplifying fractions to their lowest terms efficiently. There are several methods to find the GCD, including:
- Listing Factors: List all the factors of both numbers and find the largest one they share.
- Prime Factorization: Break down both numbers into their prime factors and multiply the common factors.
- Euclidean Algorithm: A more efficient method for larger numbers. This involves repeatedly applying division with remainder until the remainder is 0. The last non-zero remainder is the GCD.
For 8 and 12, the GCD is 4. Dividing both the numerator and denominator of 8/12 by 4 gives us the simplest form: 2/3.
Simplifying Fractions: Why It Matters
Simplifying fractions is important for several reasons:
- Clarity: Simpler fractions are easier to understand and visualize.
- Efficiency: Simpler fractions make calculations easier and faster.
- Standardization: Expressing fractions in their simplest form ensures consistency and avoids confusion.
Real-World Applications of Equivalent Fractions
Equivalent fractions are not just abstract mathematical concepts; they have widespread applications in everyday life:
-
Cooking and Baking: Recipes often require fractions of ingredients. Understanding equivalent fractions allows you to adjust recipes based on the available quantities. For instance, if a recipe calls for 1/2 cup of sugar and you only have 1/4 cup measures, you know you need two 1/4 cups.
-
Measurement and Construction: Construction and engineering rely heavily on precise measurements, often involving fractions. Equivalent fractions help in converting between different units of measurement.
-
Sharing and Division: Fairly dividing resources among multiple people often involves fractions. Understanding equivalent fractions ensures everyone receives an equal share.
-
Data Analysis and Statistics: Representing data using fractions and percentages involves the concept of equivalent fractions. Converting between fractions, decimals, and percentages relies on this understanding.
-
Finance: Understanding fractions is essential for working with percentages, interest rates, and proportions in financial calculations.
Beyond 8/12: Exploring Other Equivalent Fractions
While we've focused on 8/12, the principles discussed apply to any fraction. To find equivalent fractions for any given fraction:
-
Identify the simplest form: Find the greatest common divisor (GCD) of the numerator and denominator and divide both by the GCD.
-
Generate equivalent fractions: Multiply both the numerator and denominator of the simplest form by any whole number (other than zero) to generate an infinite number of equivalent fractions.
Practice Problems
Let's test your understanding with some practice problems:
- Find three fractions equivalent to 6/9.
- Simplify the fraction 15/25 to its simplest form.
- Are 12/18 and 2/3 equivalent fractions? Explain your answer.
- A recipe calls for 2/3 cup of flour. You only have a 1/6 cup measuring cup. How many 1/6 cups of flour do you need?
- If you have 16/24 of a pizza, what fraction of the pizza represents the simplest form?
Solutions:
- 2/3, 12/18, 18/27
- 3/5
- Yes, both simplify to 2/3.
- You need 4 (1/6) cups of flour.
- 2/3
Conclusion
Understanding equivalent fractions is a crucial skill in mathematics with numerous real-world applications. Mastering the techniques to find, simplify, and utilize equivalent fractions empowers you to tackle various mathematical problems and challenges with confidence. Remember the importance of simplifying fractions to their simplest form for clarity, efficiency, and consistency. By consistently practicing these concepts, you'll build a strong foundation in fractions and their broader applications in diverse fields.
Latest Posts
Latest Posts
-
How Many Ms In An Hour
May 10, 2025
-
How Many Megabytes Are In 16 Gigabytes
May 10, 2025
-
How Many Days Since Sept 1
May 10, 2025
-
How Many Days Since Jan 29
May 10, 2025
-
6 Out Of 21 As A Percentage
May 10, 2025
Related Post
Thank you for visiting our website which covers about Fractions That Are Equivalent To 8/12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.