How To Find Length And Width From Perimeter
Treneri
Apr 05, 2025 · 5 min read
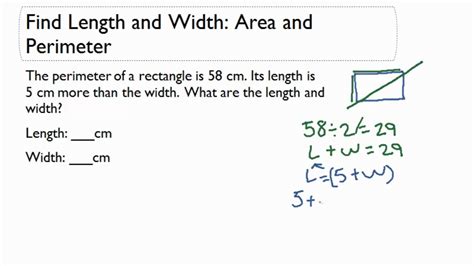
Table of Contents
How to Find Length and Width from Perimeter: A Comprehensive Guide
Knowing how to determine the length and width of a rectangle given its perimeter is a fundamental skill in geometry and has practical applications in various fields, from construction and design to everyday problem-solving. This comprehensive guide will walk you through different methods, scenarios, and considerations to master this essential calculation.
Understanding Perimeter
Before diving into calculations, let's establish a clear understanding of what perimeter means. The perimeter of a two-dimensional shape is the total distance around its outer edge. For a rectangle, this is calculated by adding the lengths of all four sides. Since a rectangle has two pairs of equal sides (opposite sides are equal), the formula for the perimeter (P) is:
P = 2(length + width) or P = 2l + 2w
Where:
- P represents the perimeter
- l represents the length
- w represents the width
Scenario 1: One Dimension is Known
The simplest scenario involves knowing the perimeter and one of the dimensions (either length or width). Let's say we know the perimeter and the length. We can rearrange the perimeter formula to solve for the unknown width:
P = 2l + 2w
Subtract 2l from both sides:
P - 2l = 2w
Divide both sides by 2:
w = (P - 2l) / 2
Example:
A rectangle has a perimeter of 28 meters and a length of 10 meters. What is its width?
- Substitute the known values into the formula:
w = (28 - 2 * 10) / 2 - Calculate:
w = (28 - 20) / 2 = 8 / 2 = 4meters
Therefore, the width of the rectangle is 4 meters. The same principle applies if you know the perimeter and the width; simply rearrange the formula to solve for the length:
l = (P - 2w) / 2
Scenario 2: Only the Perimeter and a Relationship Between Length and Width are Known
This scenario introduces an additional layer of complexity. You'll be given the perimeter and a relationship between the length and width. This relationship might be expressed in words or as an equation.
Example:
A rectangle has a perimeter of 36 cm. The length is 3 cm more than the width. Find the length and width.
- Define Variables: Let's represent the width as 'w' and the length as 'l'.
- Express the Relationship: We know that
l = w + 3. - Substitute: Substitute this relationship into the perimeter formula:
36 = 2(w + 3) + 2w - Solve for w:
36 = 2w + 6 + 2w36 = 4w + 630 = 4ww = 7.5cm
- Solve for l: Substitute the value of 'w' back into the relationship
l = w + 3:l = 7.5 + 3 = 10.5cm
Therefore, the width is 7.5 cm and the length is 10.5 cm.
Scenario 3: Area and Perimeter are Known
This presents a more challenging problem requiring the solution of a quadratic equation. You'll need to use both the perimeter and area formulas:
- Perimeter:
P = 2l + 2w - Area:
A = lw
Example:
A rectangle has an area of 72 square meters and a perimeter of 34 meters. Find its length and width.
- Express one variable: Solve the perimeter equation for one variable (e.g., 'l'):
l = (34 - 2w) / 2 = 17 - w - Substitute: Substitute this expression for 'l' into the area equation:
72 = (17 - w)w - Solve the quadratic equation:
72 = 17w - w²w² - 17w + 72 = 0- This quadratic equation can be factored:
(w - 8)(w - 9) = 0 - This gives two possible solutions for w:
w = 8orw = 9.
- Find the corresponding length:
- If
w = 8, thenl = 17 - 8 = 9 - If
w = 9, thenl = 17 - 9 = 8
- If
Both solutions are valid because they represent the same rectangle (length and width are interchangeable). Therefore, the dimensions of the rectangle are 8 meters and 9 meters.
Dealing with Different Units
Always ensure that all measurements are in the same unit before performing calculations. If the perimeter is given in meters and the length is given in centimeters, convert one to match the other before applying the formula.
Practical Applications
The ability to calculate length and width from perimeter is invaluable in many practical situations:
- Construction: Determining the dimensions of rooms, walls, or foundations.
- Gardening: Designing garden beds or layouts.
- Interior Design: Planning furniture placement and room dimensions.
- Fabric Cutting: Calculating the required fabric for sewing projects.
- Packaging: Designing boxes or containers of specific dimensions.
Advanced Scenarios and Considerations
While the scenarios above cover the most common cases, more complex problems might involve:
- Irregular Shapes: The principles can be adapted to other shapes with known relationships between sides.
- Three-Dimensional Objects: Calculations extend to finding dimensions of cuboids or other 3D shapes, requiring the use of volume and surface area formulas.
- Real-World Constraints: Practical problems might involve additional constraints, such as limitations on material or budget.
Troubleshooting Common Mistakes
- Incorrect Formula: Double-check that you're using the correct formula for the perimeter of a rectangle.
- Unit Inconsistency: Ensure all measurements are in the same units.
- Algebraic Errors: Carefully check your algebraic steps when solving equations.
- Misinterpreting the Problem: Thoroughly read and understand the problem statement before attempting to solve it.
Conclusion
Finding the length and width of a rectangle from its perimeter involves applying fundamental geometric principles and algebraic techniques. By understanding the various scenarios and mastering the relevant formulas, you’ll equip yourself with a valuable skill applicable to numerous practical situations and further mathematical explorations. Remember to always double-check your work, and don't hesitate to break down complex problems into smaller, manageable steps. This comprehensive guide provides a solid foundation for confidently tackling these types of problems. Practice is key to mastering these calculations; work through various examples to solidify your understanding and build your problem-solving skills.
Latest Posts
Latest Posts
-
Magnitude Of The Force Of Friction
Apr 06, 2025
-
What Is A 12 Out Of 15 Grade
Apr 06, 2025
-
How Many Cubic Inches Is In A Gallon
Apr 06, 2025
-
1 Kg Is How Many Ounces
Apr 06, 2025
-
How Many Hours Are In 12 Years
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about How To Find Length And Width From Perimeter . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
