Is 1 2 Greater Than 1
Treneri
May 11, 2025 · 4 min read
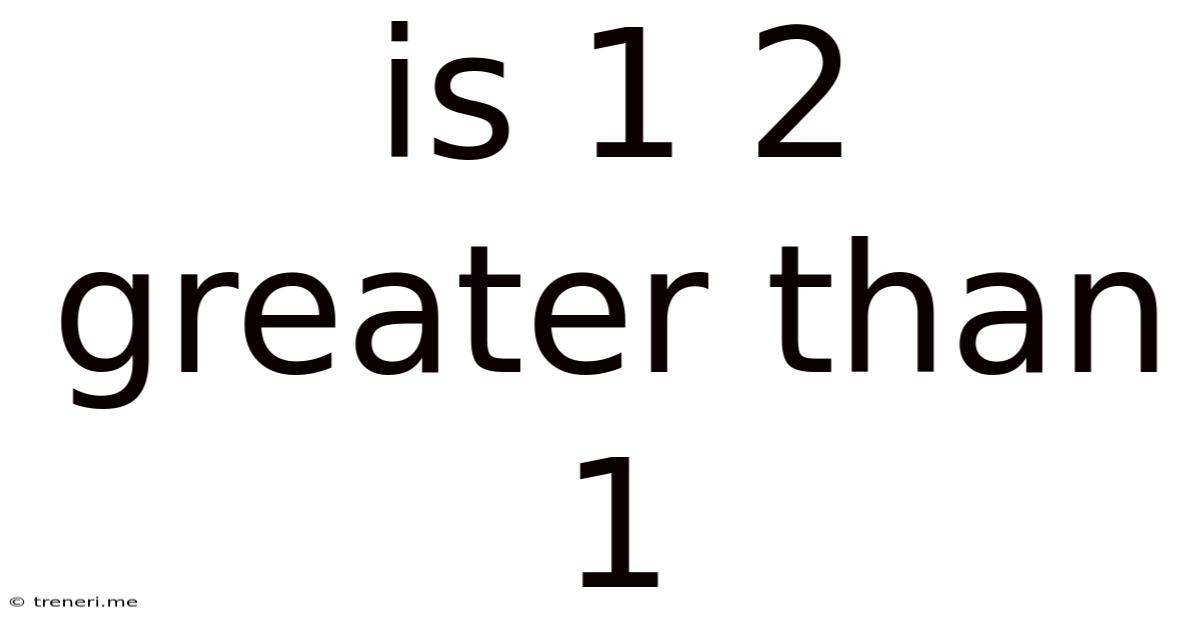
Table of Contents
Is 1.2 Greater Than 1? A Deep Dive into Number Comparison
The seemingly simple question, "Is 1.2 greater than 1?", might appear trivial at first glance. However, exploring this question allows us to delve into fundamental concepts of number systems, numerical representation, and the logic behind comparisons. This article will not only answer the question definitively but will also explore the broader implications and related mathematical concepts.
Understanding Number Systems
Before directly comparing 1.2 and 1, let's establish a firm understanding of the number systems involved. We primarily operate within the real number system, which encompasses various subsets:
1. Natural Numbers (Counting Numbers):
These are the numbers we use for counting: 1, 2, 3, 4, and so on. They are positive integers and form the foundation of many mathematical concepts.
2. Integers:
Integers extend natural numbers to include zero and negative whole numbers: ..., -3, -2, -1, 0, 1, 2, 3, ...
3. Rational Numbers:
These numbers can be expressed as a fraction p/q, where p and q are integers, and q is not zero. Rational numbers include integers (e.g., 2/1 = 2), as well as fractions and terminating or repeating decimals (e.g., 1/2 = 0.5, 1/3 = 0.333...).
4. Irrational Numbers:
These numbers cannot be expressed as a fraction of two integers. They have non-terminating, non-repeating decimal representations. Famous examples include π (pi) and √2 (the square root of 2).
5. Real Numbers:
The real number system encompasses all rational and irrational numbers. It represents all points on the number line.
Decimal Representation and Place Value
The numbers 1.2 and 1 are represented in decimal notation. Understanding place value is crucial for comparison. In the decimal system, each digit's position represents a power of 10.
For 1.2:
- 1: Represents one unit (10⁰).
- 2: Represents two tenths (2 * 10⁻¹).
For 1:
- 1: Represents one unit (10⁰).
Direct Comparison: 1.2 vs. 1
Now, let's directly compare 1.2 and 1. Since 1.2 has a '2' in the tenths place, it signifies an additional two-tenths of a unit compared to 1. Therefore:
Yes, 1.2 is greater than 1.
This can be visually represented on a number line, where 1.2 is positioned to the right of 1, indicating its larger value.
Extending the Concept: Inequality Symbols
We can express the relationship between 1.2 and 1 using inequality symbols:
- 1.2 > 1 (1.2 is greater than 1)
- 1 < 1.2 (1 is less than 1.2)
These symbols are fundamental in mathematics for representing comparisons between numbers.
Practical Applications and Real-World Examples
The concept of comparing decimal numbers is ubiquitous in daily life:
- Finance: Comparing prices, interest rates, and financial metrics. A price of $1.20 is higher than $1.00.
- Measurement: Comparing lengths, weights, volumes, and other physical quantities. A length of 1.2 meters is longer than 1 meter.
- Science: Comparing experimental results, data points, and scientific measurements. A temperature of 1.2 degrees Celsius is higher than 1 degree Celsius.
- Computing: Comparing numerical values in computer programs and algorithms.
Beyond Simple Comparison: Order of Operations and Complex Expressions
While comparing 1.2 and 1 is straightforward, the principle extends to more complex mathematical expressions. Remember the order of operations (PEMDAS/BODMAS) when evaluating expressions involving decimals:
- Parentheses/Brackets
- Exponents/Orders
- Multiplication and Division (from left to right)
- Addition and Subtraction (from left to right)
For example, consider the expression: 2.5 + 1.2 - 1. Following the order of operations, we first add 2.5 and 1.2 (3.7), then subtract 1, resulting in 2.7. Even in complex scenarios, understanding the relative magnitude of decimal numbers remains critical for accurate calculations.
Number Line Visualization and Intuition
Visualizing numbers on a number line provides an intuitive understanding of their relative magnitudes. Plotting 1 and 1.2 clearly demonstrates that 1.2 lies to the right of 1, reinforcing its greater value. This visual approach is particularly helpful for beginners grasping numerical comparisons.
Error Analysis and Significance of Decimal Places
In scientific and engineering applications, the precision of decimal numbers is crucial. The number of decimal places indicates the accuracy of the measurement or calculation. For instance, 1.20 suggests a higher level of accuracy than 1.2. Understanding significant figures and rounding is vital when dealing with decimal numbers in contexts where precision matters.
Conclusion: The Importance of Foundational Concepts
The simple comparison of 1.2 and 1 serves as a gateway to understanding broader mathematical concepts. From number systems and decimal representation to inequality symbols and order of operations, a grasp of these fundamentals lays the groundwork for more advanced mathematical explorations. The seemingly basic question highlights the significance of understanding the foundational building blocks of mathematics, which are essential for problem-solving in various fields. The ability to accurately compare and manipulate decimal numbers is a crucial skill applicable across diverse disciplines.
Latest Posts
Latest Posts
-
180 Days From September 4 2024
May 12, 2025
-
3 4 1 5 As A Fraction
May 12, 2025
-
What Is An Equivalent Fraction For 2 6
May 12, 2025
-
How Many Days Till 1st Of June
May 12, 2025
-
Cuanto Falta Para El 8 De Abril
May 12, 2025
Related Post
Thank you for visiting our website which covers about Is 1 2 Greater Than 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.