Is 3 12 Equal To 1 4
Treneri
Apr 26, 2025 · 4 min read
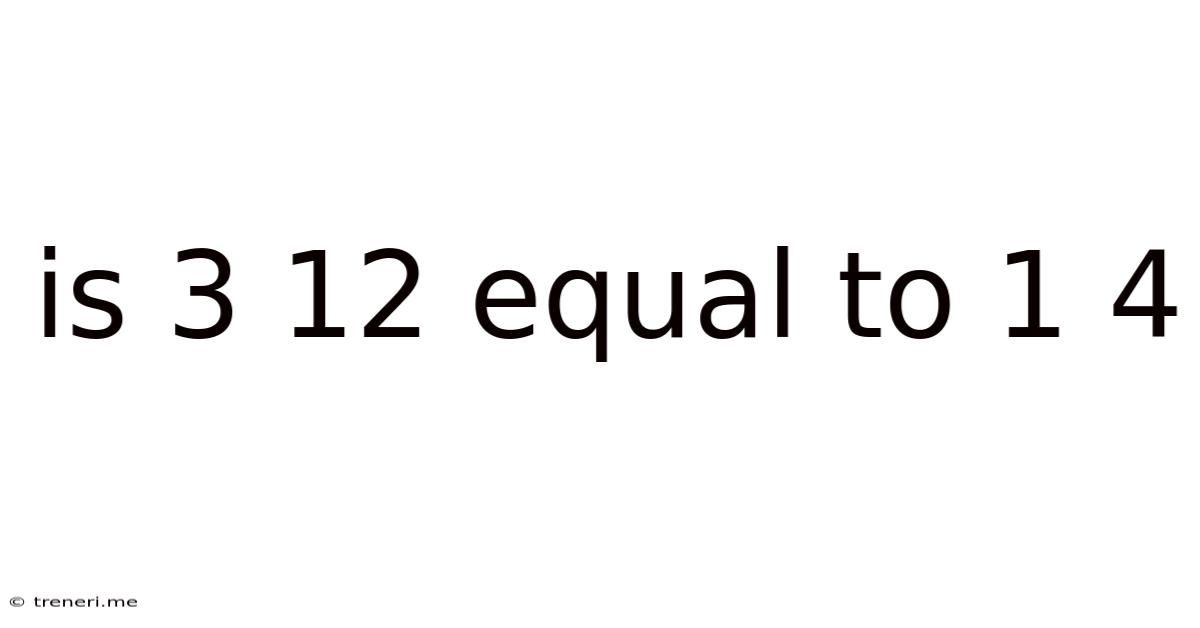
Table of Contents
Is 3/12 Equal to 1/4? A Deep Dive into Fraction Equivalence
The question, "Is 3/12 equal to 1/4?" might seem simple at first glance, particularly for those comfortable with fractions. However, a deeper exploration reveals a wealth of underlying mathematical concepts crucial for understanding fractional equivalence, simplification, and the fundamental building blocks of arithmetic. This article will delve into this seemingly straightforward question, exploring the various methods to determine equivalence, its practical applications, and the broader implications for mathematical understanding.
Understanding Fractions: Numerator and Denominator
Before directly addressing the equivalence of 3/12 and 1/4, let's revisit the core components of a fraction: the numerator and the denominator.
- Numerator: The top number in a fraction represents the number of parts we have. In 3/12, the numerator is 3, indicating that we possess 3 parts.
- Denominator: The bottom number represents the total number of equal parts the whole is divided into. In 3/12, the denominator is 12, meaning the whole has been divided into 12 equal parts.
Therefore, 3/12 signifies that we have 3 out of a possible 12 equal parts.
Method 1: Simplifying Fractions
The most straightforward way to determine if 3/12 is equal to 1/4 is to simplify 3/12. Simplifying a fraction means reducing it to its lowest terms, where the numerator and denominator share no common factors other than 1.
To simplify 3/12, we need to find the greatest common divisor (GCD) of 3 and 12. The GCD is the largest number that divides both 3 and 12 without leaving a remainder. In this case, the GCD is 3.
We divide both the numerator and the denominator by the GCD:
3 ÷ 3 = 1 12 ÷ 3 = 4
This simplifies 3/12 to 1/4. Therefore, yes, 3/12 is equal to 1/4.
Method 2: Finding Equivalent Fractions
Another approach is to find equivalent fractions. Equivalent fractions represent the same portion of a whole, even though they have different numerators and denominators. We can create equivalent fractions by multiplying or dividing both the numerator and the denominator by the same non-zero number.
Let's see if we can transform 1/4 into 3/12:
To get from 4 (the denominator of 1/4) to 12 (the denominator of 3/12), we multiply by 3 (12 ÷ 4 = 3). Since we must perform the same operation on both the numerator and the denominator to maintain equivalence, we multiply the numerator (1) by 3 as well:
1 × 3 = 3 4 × 3 = 12
This results in 3/12. Thus, we've demonstrated that 1/4 and 3/12 are equivalent fractions. Again, this confirms that yes, 3/12 is equal to 1/4.
Visual Representation: Understanding Fractions Geometrically
Visual aids can significantly enhance our understanding of fractional equivalence. Imagine a pizza cut into 12 slices. If you take 3 slices, you've consumed 3/12 of the pizza.
Now, imagine a smaller pizza cut into only 4 slices. If you take one slice, you've consumed 1/4 of the pizza. Visually comparing the amount of pizza consumed in both scenarios reveals that they are identical. This visual representation reinforces the equivalence of 3/12 and 1/4.
Practical Applications: Real-World Examples of Fractional Equivalence
The concept of fractional equivalence isn't confined to theoretical mathematics; it has widespread practical applications in various fields:
- Cooking and Baking: Recipes often require adjustments based on the number of servings. Understanding fractional equivalence allows for accurate scaling of ingredients. If a recipe calls for 1/4 cup of sugar and you want to double the recipe, you'll need 2/4 cup, which simplifies to 1/2 cup.
- Construction and Engineering: Accurate measurements are vital in construction and engineering. Fractional equivalence ensures precision in calculations involving lengths, volumes, and weights.
- Finance: Understanding fractions is critical in finance for calculating interest rates, portions of investments, and profit margins.
- Data Analysis: Fractions are frequently used to represent proportions and percentages in data analysis. Simplifying fractions makes data interpretation clearer and more concise.
Beyond the Basics: Extending the Concept of Fractional Equivalence
The equivalence of 3/12 and 1/4 serves as a fundamental stepping stone to more advanced mathematical concepts:
- Ratio and Proportion: Fractional equivalence is intrinsically linked to ratios and proportions. The ratio 3:12 is equivalent to the ratio 1:4.
- Decimal Representation: Fractions can be converted to decimals, providing another way to compare their values. Both 3/12 and 1/4 are equal to 0.25.
- Percentage Calculation: Fractions can be easily converted into percentages. Both 3/12 and 1/4 represent 25%.
Conclusion: Mastering Fractions – A Foundation for Mathematical Proficiency
The question, "Is 3/12 equal to 1/4?" may appear elementary, but its exploration illuminates the fundamental concepts of fractional equivalence, simplification, and the practical applications of these mathematical principles. Understanding these concepts is crucial not only for academic success but also for navigating everyday situations requiring fractional calculations. Mastering fractions forms a solid foundation for more advanced mathematical studies, enhancing problem-solving abilities and fostering a deeper appreciation for the elegance and practicality of mathematics. By grasping the equivalence of fractions, we unlock a pathway to a more comprehensive understanding of the numerical world around us.
Latest Posts
Latest Posts
-
80 801 Rounded To The Nearest Ten Thousand
May 09, 2025
-
How Many 2x4 To Build A House
May 09, 2025
-
What Is The Quotient A 3 7 3 A 21
May 09, 2025
-
How Many Pounds Is 56 6 Liters
May 09, 2025
-
What Is 17 Degrees In Celsius
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 3 12 Equal To 1 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.