Least Common Denominator Of 8 And 4
Treneri
Apr 06, 2025 · 5 min read
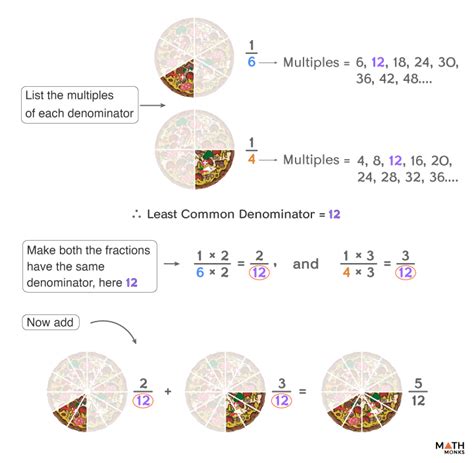
Table of Contents
Finding the Least Common Denominator (LCD) of 8 and 4: A Comprehensive Guide
Finding the least common denominator (LCD) is a fundamental concept in arithmetic, crucial for adding and subtracting fractions. This comprehensive guide will delve into the process of determining the LCD of 8 and 4, explaining various methods and providing a solid understanding of the underlying principles. We'll also explore the broader context of LCDs and their importance in mathematics.
Understanding Least Common Denominator (LCD)
Before we dive into finding the LCD of 8 and 4, let's establish a clear understanding of what an LCD is. The least common denominator is the smallest whole number that is a multiple of all the denominators in a set of fractions. It's the smallest number that all the denominators can divide into evenly. This concept is vital because it allows us to add or subtract fractions with different denominators. Without a common denominator, adding or subtracting fractions directly is impossible.
Think of it like this: you can't directly add apples and oranges without first converting them into a common unit, like pieces of fruit. Similarly, fractions with different denominators require conversion to a common denominator before they can be added or subtracted.
Methods for Finding the LCD of 8 and 4
There are several methods for determining the least common denominator. Let's explore the most common approaches, using the example of 8 and 4:
1. Listing Multiples Method
This is a straightforward method, particularly useful for smaller numbers. We list the multiples of each number until we find the smallest multiple that is common to both.
- Multiples of 4: 4, 8, 12, 16, 20, 24...
- Multiples of 8: 8, 16, 24, 32...
By examining the lists, we can see that the smallest common multiple is 8. Therefore, the LCD of 8 and 4 is 8.
2. Prime Factorization Method
This method is more efficient for larger numbers or when dealing with more than two denominators. It involves breaking down each number into its prime factors.
- Prime factorization of 4: 2 x 2 = 2²
- Prime factorization of 8: 2 x 2 x 2 = 2³
To find the LCD, we take the highest power of each prime factor present in the factorizations:
The only prime factor is 2, and the highest power is 2³ (which is 8). Therefore, the LCD of 8 and 4 is 8.
3. Greatest Common Divisor (GCD) Method
This method utilizes the relationship between the LCD and the greatest common divisor (GCD). The GCD is the largest number that divides both numbers without leaving a remainder. The formula connecting LCD and GCD is:
LCD(a, b) = (a x b) / GCD(a, b)
Let's apply this to 8 and 4:
- GCD of 8 and 4: The largest number that divides both 8 and 4 is 4.
- Applying the formula: (8 x 4) / 4 = 8
Therefore, the LCD of 8 and 4 is 8.
Why the LCD is 8: A Deeper Look
The fact that the LCD of 8 and 4 is 8 is not coincidental. 8 is a multiple of both 4 and 8. This means that 8 can be divided evenly by both 4 and 8 without leaving a remainder. Any other common multiple, such as 16 or 24, would be larger than 8, making 8 the least common multiple.
This concept directly applies to fraction operations. If we have the fractions 1/4 and 3/8, we would need to find a common denominator to add or subtract them. Since the LCD is 8, we can rewrite the fractions as:
- 1/4 = 2/8 (by multiplying both the numerator and denominator by 2)
- 3/8 remains as 3/8
Now we can easily add or subtract the fractions: 2/8 + 3/8 = 5/8.
Applications of LCD in Real-World Scenarios
Understanding and calculating the LCD is not just a theoretical exercise; it has practical applications across numerous fields:
-
Construction and Engineering: Calculating quantities of materials often involves fractions. Finding the LCD ensures accurate measurements and prevents errors.
-
Cooking and Baking: Recipes frequently use fractional measurements. Correctly converting to a common denominator is essential for accurate results.
-
Finance: Working with percentages and proportions in financial calculations requires a solid understanding of fractions and LCDs.
-
Data Analysis: Many data analysis techniques involve working with fractions and proportions. Determining the LCD is crucial for accurate calculations and comparisons.
Advanced Concepts: LCDs with More Than Two Numbers
The methods described above can be extended to find the LCD of more than two numbers. For example, to find the LCD of 4, 6, and 8, we can use the prime factorization method:
- Prime factorization of 4: 2²
- Prime factorization of 6: 2 x 3
- Prime factorization of 8: 2³
The highest power of each prime factor is: 2³ and 3¹. Therefore, the LCD is 2³ x 3 = 24.
Conclusion: Mastering the LCD
The least common denominator is a fundamental concept in mathematics with significant practical applications. Mastering the various methods for calculating the LCD empowers you to tackle fraction operations with confidence and accuracy. Understanding the underlying principles—multiples, prime factorization, and the relationship between LCD and GCD—provides a solid foundation for further mathematical exploration. By using the appropriate method based on the complexity of the numbers involved, you can efficiently and accurately determine the LCD and apply it to various mathematical problems and real-world situations. Remember, practice makes perfect, so continue to work through examples to solidify your understanding and build your skills in finding the LCD.
Latest Posts
Latest Posts
-
How Many Cc Are In 1 Liter
Apr 07, 2025
-
How Many Minutes In 4 Years
Apr 07, 2025
-
How Many Grams In A Fluid Oz
Apr 07, 2025
-
1 4 Ounce Yeast To Teaspoon
Apr 07, 2025
-
How Old Is Someone Born Dec 18 1989
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Least Common Denominator Of 8 And 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
