Standard Form To Point Slope Form
Treneri
Apr 05, 2025 · 5 min read
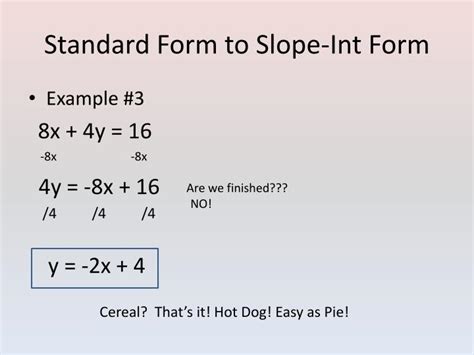
Table of Contents
From Standard Form to Point-Slope Form: A Comprehensive Guide
Converting equations between different forms is a fundamental skill in algebra. Understanding these transformations allows for a deeper comprehension of linear relationships and facilitates problem-solving across various mathematical contexts. This article delves into the process of converting a linear equation from standard form to point-slope form, providing a clear, step-by-step guide with illustrative examples. We'll also explore the advantages of using each form and how this conversion can enhance your understanding of linear functions.
Understanding the Forms
Before diving into the conversion process, let's solidify our understanding of both standard form and point-slope form.
Standard Form: Ax + By = C
The standard form of a linear equation is represented as Ax + By = C, where:
- A, B, and C are integers (whole numbers).
- A is typically a non-negative integer.
- A and B are not both zero.
This form is useful for quickly determining the x- and y-intercepts of a line. The x-intercept is found by setting y = 0 and solving for x, and the y-intercept is found by setting x = 0 and solving for y.
Point-Slope Form: y - y₁ = m(x - x₁)
The point-slope form of a linear equation is represented as y - y₁ = m(x - x₁), where:
- m represents the slope of the line.
- (x₁, y₁) represents a point on the line.
This form is particularly useful when you know the slope of a line and a point that lies on it. It allows for easy plotting and quick visualization of the line.
Converting Standard Form to Point-Slope Form: A Step-by-Step Guide
The conversion from standard form (Ax + By = C) to point-slope form (y - y₁ = m(x - x₁)) involves two key steps: finding the slope (m) and identifying a point (x₁, y₁) on the line.
Step 1: Find the Slope (m)
The slope of a line in standard form (Ax + By = C) can be directly derived by manipulating the equation. Here's how:
-
Isolate the y term: Begin by isolating the term containing 'y' on one side of the equation. This involves subtracting 'Ax' from both sides:
Ax + By = C By = -Ax + C -
Solve for y: Divide both sides by 'B' to solve for 'y':
y = (-A/B)x + (C/B) -
Identify the slope: The coefficient of 'x' in this equation represents the slope (m). Therefore:
m = -A/B
Step 2: Find a Point (x₁, y₁)
Once the slope is determined, we need to find a point that lies on the line. The easiest way to do this is by using the intercepts.
-
Find the x-intercept: Set y = 0 in the standard form equation (Ax + By = C) and solve for x. This gives you the point (x, 0).
-
Find the y-intercept: Set x = 0 in the standard form equation (Ax + By = C) and solve for y. This gives you the point (0, y).
You can choose either intercept (or any other point on the line) to use in the point-slope form.
Step 3: Substitute into Point-Slope Form
Once you have the slope (m) and a point (x₁, y₁), substitute these values into the point-slope form equation: y - y₁ = m(x - x₁).
Illustrative Examples
Let's work through a few examples to solidify the process.
Example 1: Convert 2x + 3y = 6 to point-slope form.
-
Find the slope: A = 2, B = 3. Therefore, m = -A/B = -2/3.
-
Find a point:
- x-intercept: Set y = 0: 2x + 3(0) = 6 => x = 3. Point: (3, 0).
- y-intercept: Set x = 0: 2(0) + 3y = 6 => y = 2. Point: (0, 2).
-
Substitute into point-slope form: Using the point (3, 0): y - 0 = (-2/3)(x - 3) This simplifies to y = (-2/3)x + 2
Using the point (0,2): y - 2 = (-2/3)(x - 0) This simplifies to y = (-2/3)x + 2
Notice that both points yield the same point-slope equation, demonstrating the flexibility of the method.
Example 2: Convert 5x - 2y = 10 to point-slope form.
-
Find the slope: A = 5, B = -2. Therefore, m = -A/B = -5/(-2) = 5/2.
-
Find a point:
- x-intercept: Set y = 0: 5x - 2(0) = 10 => x = 2. Point: (2, 0).
- y-intercept: Set x = 0: 5(0) - 2y = 10 => y = -5. Point: (0, -5).
-
Substitute into point-slope form: Using the point (2, 0): y - 0 = (5/2)(x - 2) This simplifies to y = (5/2)x - 5
Using the point (0,-5): y - (-5) = (5/2)(x - 0) This simplifies to y = (5/2)x - 5
Example 3: Dealing with negative values
Convert -4x + y = -8 to point-slope form.
-
Find the slope: A = -4, B = 1. Therefore, m = -A/B = -(-4)/1 = 4.
-
Find a point:
- x-intercept: Set y = 0: -4x + 0 = -8 => x = 2. Point: (2, 0).
- y-intercept: Set x = 0: -4(0) + y = -8 => y = -8. Point: (0, -8).
-
Substitute into point-slope form: Using the point (2, 0): y - 0 = 4(x - 2) This simplifies to y = 4x - 8
Using the point (0,-8): y - (-8) = 4(x - 0) This simplifies to y = 4x - 8
Advantages of Using Point-Slope Form
The point-slope form offers several advantages:
-
Ease of graphing: It directly provides a point and the slope, making plotting the line on a graph straightforward.
-
Clarity of slope and intercept: The slope is explicitly shown, providing immediate insight into the line's steepness and direction.
-
Efficient calculations: It simplifies calculations when dealing with problems involving slope and a point.
Conclusion
Converting linear equations from standard form to point-slope form is a crucial skill in algebra. Mastering this conversion enhances your understanding of linear relationships, facilitates problem-solving, and empowers you to more easily visualize and manipulate linear functions. The step-by-step guide provided, along with illustrative examples, ensures a clear understanding of the process. Remember to practice regularly to solidify your understanding and build confidence in your algebraic abilities. By consistently applying these techniques, you will be well-equipped to tackle more complex mathematical challenges.
Latest Posts
Latest Posts
-
How Many Pints Are In 7 Cups
Apr 06, 2025
-
97 Months Is How Many Years
Apr 06, 2025
-
How Many Weeks Is 91 Days
Apr 06, 2025
-
Cubic Inches Per Gallon Of Water
Apr 06, 2025
-
15000 Feet Is How Many Miles
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Standard Form To Point Slope Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
