The Quotient Of 9 And A Number
Treneri
May 10, 2025 · 6 min read
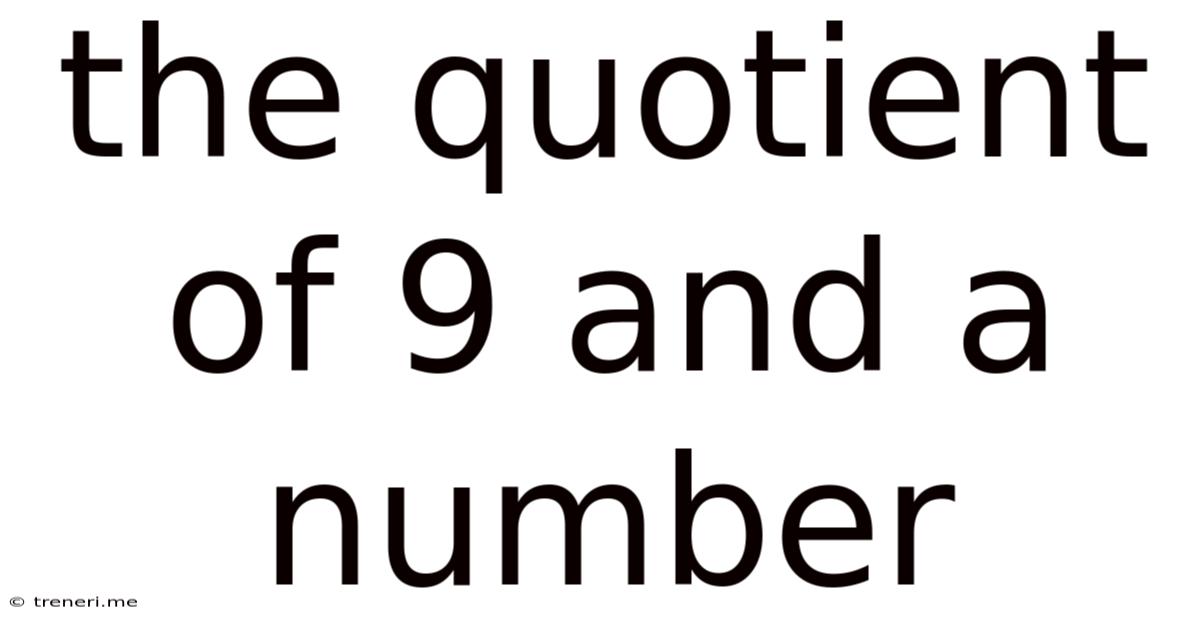
Table of Contents
The Quotient of 9 and a Number: A Deep Dive into Mathematical Concepts and Applications
The seemingly simple phrase "the quotient of 9 and a number" opens a door to a wide array of mathematical concepts, from basic arithmetic to advanced algebraic manipulations. Understanding this fundamental concept is crucial for mastering various mathematical disciplines and their real-world applications. This article will explore the meaning of this phrase, delve into its representation in different mathematical contexts, examine its use in problem-solving, and illustrate its importance in various fields.
Understanding the Quotient
In mathematics, a quotient is the result of dividing one number (the dividend) by another number (the divisor). Therefore, "the quotient of 9 and a number" signifies the result obtained when 9 is divided by an unspecified number. Let's represent this unknown number with the variable 'x'. The expression can then be written as:
9 / x or 9 ÷ x
This simple expression forms the foundation for many more complex mathematical operations and problem-solving scenarios.
Representing the Quotient Algebraically
The algebraic representation, 9/x, allows for a deeper understanding and manipulation of the quotient. This representation enables us to:
1. Solve for x:
If we know the value of the quotient, we can solve for x. For example, if the quotient of 9 and a number is 3, we can set up the equation:
9 / x = 3
To solve for x, we multiply both sides of the equation by x:
9 = 3x
Then, we divide both sides by 3:
x = 3
Therefore, the number is 3. This demonstrates how the algebraic representation facilitates problem-solving.
2. Explore the behavior of the quotient as x varies:
The algebraic representation allows us to analyze how the quotient changes as the value of x changes. For instance:
- If x > 9, the quotient will be less than 1.
- If x = 9, the quotient will be equal to 1.
- If 0 < x < 9, the quotient will be greater than 1.
- If x = 0, the quotient is undefined (division by zero is not allowed).
- If x < 0, the quotient will be negative.
This analysis reveals the inverse relationship between x and the quotient: as x increases, the quotient decreases, and vice versa. This understanding is vital in various applications, including physics and engineering.
3. Use in more complex equations:
The expression 9/x can be a component of more complex algebraic equations. For example, consider the equation:
(9/x) + 5 = 10
To solve this equation, we first isolate the term with x:
9/x = 5
Then, we solve for x as shown in the previous example, resulting in x = 9/5 or 1.8. This shows how understanding the quotient of 9 and a number is a building block for solving more intricate mathematical problems.
Applications of the Quotient of 9 and a Number
The concept of "the quotient of 9 and a number" isn't just a theoretical exercise; it has practical applications across several fields:
1. Rate and Ratio Problems:
Many real-world problems involve calculating rates or ratios. For example:
- Speed: If a car travels 9 miles in 'x' hours, the average speed is 9/x miles per hour.
- Unit Cost: If 9 items cost 'x' dollars, the cost per item is 9/x dollars.
- Density: If 9 grams of a substance occupy 'x' cubic centimeters, the density is 9/x grams per cubic centimeter.
These examples highlight the ubiquitous nature of the quotient in calculating rates and ratios which are essential concepts in various practical scenarios.
2. Financial Calculations:
Financial calculations often involve quotients. For example:
- Return on Investment (ROI): If you invest 9 dollars and receive a profit of 'x' dollars, the ROI is (x/9) * 100%.
- Earnings Per Share (EPS): If a company earns 9 million dollars and has 'x' million shares outstanding, the EPS is 9/x dollars per share.
- Interest Rate Calculations: Calculating simple interest or compound interest often involves dividing total interest earned by the principal amount invested, a concept closely related to the quotient.
These financial applications emphasize the importance of understanding quotients for informed decision-making in financial matters.
3. Physics and Engineering:
In physics and engineering, quotients are frequently used. For instance:
- Average Velocity: Average velocity is calculated by dividing the total displacement by the time taken, a concept directly related to our central theme.
- Acceleration: Acceleration involves dividing the change in velocity by the time taken, again emphasizing the importance of the quotient.
- Force: In physics, force is often calculated using the concept of dividing momentum by time. This again hinges upon the concept of a quotient.
The abundance of applications in physics and engineering underlines the crucial role quotients play in understanding and modeling physical phenomena.
4. Computer Science:
Computer algorithms frequently employ division. For example, many sorting algorithms use division to determine sub-array sizes or to manage data structures effectively. Hash functions often involve modulo operations which rely on division concepts. The quotient is a fundamental component of many computer science operations.
5. Everyday Life:
Beyond the academic realm, the quotient plays a significant role in everyday life. Consider:
- Sharing: If you have 9 cookies and want to share them equally among 'x' friends, each friend receives 9/x cookies.
- Recipe Scaling: If a recipe calls for 9 cups of flour and you want to make a smaller portion, you would divide the amount by the scaling factor.
- Fuel Consumption: Calculating the fuel efficiency of a vehicle involves dividing the distance traveled by the amount of fuel consumed.
These examples show how naturally quotients appear in various aspects of daily life. Understanding this concept improves problem-solving skills in diverse situations.
The Importance of Understanding the Concept
The ability to understand and manipulate the concept of "the quotient of 9 and a number" is not just about solving mathematical problems; it's about developing a crucial mathematical skill that underpins problem-solving abilities in various real-world scenarios. Mastering this simple concept strengthens the foundation for tackling more complex mathematical challenges and enhances critical thinking skills. It demonstrates the power of basic mathematical concepts and their profound impact on various disciplines. Understanding the behavior of this simple quotient, especially as the divisor changes, enhances mathematical intuition and builds a strong understanding of fundamental mathematical relationships.
Conclusion
The seemingly simple concept of "the quotient of 9 and a number" is a cornerstone of mathematical understanding. Its representation in algebraic form, 9/x, allows for manipulation and analysis, enabling us to solve for unknowns, explore relationships, and apply it to a wide range of practical problems across diverse fields. From everyday tasks to complex scientific calculations and computer algorithms, the concept of the quotient permeates our understanding of the world. A strong grasp of this fundamental concept is essential for anyone seeking mathematical proficiency and effective problem-solving skills. Its importance cannot be overstated in the context of developing a solid mathematical foundation.
Latest Posts
Latest Posts
-
What Is The Gcf Of 16 And 18
May 10, 2025
-
How Many Bags Of Salt For A 15000 Gallon Pool
May 10, 2025
-
What Is 20 Percent Of 800 000
May 10, 2025
-
Besides 15 And 1 What Is One Factor Of 15
May 10, 2025
-
How To Convert Foot Pounds Into Inch Pounds
May 10, 2025
Related Post
Thank you for visiting our website which covers about The Quotient Of 9 And A Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.