What Fraction Is Equal To 4/10
Treneri
May 12, 2025 · 6 min read
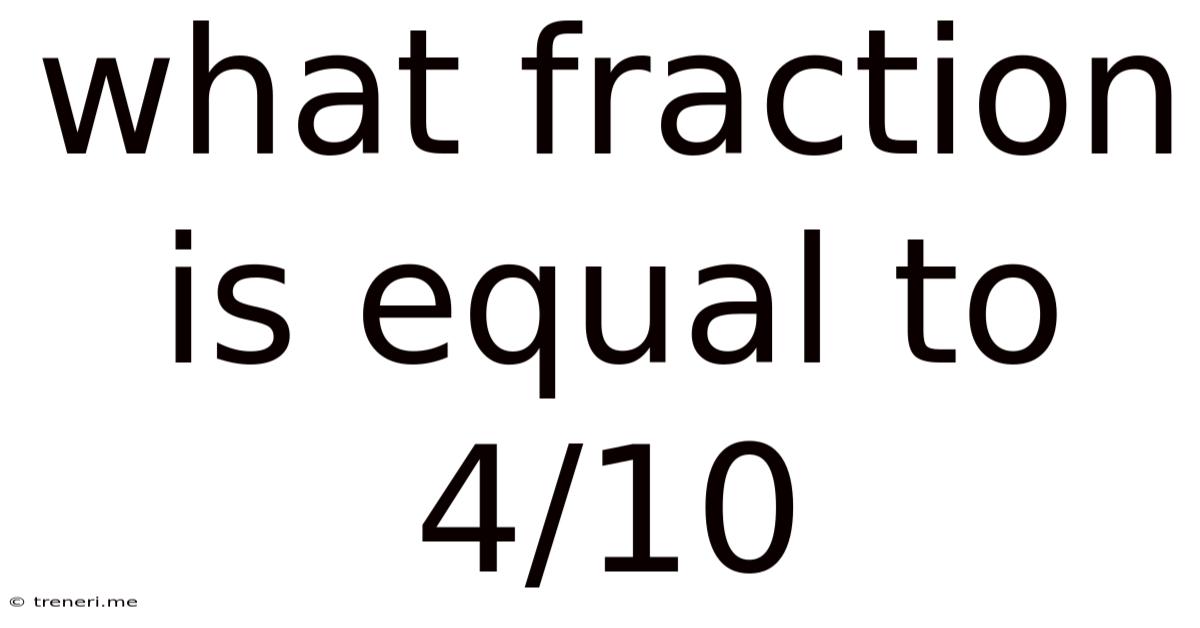
Table of Contents
What Fraction is Equal to 4/10? A Comprehensive Exploration of Equivalent Fractions
Understanding fractions is a fundamental skill in mathematics, essential for various applications in daily life and advanced studies. This article delves into the question, "What fraction is equal to 4/10?", exploring not just the answer but also the broader concept of equivalent fractions, simplification, and their practical applications. We'll cover different methods to find equivalent fractions and demonstrate how to apply this knowledge to solve real-world problems.
Finding Equivalent Fractions: The Core Concept
The fraction 4/10 represents four parts out of a total of ten equal parts. Equivalent fractions represent the same proportion or value but are expressed using different numerators and denominators. The key principle is that multiplying or dividing both the numerator and the denominator by the same non-zero number results in an equivalent fraction.
Think of it like slicing a pizza. If you have a pizza cut into 10 slices and you take 4, you've eaten 4/10 of the pizza. Now, imagine you cut that same pizza into 5 slices instead. You would still have eaten the same amount, only now you've eaten 2 out of 5 slices, which is 2/5. Both 4/10 and 2/5 represent the same portion of the pizza.
Method 1: Dividing by the Greatest Common Divisor (GCD)
The most efficient method to find the simplest equivalent fraction is by dividing both the numerator and the denominator by their Greatest Common Divisor (GCD). The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
For 4/10:
- Find the factors of 4: 1, 2, 4
- Find the factors of 10: 1, 2, 5, 10
- Identify the greatest common factor: 2
Now, divide both the numerator and the denominator by 2:
4 ÷ 2 = 2 10 ÷ 2 = 5
Therefore, the simplest equivalent fraction to 4/10 is 2/5.
This simplified fraction is also known as the fraction in its lowest terms. It's the most concise way to represent the proportion.
Method 2: Systematic Division
If finding the GCD isn't immediately apparent, you can systematically divide the numerator and denominator by common factors until no further simplification is possible.
Let's start with 4/10:
- We know that both 4 and 10 are even numbers, so they are both divisible by 2.
- Dividing both by 2 gives us 2/5.
- Now, check if there are any other common factors between 2 and 5. There aren't. Thus, 2/5 is the simplified fraction.
Understanding the Significance of Simplification
Simplifying fractions is crucial for several reasons:
- Clarity and Readability: Simplified fractions are easier to understand and compare. 2/5 is much clearer than 4/10.
- Efficiency in Calculations: Working with simplified fractions makes calculations significantly simpler and reduces the risk of errors. Imagine multiplying 4/10 by another fraction; it's easier with 2/5.
- Standardized Representation: In mathematics, presenting answers in the simplest form is a standard practice for consistency and ease of understanding.
Other Equivalent Fractions to 4/10
While 2/5 is the simplest equivalent fraction, infinitely many other equivalent fractions exist. We can generate them by multiplying both the numerator and the denominator by the same number.
For example:
- Multiplying both by 2: (4 x 2) / (10 x 2) = 8/20
- Multiplying both by 3: (4 x 3) / (10 x 3) = 12/30
- Multiplying both by 4: (4 x 4) / (10 x 4) = 16/40
And so on. All these fractions – 8/20, 12/30, 16/40, etc. – are equivalent to 4/10 and 2/5. They all represent the same proportion, but they are not in their simplest form.
Real-World Applications of Equivalent Fractions
Equivalent fractions appear frequently in everyday situations:
-
Cooking and Baking: Recipes often require fractions of ingredients. Understanding equivalent fractions allows you to adjust recipes based on the available quantities. For instance, if a recipe calls for 2/5 cup of sugar, and you only have a 1/10 cup measuring spoon, you know you need to use it four times (4/10 = 2/5).
-
Measurements: In various measurement systems, converting units often involves working with equivalent fractions. For instance, converting inches to feet requires understanding that 12 inches is equivalent to 1 foot.
-
Percentages: Percentages are essentially fractions with a denominator of 100. Converting a fraction to a percentage involves finding an equivalent fraction with a denominator of 100. For example, 2/5 can be converted to a percentage by finding an equivalent fraction with a denominator of 100: 40/100, which is 40%.
-
Data Analysis: In data analysis and statistics, representing proportions and ratios using fractions and their equivalent forms is common.
-
Financial Calculations: Many financial calculations, like calculating interest rates, involve working with fractions and their equivalents.
Beyond the Basics: Decimal Representation
Fractions can also be expressed as decimals. To convert 4/10 to a decimal, simply divide the numerator (4) by the denominator (10):
4 ÷ 10 = 0.4
This shows that 4/10, 2/5, and 0.4 all represent the same value. Understanding the relationship between fractions and decimals is essential for various mathematical operations and applications.
Visual Representations of Equivalent Fractions
Visual aids, such as diagrams or shapes, can help solidify the understanding of equivalent fractions. Imagine a rectangle divided into 10 equal parts; shading 4 parts visually demonstrates 4/10. Then, dividing that same rectangle into 5 equal parts and shading 2 shows the equivalence to 2/5. This visual representation reinforces the concept that the shaded area remains the same despite the different number of parts.
Common Mistakes to Avoid
A common mistake is incorrectly simplifying fractions by dividing only the numerator or only the denominator. Remember, both the numerator and the denominator must be divided by the same number to maintain the equivalence.
Another mistake is failing to find the greatest common divisor. This might lead to a simplified fraction that is not in its lowest terms, requiring further simplification steps.
Practice Problems
To reinforce your understanding, try simplifying the following fractions:
- 6/12
- 15/25
- 24/36
- 18/27
- 35/49
Conclusion
Understanding equivalent fractions is a cornerstone of mathematical fluency. The ability to simplify fractions and recognize their various representations, whether as simpler fractions or decimals, is crucial for success in mathematics and its numerous applications in various fields. By mastering this concept, one opens the door to a deeper understanding of ratios, proportions, and numerical relationships essential to navigating various quantitative aspects of our world. Remember the fundamental rule: multiplying or dividing both the numerator and denominator by the same non-zero number results in an equivalent fraction, and always aim to express your fraction in its simplest form for clarity and efficiency.
Latest Posts
Latest Posts
-
Cuanto Es 9 Grados En Fahrenheit
May 12, 2025
-
Work Out The Lengths Of Sides A And B
May 12, 2025
-
120 Ml Of Milk To Cups
May 12, 2025
-
What Material Is 19 Times As Dense As Water
May 12, 2025
-
140 Grams Of Flour In Cups
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Fraction Is Equal To 4/10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.