What Fractions Are Equal To 2/4
Treneri
May 11, 2025 · 5 min read
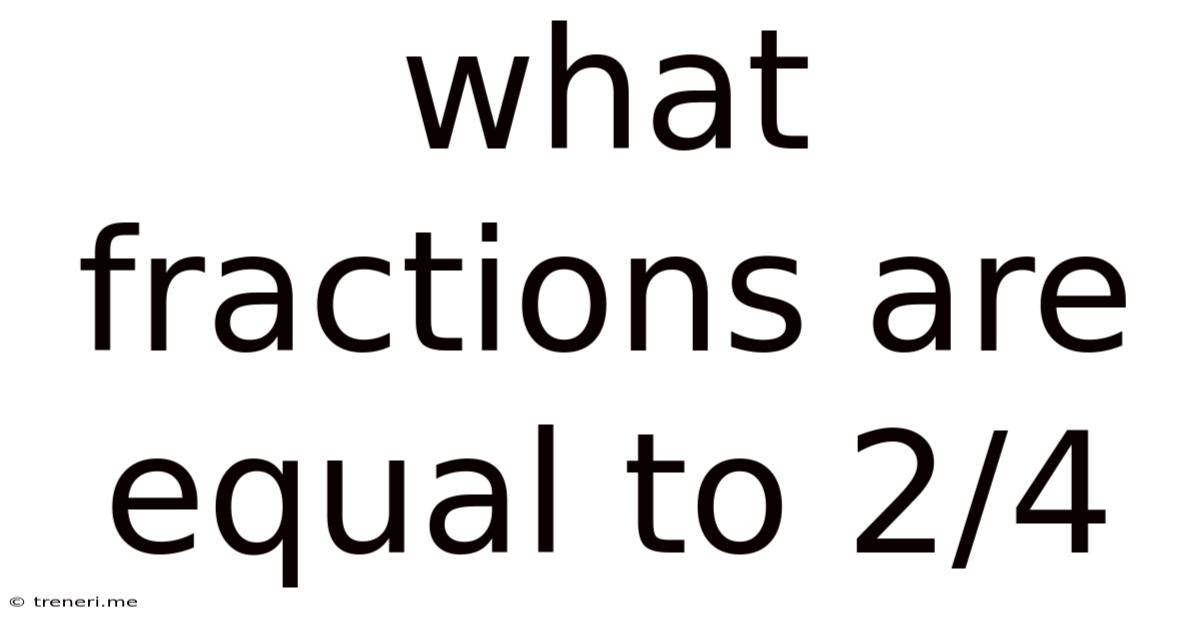
Table of Contents
What Fractions Are Equal to 2/4? A Deep Dive into Equivalent Fractions
Understanding equivalent fractions is a cornerstone of mathematical proficiency. This comprehensive guide delves into the fascinating world of fractions equal to 2/4, exploring various methods to identify them, their practical applications, and how to teach this concept effectively. We'll move beyond simple equivalence and explore the broader implications of simplifying fractions and understanding their representation on a number line.
Understanding the Basics: What are Equivalent Fractions?
Equivalent fractions represent the same portion or value, even though they appear different. They are essentially different ways of expressing the same ratio. Think of it like cutting a pizza: if you cut a pizza into four slices and take two, you've eaten half the pizza. If you cut the same pizza into eight slices and take four, you've still eaten half. Both 2/4 and 4/8 represent the same amount – one-half.
Key Concept: Equivalent fractions are created by multiplying or dividing both the numerator (top number) and the denominator (bottom number) by the same non-zero number. This process doesn't change the overall value of the fraction.
Finding Fractions Equal to 2/4: A Step-by-Step Approach
The fraction 2/4 is a simple fraction that can be easily simplified. To find equivalent fractions, we apply the core principle: multiply or divide both the numerator and the denominator by the same number.
1. Simplifying 2/4 to its Simplest Form:
The simplest form of a fraction is one where the numerator and denominator have no common factors other than 1. For 2/4, both the numerator (2) and the denominator (4) are divisible by 2.
2 ÷ 2 = 1 4 ÷ 2 = 2
Therefore, the simplest form of 2/4 is 1/2.
2. Generating Equivalent Fractions by Multiplying:
To find other equivalent fractions, we multiply both the numerator and the denominator of 1/2 (or 2/4) by the same number. Let's try a few examples:
- Multiplying by 2: (1 x 2) / (2 x 2) = 2/4 (This is our starting fraction)
- Multiplying by 3: (1 x 3) / (2 x 3) = 3/6
- Multiplying by 4: (1 x 4) / (2 x 4) = 4/8
- Multiplying by 5: (1 x 5) / (2 x 5) = 5/10
- Multiplying by 10: (1 x 10) / (2 x 10) = 10/20
- Multiplying by 100: (1 x 100) / (2 x 100) = 100/200
And so on! You can generate an infinite number of equivalent fractions by multiplying by any non-zero number.
3. Representing Equivalent Fractions on a Number Line:
Visualizing equivalent fractions on a number line reinforces the understanding that they represent the same point on the number line. All the fractions we've identified (1/2, 2/4, 3/6, 4/8, etc.) will all fall at the exact same point, halfway between 0 and 1.
The Importance of Simplifying Fractions
While there are infinitely many equivalent fractions for any given fraction, it's crucial to simplify fractions to their simplest form. This makes them easier to understand, compare, and use in calculations. Simplified fractions provide a more concise and efficient representation of the value.
Benefits of Simplification:
- Clarity: Simplified fractions are easier to read and interpret.
- Efficiency: Simpler fractions make calculations faster and less prone to errors.
- Comparison: Comparing simplified fractions is much easier than comparing complex ones.
Real-World Applications of Equivalent Fractions
Equivalent fractions are not just an abstract mathematical concept; they have many practical applications in everyday life:
-
Cooking and Baking: Recipes often require fractions of ingredients. Understanding equivalent fractions allows for adjustments based on the available quantities. For example, if a recipe calls for 1/2 cup of sugar and you only have a 1/4 cup measuring cup, you know you need two 1/4 cups.
-
Measurement: Converting units of measurement often involves working with equivalent fractions. For example, converting inches to feet or centimeters to meters.
-
Sharing and Division: Dividing things equally among people often involves fractions. Equivalent fractions help ensure fair distribution.
-
Data Analysis: In data analysis, representing proportions as fractions and simplifying them allows for clearer representation and comparison.
Teaching Equivalent Fractions Effectively
Teaching this concept requires a multi-faceted approach that combines visual aids, hands-on activities, and real-world examples.
Effective Teaching Strategies:
-
Visual Aids: Use diagrams, pictures, and manipulatives like fraction circles or bars to visually represent fractions and their equivalence.
-
Hands-on Activities: Engage students in activities that involve physically dividing objects and representing them as fractions. For example, have them divide a pizza into different numbers of slices and compare the portions.
-
Real-world Examples: Connect the concept of equivalent fractions to real-world scenarios they can relate to, like sharing food, measuring ingredients, or dividing tasks.
-
Games and Puzzles: Incorporate games and puzzles that involve fractions to make learning engaging and fun.
-
Step-by-Step Guidance: Provide clear and step-by-step instructions for simplifying and finding equivalent fractions.
Beyond the Basics: Exploring Further Concepts
Understanding equivalent fractions forms a foundation for more advanced mathematical concepts.
-
Fraction Operations: Adding, subtracting, multiplying, and dividing fractions require a solid understanding of equivalent fractions to find common denominators.
-
Ratio and Proportion: Equivalent fractions are directly related to ratios and proportions, which are fundamental concepts in algebra and higher-level mathematics.
-
Decimals and Percentages: Understanding equivalent fractions helps in converting between fractions, decimals, and percentages, which are essential skills in various fields.
Conclusion: Mastering the Art of Equivalent Fractions
The ability to identify and manipulate equivalent fractions is a valuable skill that transcends simple arithmetic. It underpins a deep understanding of number relationships, lays the groundwork for more advanced mathematical concepts, and finds practical application in numerous real-world scenarios. By employing a combination of visual aids, hands-on activities, and real-world connections, educators can effectively impart this crucial knowledge to students, enabling them to confidently navigate the world of fractions and beyond. Remember, the journey to mastering equivalent fractions starts with a solid understanding of the fundamental principles and a willingness to explore the many facets of this important concept. The seemingly simple question, "What fractions are equal to 2/4?" opens a door to a rich and expansive world of mathematical understanding.
Latest Posts
Latest Posts
-
What Is The Gcf Of 70 And 28
May 13, 2025
-
What Size Bbq Grill Do I Need
May 13, 2025
-
3 2 Rounded To The Nearest Tenth
May 13, 2025
-
What Is The Greatest Common Factor Of 72 And 84
May 13, 2025
-
How Many Weeks Is 121 Days
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Fractions Are Equal To 2/4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.