What Is 1 3 Of 10000
Treneri
May 11, 2025 · 4 min read
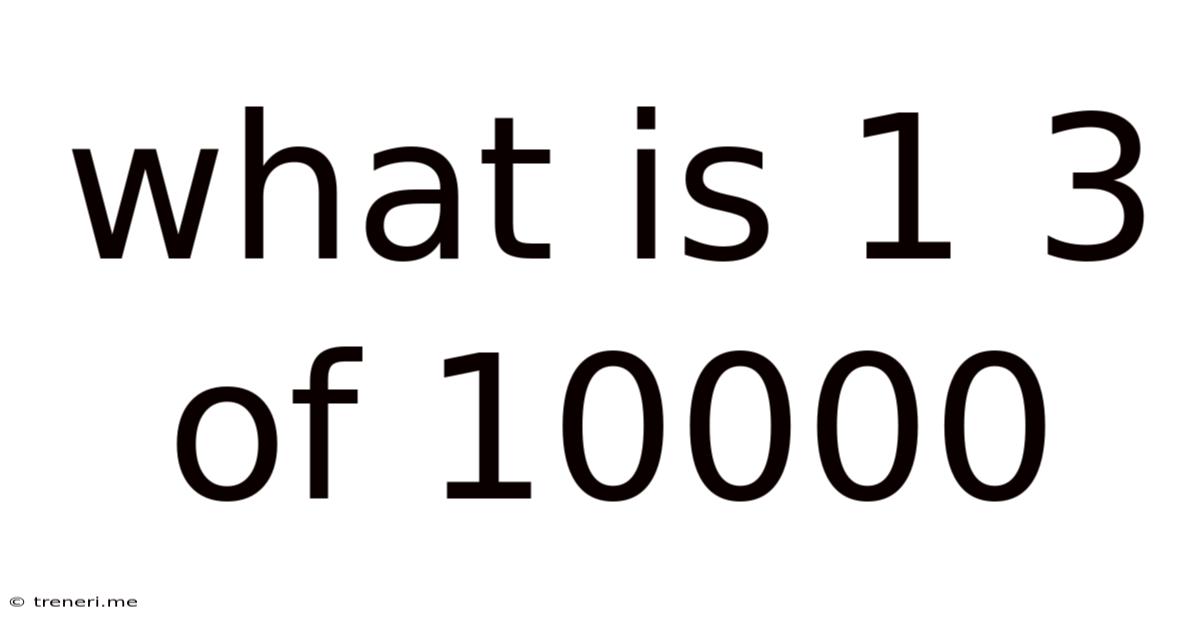
Table of Contents
What is 1/3 of 10000? A Comprehensive Exploration of Fractions and Their Applications
Finding a fraction of a number is a fundamental concept in mathematics with widespread applications in various fields. This article delves into the calculation of 1/3 of 10000, exploring the underlying principles, different methods of calculation, and practical examples showcasing its relevance in everyday life and professional contexts. We’ll also touch upon the importance of understanding fractions in broader mathematical contexts and their significance in fields ranging from finance to engineering.
Understanding Fractions: A Quick Refresher
Before we dive into the calculation, let's quickly refresh our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of parts the whole is divided into.
For example, in the fraction 1/3, 1 is the numerator and 3 is the denominator. This means we are considering one part out of a total of three equal parts.
Calculating 1/3 of 10000: Three Different Approaches
There are several ways to calculate 1/3 of 10000. Let's explore three common methods:
Method 1: Direct Division
The most straightforward approach is to divide 10000 by the denominator (3) and then multiply the result by the numerator (1). This is essentially the same as finding one-third of 10000.
10000 / 3 = 3333.333...
Therefore, 1/3 of 10000 is approximately 3333.33.
The repeating decimal indicates that 1/3 is a non-terminating decimal. This means the decimal representation goes on forever without repeating in a finite pattern. For practical purposes, we often round the result to a suitable number of decimal places, as shown above.
Method 2: Using Decimals
We can convert the fraction 1/3 into its decimal equivalent, which is approximately 0.3333. Then, we multiply this decimal by 10000:
0.3333 * 10000 = 3333
This method provides a slightly less precise result than direct division because we're using a rounded decimal approximation of 1/3. The precision depends on the number of decimal places used in the approximation of 1/3. Using more decimal places will result in a more accurate approximation.
Method 3: Understanding the Concept of Thirds
Visualizing the problem can be helpful. Imagine dividing 10000 into three equal parts. To find one of these parts (1/3), we perform the division:
10000 / 3 ≈ 3333.33
This provides the same result as the previous methods.
Practical Applications of Finding 1/3 of 10000
The concept of finding a fraction of a number, like calculating 1/3 of 10000, has numerous practical applications across various disciplines:
1. Finance and Budgeting:
- Profit Sharing: Imagine three partners in a business that made a profit of $10,000. Each partner would receive 1/3 of the profit, which is approximately $3333.33.
- Investment Allocation: An investor might decide to allocate 1/3 of their $10,000 investment portfolio to a specific asset class.
- Expense Distribution: If three individuals share a $10,000 expense equally, each would pay approximately $3333.33.
2. Engineering and Construction:
- Material Allocation: A construction project might require 10,000 bricks, and 1/3 of these are needed for a particular section of the building.
- Resource Management: In project planning, 1/3 of the total project budget or time might be allocated to a specific phase.
3. Data Analysis and Statistics:
- Sampling: Researchers might select 1/3 of a 10,000-item dataset for a pilot study.
- Data Representation: A chart or graph might represent 1/3 of a total dataset.
4. Everyday Life:
- Recipe Scaling: A recipe that calls for 1/3 of a cup of sugar for 3 servings could be scaled up for 10,000 servings (though this would be a very large recipe!).
- Resource Division: Fairly dividing resources among three people.
Beyond the Calculation: Expanding Mathematical Understanding
Understanding the calculation of 1/3 of 10000 extends beyond the simple arithmetic. It reinforces crucial mathematical concepts:
- Fractions and Decimals: The calculation involves converting a fraction to a decimal and understanding the concept of repeating decimals.
- Division: The core operation involved is division, a fundamental arithmetic operation.
- Approximation and Rounding: Dealing with repeating decimals necessitates understanding the concepts of approximation and rounding to appropriate levels of precision.
Mastering these concepts provides a strong foundation for more advanced mathematical studies.
Conclusion: The Significance of Fractions in a Numerical World
Calculating 1/3 of 10000, while seemingly a straightforward task, underscores the fundamental importance of fractional arithmetic in our daily lives and professional endeavors. The ability to accurately and efficiently perform such calculations is crucial for various tasks, ranging from simple budgeting to complex engineering projects. A solid grasp of fractions and their applications not only simplifies numerous calculations but also contributes to a broader understanding of mathematical principles and problem-solving strategies. This knowledge empowers you to approach a wide array of numerical challenges with confidence and efficiency, enhancing your abilities in both personal and professional spheres. Therefore, reinforcing your understanding of fraction arithmetic, including calculations like this one, remains a valuable investment in your mathematical literacy.
Latest Posts
Latest Posts
-
1 1 2 As A Improper Fraction
May 11, 2025
-
Find The Area Of The Given Figure
May 11, 2025
-
How Many Ounces Are In 2 And 1 2 Cups
May 11, 2025
-
Falta Quantos Dia Para O Ano Novo
May 11, 2025
-
Metacam 1 5 Mg Ml Dosage For Dogs
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 3 Of 10000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.