What Is 1 5 Times 2
Treneri
May 14, 2025 · 5 min read
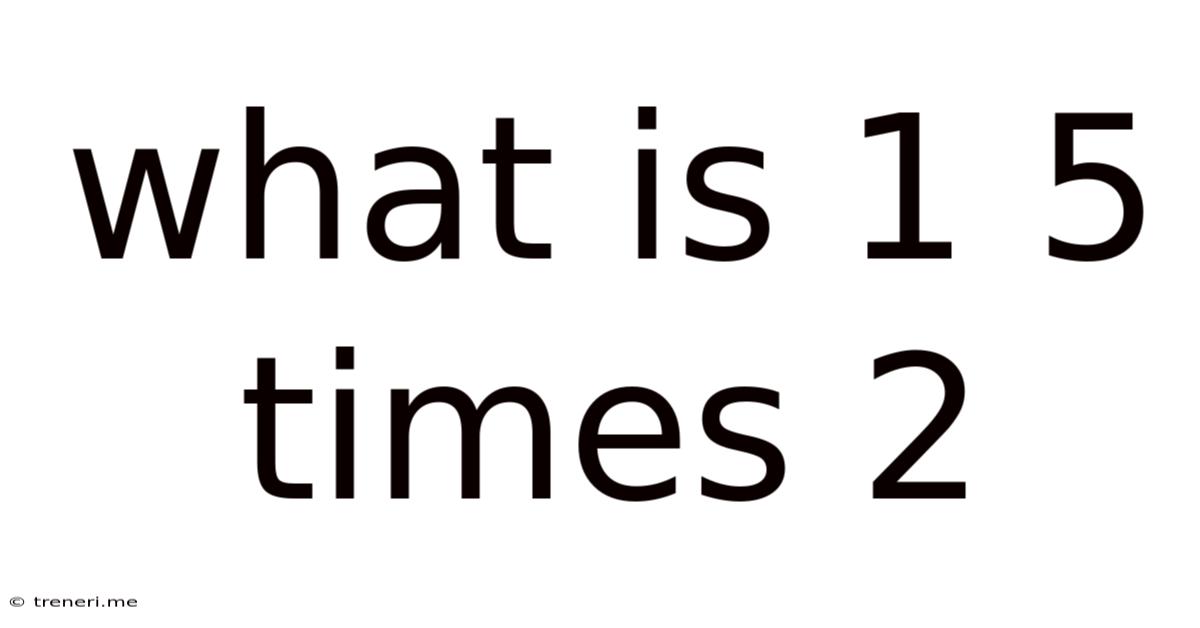
Table of Contents
What is 1.5 Times 2? A Deep Dive into Multiplication and its Applications
This seemingly simple question, "What is 1.5 times 2?", opens the door to a fascinating exploration of fundamental mathematical concepts and their widespread applications in everyday life. While the answer itself is straightforward (3), the process of arriving at it, and understanding the underlying principles, reveals a wealth of knowledge. This article delves into the intricacies of multiplication, specifically focusing on multiplying decimals and fractions, and explores its relevance across various fields.
Understanding Multiplication: Beyond Rote Learning
Multiplication, at its core, is repeated addition. When we say "1.5 times 2," we're essentially asking, "What is the result of adding 1.5 to itself two times?" This simple interpretation lays the foundation for grasping more complex multiplication problems. However, rote memorization alone isn't sufficient for a deep understanding. We need to explore the underlying principles to truly master this fundamental operation.
The Commutative Property: Order Doesn't Matter
One crucial property of multiplication is its commutative nature. This means that the order of the numbers being multiplied doesn't affect the final result. In our case, 1.5 times 2 is the same as 2 times 1.5. This seemingly trivial observation is incredibly powerful, allowing us to approach multiplication problems from different perspectives and choose the method that best suits our understanding.
The Associative Property: Grouping for Efficiency
The associative property allows us to group numbers in multiplication without altering the outcome. This is particularly helpful when dealing with multiple numbers. For instance, if we were to calculate (1.5 x 2) x 3, we could equally calculate 1.5 x (2 x 3). This property facilitates efficient calculation and simplifies complex expressions.
The Distributive Property: Breaking Down Complexity
The distributive property is a cornerstone of algebraic manipulation. It states that multiplying a number by a sum is equivalent to multiplying the number by each term in the sum and then adding the results. For example, 2 x (1 + 0.5) = (2 x 1) + (2 x 0.5) = 2 + 1 = 3. This property is invaluable in simplifying complex expressions and solving equations.
Multiplying Decimals: A Step-by-Step Approach
Multiplying decimals might seem daunting, but it's essentially the same as multiplying whole numbers, with an added step for handling the decimal point. Let's break down the calculation of 1.5 times 2:
-
Ignore the decimal point: Temporarily treat 1.5 as 15 and multiply it by 2. 15 x 2 = 30.
-
Count the decimal places: In 1.5, there's one decimal place (the digit after the decimal point).
-
Place the decimal point: In the result (30), move the decimal point one place to the left. This gives us 3.0, or simply 3.
Therefore, 1.5 times 2 equals 3.
Alternative Approach: Fraction Multiplication
We can also approach this problem using fractions. 1.5 can be expressed as the fraction 3/2. The multiplication then becomes:
(3/2) x 2 = (3 x 2) / 2 = 6 / 2 = 3
This fractional approach provides an alternative perspective and reinforces the concept of multiplication as repeated addition in a different context. It also demonstrates the flexibility of mathematical operations.
Real-World Applications of Multiplication: Beyond the Classroom
The seemingly simple operation of multiplying 1.5 by 2 has far-reaching applications in various fields:
Finance and Budgeting:
- Calculating costs: If a kilogram of apples costs $1.50, the cost of 2 kilograms would be 1.5 x 2 = $3.00.
- Calculating interest: Simple interest calculations often involve multiplying the principal amount by the interest rate and the time period.
- Calculating discounts: A 50% discount on a $30 item can be calculated as 0.5 x $30.
Engineering and Construction:
- Scaling blueprints: Architects and engineers frequently use multiplication to scale drawings and plans. A blueprint with dimensions multiplied by a factor represents a scaled-up or scaled-down version of the actual structure.
- Calculating material quantities: Determining the amount of materials needed for a construction project often involves multiplication, considering factors like length, width, and quantity.
Science and Measurement:
- Converting units: Converting between different units of measurement frequently involves multiplication. For example, converting centimeters to meters involves multiplying by 0.01.
- Calculating areas and volumes: Finding the area of a rectangle requires multiplying its length and width. Similarly, calculating the volume of a rectangular prism involves multiplying length, width, and height.
Everyday Life:
- Shopping: Calculating the total cost of multiple items involves multiplication. Buying 2 items at $1.50 each is a simple application of 1.5 x 2.
- Cooking: Following a recipe often requires multiplying ingredient quantities to scale a recipe up or down. Doubling a recipe involves multiplying all ingredient amounts by 2.
Advanced Concepts: Expanding on the Fundamentals
While the problem of 1.5 times 2 is relatively simple, its solution forms a building block for understanding more complex mathematical concepts:
Algebra:
Multiplication is a fundamental operation in algebra. It's crucial for solving equations, simplifying expressions, and working with variables. Understanding the distributive property and other properties of multiplication is essential for success in algebra and beyond.
Calculus:
Calculus relies heavily on the concept of limits, which involve infinitesimally small changes. Understanding multiplication at a fundamental level is vital for grasping the intricacies of derivatives and integrals, essential concepts in calculus.
Statistics and Probability:
Statistical analysis often involves multiplying probabilities or manipulating data sets. A solid understanding of multiplication forms the foundation for performing various statistical calculations and interpreting results.
Conclusion: The Power of a Simple Equation
The seemingly simple question, "What is 1.5 times 2?" encapsulates a wealth of mathematical knowledge. Understanding the underlying principles of multiplication, from its commutative and distributive properties to its applications in fractions and decimals, empowers us to tackle more complex mathematical problems and apply this fundamental operation to diverse real-world situations. From budgeting to building, from scientific calculations to everyday life, multiplication is an indispensable tool that shapes our understanding and interaction with the world around us. The answer, 3, is not just a numerical result; it's a gateway to a deeper comprehension of mathematics and its boundless applications.
Latest Posts
Latest Posts
-
Cuanto Es El 10 De 200
May 14, 2025
-
How Long Is 65 Days In Months
May 14, 2025
-
How Many Gallons Are In 1 Cubic Foot
May 14, 2025
-
Given Two Sides Of A Triangle Find The Third Side
May 14, 2025
-
Cuantas Libras Son En 58 Kilos
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 5 Times 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.