What Is 1 In 5000 As A Percentage
Treneri
May 15, 2025 · 4 min read
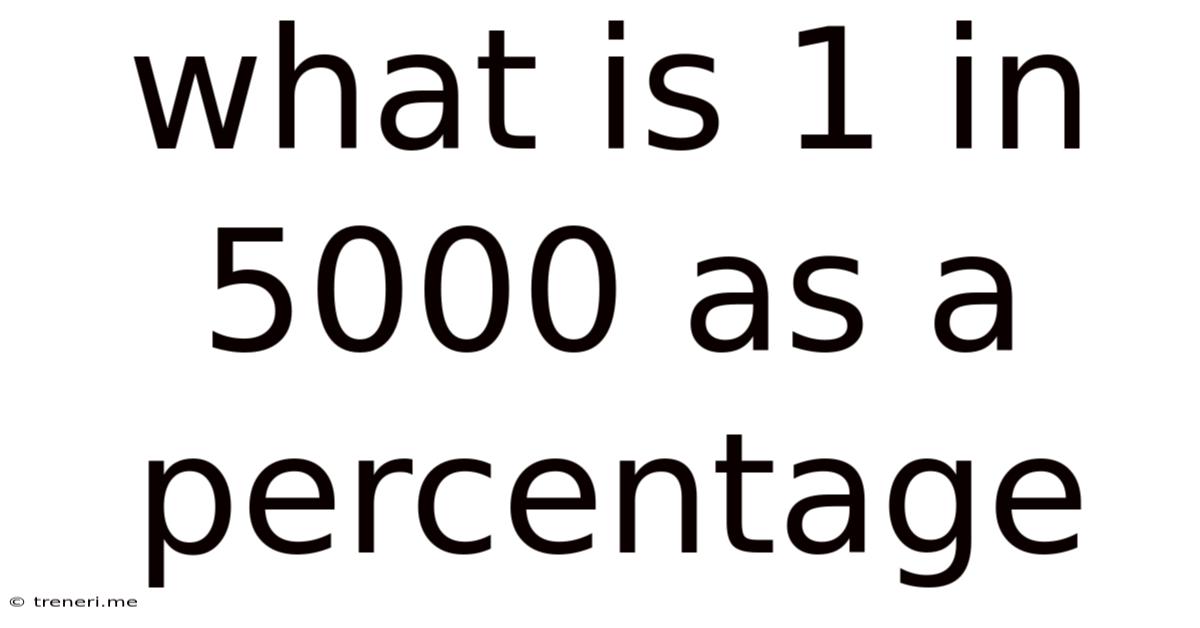
Table of Contents
What is 1 in 5000 as a Percentage? A Comprehensive Guide
Understanding the conversion of ratios to percentages is a fundamental skill in various fields, from statistics and finance to everyday life. This comprehensive guide will thoroughly explore how to calculate "1 in 5000" as a percentage, providing multiple approaches and contextual examples to solidify your understanding. We'll also delve into the practical applications and implications of this conversion.
Understanding Ratios and Percentages
Before diving into the calculation, let's clarify the basics. A ratio expresses the relative size of two or more values. In our case, "1 in 5000" is a ratio representing one occurrence out of a total of 5000 possibilities. A percentage, on the other hand, expresses a fraction as a portion of 100. Converting a ratio to a percentage involves finding the equivalent fraction with a denominator of 100.
Method 1: The Direct Calculation
The most straightforward approach involves converting the ratio to a decimal and then multiplying by 100%.
1. Convert the ratio to a decimal:
To do this, divide the numerator (1) by the denominator (5000):
1 / 5000 = 0.0002
2. Multiply by 100%:
This converts the decimal to a percentage:
0.0002 * 100% = 0.02%
Therefore, 1 in 5000 is equal to 0.02%.
Method 2: Using Proportions
Another effective method utilizes proportions to find the equivalent percentage. We can set up a proportion where 'x' represents the percentage:
1/5000 = x/100
To solve for 'x', cross-multiply:
5000x = 100
Now, divide both sides by 5000:
x = 100 / 5000 = 0.02
Again, multiplying by 100% gives us 0.02%.
Method 3: Understanding the Significance of Small Percentages
A percentage of 0.02% might seem insignificant at first glance. However, its importance depends heavily on the context. Consider these examples:
-
Medical Research: If a new drug has a 0.02% chance of causing a severe side effect, this represents a low but still potentially significant risk, especially when considering large populations. Pharmaceutical companies meticulously analyze such low probabilities to ensure patient safety. The rarity of the side effect doesn't negate its potential impact.
-
Manufacturing Defects: In a high-volume manufacturing process, a 0.02% defect rate might translate into a substantial number of faulty products. While seemingly small, this rate could necessitate costly rework, quality control measures, and potentially, product recalls. Statistical process control (SPC) is used to continuously monitor and reduce such low defect rates.
-
Lottery Odds: The odds of winning a particular lottery might be 1 in 5000. While the probability is extremely low (0.02%), the potential reward can still attract many participants. Understanding the low probability helps in setting realistic expectations.
-
Environmental Science: In the analysis of environmental pollutants, a 0.02% change in a critical parameter could signal a significant trend, especially when observed over time. Small, seemingly insignificant changes can point to larger environmental shifts.
-
Financial Modeling: In complex financial models, such as those used in risk management or portfolio optimization, even small percentages like 0.02% can contribute to substantial changes in the overall outcome. The cumulative effect of small probabilities needs careful analysis.
Practical Applications and Implications
The conversion of "1 in 5000" to 0.02% has numerous practical applications across various domains:
-
Risk Assessment: Businesses use this type of calculation to assess the risk associated with different scenarios. Understanding the probability of an event allows for better risk management strategies.
-
Quality Control: Manufacturers constantly monitor defect rates to ensure product quality. Converting defect ratios to percentages helps them track progress and identify areas for improvement.
-
Data Analysis: Statisticians use percentage conversions to interpret data and present findings in a clear and concise manner. Percentages provide a standardized way of comparing proportions across different datasets.
-
Probability and Statistics: This conversion is fundamental to understanding probabilities, and it's crucial in calculating confidence intervals and conducting hypothesis tests.
-
Decision Making: In many decision-making processes, whether personal or professional, understanding probabilities as percentages helps in evaluating the potential outcomes of different choices.
Beyond the Calculation: Interpreting the Result
While the numerical result (0.02%) is straightforward, understanding its implications requires careful consideration of the context. The small percentage doesn't automatically mean it's negligible. Factors like the potential consequences of the event (e.g., the severity of a side effect, the cost of a manufacturing defect) heavily influence its significance.
Common Mistakes to Avoid
-
Incorrect decimal placement: A common error is misplacing the decimal point during the conversion. Double-check your calculations to ensure accuracy.
-
Confusing ratios with percentages: Remember that a ratio expresses a relationship between two numbers, while a percentage is always relative to 100.
-
Oversimplification: Don't overlook the context. A small percentage might have significant consequences depending on the situation.
Conclusion: The Power of Percentage Conversion
Converting a ratio like "1 in 5000" into a percentage (0.02%) might appear simple, but its application is far-reaching. Understanding this fundamental conversion allows for clear communication, informed decision-making, and effective analysis across numerous fields. By grasping the mathematical process and appreciating the contextual nuances, you gain a powerful tool for interpreting data and tackling real-world problems. Remember to always consider the implications of the result within its specific context to avoid misinterpretations and ensure accurate conclusions.
Latest Posts
Latest Posts
-
2001 Al 2024 Cuantos Anos Son
May 15, 2025
-
What Is A Fraction Equivalent To 2 5
May 15, 2025
-
2400 Feet Is How Many Miles
May 15, 2025
-
How Many Ounces Are In 7 Cup
May 15, 2025
-
3 Tbsp Of Flour In Grams
May 15, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 In 5000 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.