What Is 10 Percent Of 29
Treneri
May 13, 2025 · 5 min read
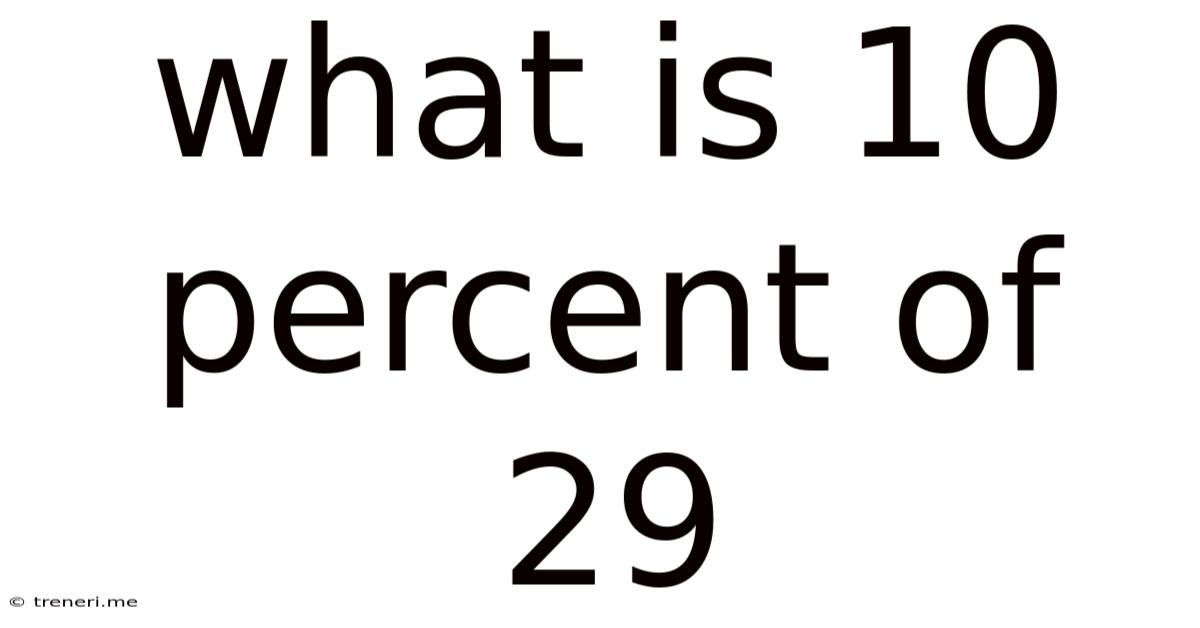
Table of Contents
What is 10 Percent of 29? A Deep Dive into Percentages and Their Applications
Finding 10 percent of 29 might seem like a simple arithmetic problem, easily solvable with a calculator. However, understanding the underlying concepts of percentages and their widespread applications unveils a much richer mathematical landscape. This article will not only answer the question directly but will also delve into the practical uses of percentages, exploring different methods of calculation, and highlighting their importance in various fields.
Calculating 10% of 29: The Basics
The most straightforward way to calculate 10% of 29 is to convert the percentage to a decimal and multiply. 10% is equivalent to 0.10 (or simply 0.1). Therefore:
0.10 x 29 = 2.9
Therefore, 10 percent of 29 is 2.9.
This simple calculation is the foundation for understanding more complex percentage problems.
Understanding Percentages: Beyond the Basics
Percentages are a way of expressing a number as a fraction of 100. They provide a standardized method for comparing proportions and making relative comparisons. The term "percent" itself is derived from the Latin "per centum," meaning "out of one hundred."
Key Concepts:
- Percentage: A ratio expressed as a fraction of 100.
- Decimal Equivalent: The decimal representation of a percentage (e.g., 10% = 0.10).
- Fraction Equivalent: The fractional representation of a percentage (e.g., 10% = 10/100 = 1/10).
Understanding these fundamental concepts is crucial for solving a wide range of percentage problems, from calculating discounts to understanding financial reports.
Alternative Methods for Calculating Percentages
While the decimal method is often the most efficient, other approaches can be equally effective, especially for mental calculations or scenarios where a calculator isn't readily available.
Method 1: Using Fractions
As mentioned earlier, percentages can be expressed as fractions. 10% is equivalent to 1/10. Therefore, finding 10% of 29 can be calculated as:
(1/10) x 29 = 29/10 = 2.9
This fractional method can be particularly helpful when dealing with percentages like 25% (1/4), 50% (1/2), or 75% (3/4), where the fractional equivalents are easily recognizable.
Method 2: Finding 1% and Scaling Up
Another approach involves first calculating 1% of the number and then scaling it up to the desired percentage. 1% of 29 is:
29/100 = 0.29
To find 10%, we simply multiply this value by 10:
0.29 x 10 = 2.9
This method is useful for mental calculations and for quickly estimating percentages.
Real-World Applications of Percentages
Percentages are ubiquitous in everyday life, permeating numerous fields and impacting our daily decisions.
1. Finance and Economics:
- Interest Rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts. Understanding compound interest, which involves calculating interest on both the principal and accumulated interest, is crucial for financial planning.
- Investment Returns: Investment performance is often expressed as a percentage return on investment (ROI). This metric helps investors assess the profitability of their investments.
- Inflation and Economic Growth: Economists use percentages to track inflation rates, economic growth, and other macroeconomic indicators. These figures help policymakers make informed decisions about monetary and fiscal policy.
- Taxes: Taxes are frequently levied as a percentage of income, sales, or property value. Understanding tax rates is essential for financial planning and compliance.
- Discounts and Sales: Businesses use percentages to advertise discounts and sales, enticing customers with attractive price reductions.
2. Science and Statistics:
- Data Analysis: Percentages are frequently used in data analysis to represent proportions and make comparisons within datasets. Statistical measures like percentage change and percentage point change are commonly employed to analyze trends and patterns.
- Scientific Experiments: Scientists use percentages to express the success rates of experiments, the concentrations of substances, and other relevant parameters.
3. Everyday Life:
- Tipping: Restaurant patrons often calculate tips as a percentage of the total bill.
- Sales Tax: Sales tax is usually expressed as a percentage of the purchase price.
- Nutritional Information: Food labels often display nutritional information as percentages of recommended daily allowances.
- Grades and Scores: Academic grades and test scores are often represented as percentages.
Advanced Percentage Calculations
Beyond the simple calculations presented earlier, many more complex percentage problems exist, requiring a deeper understanding of mathematical principles.
Calculating Percentage Change:
Percentage change measures the relative change between two values over time. The formula for percentage change is:
(New Value - Old Value) / Old Value * 100%
For example, if a stock price increases from $20 to $25, the percentage change is:
(25 - 20) / 20 * 100% = 25%
This metric is crucial for analyzing trends and understanding the rate of growth or decline.
Calculating Percentage Increase/Decrease:
Similar to percentage change, percentage increase or decrease focuses on the magnitude of the change as a percentage of the original value. Calculating a percentage increase involves adding the percentage change to the original value, while a percentage decrease involves subtracting it.
Finding the Original Value:
Given a final value and a percentage increase or decrease, you can work backward to find the original value. This often involves solving algebraic equations.
Conclusion: The Enduring Importance of Percentages
The simple question, "What is 10 percent of 29?" opens the door to a vast realm of mathematical concepts and real-world applications. Mastering the art of percentage calculation is not only crucial for academic success but also for navigating the complexities of everyday life, from managing personal finances to understanding global economic trends. From calculating discounts to interpreting statistical data, percentages provide a powerful tool for understanding and quantifying relative proportions. By understanding the core concepts and various methods of calculation, individuals can confidently tackle a wide range of percentage problems and unlock a deeper understanding of the world around them. The seemingly simple calculation of 10% of 29 serves as a powerful reminder of the far-reaching influence of percentages in our lives.
Latest Posts
Latest Posts
-
Cuantos Dias Faltan Para El 23 De Enero
May 13, 2025
-
Greatest Common Factor Of 24 And 9
May 13, 2025
-
1 Cubic Yard Of Concrete Weight
May 13, 2025
-
Whats The Difference Between Milliliters And Milligrams
May 13, 2025
-
How Many Calories Do I Burn Biking 20 Miles
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Is 10 Percent Of 29 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.