What Is 100 Percent Of 100
Treneri
May 12, 2025 · 4 min read
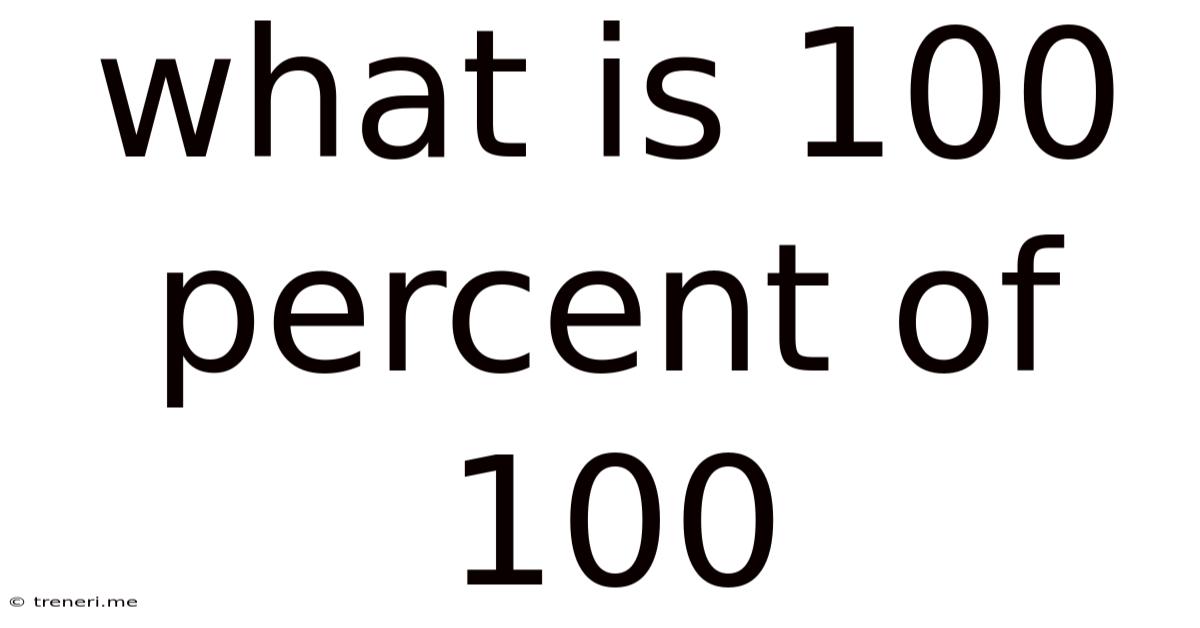
Table of Contents
What is 100 Percent of 100? A Deep Dive into Percentages and Their Applications
The question, "What is 100 percent of 100?" seems deceptively simple. At first glance, the answer is a straightforward 100. However, exploring this seemingly basic calculation opens a door to a deeper understanding of percentages, their mathematical foundation, and their widespread applications in various fields. This article delves into the concept of percentages, explains how to calculate them, explores their practical uses, and addresses common misconceptions.
Understanding Percentages: The Foundation
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" and is derived from the Latin phrase "per centum". The symbol used to represent percentages is the percent sign, %. Therefore, 100% represents 100 out of 100, or the whole. This is the key to understanding our initial question.
Calculating Percentages: The Formula
The basic formula for calculating a percentage is:
(Part / Whole) * 100% = Percentage
Where:
- Part: Represents the specific portion of the whole you're interested in.
- Whole: Represents the total amount or the entire quantity.
- Percentage: The resulting value expressed as a percentage.
Let's apply this formula to our initial question: "What is 100 percent of 100?"
In this case:
- Part: We're looking for 100% of a number, so the part is unknown (let's call it 'x').
- Whole: The whole is 100.
- Percentage: This is given as 100%.
Therefore, the equation becomes:
(x / 100) * 100% = 100%
To solve for 'x', we can simplify the equation:
- x = 100 * (100%/100%)
- x = 100 * 1
- x = 100
Thus, 100 percent of 100 is indeed 100.
Beyond the Basics: Exploring Different Percentage Calculations
While the initial question provides a simple answer, understanding percentages extends far beyond this basic example. Let's explore different scenarios and calculations involving percentages.
Calculating a Percentage of a Number:
This is the most common application of percentages. For example, "What is 25% of 80?"
Using the formula:
(Part / Whole) * 100% = Percentage
We have:
- Part: Unknown (x)
- Whole: 80
- Percentage: 25%
The equation becomes:
(x / 80) * 100% = 25%
Solving for x:
- x = (25%/100%) * 80
- x = 0.25 * 80
- x = 20
Therefore, 25% of 80 is 20.
Finding the Percentage One Number Represents of Another:
This involves determining what percentage one number represents of another. For instance, "What percentage is 15 of 60?"
Using the formula:
(Part / Whole) * 100% = Percentage
We have:
- Part: 15
- Whole: 60
- Percentage: Unknown (x)
The equation becomes:
(15 / 60) * 100% = x
Solving for x:
- x = (15/60) * 100%
- x = 0.25 * 100%
- x = 25%
Therefore, 15 represents 25% of 60.
Increasing or Decreasing a Number by a Percentage:
This is frequently used in scenarios involving price changes, discounts, or growth rates. For example, "Increase 50 by 10%".
To increase 50 by 10%, we first calculate 10% of 50:
- 10% of 50 = (10%/100%) * 50 = 5
Then, we add this result to the original number:
- 50 + 5 = 55
Therefore, increasing 50 by 10% results in 55.
Similarly, to decrease a number by a percentage, we calculate the percentage decrease and subtract it from the original number.
Real-World Applications of Percentages
Percentages are essential tools in numerous fields, including:
Finance and Business:
- Interest rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts.
- Profit margins: Businesses determine profitability by calculating profit margins as a percentage of sales.
- Taxes: Governments use percentages to levy taxes on income, sales, and other sources.
- Discounts and sales: Retailers use percentages to advertise discounts and sales.
- Investment returns: Investors track their investment performance using percentage returns.
Science and Statistics:
- Data analysis: Percentages are crucial for representing data and trends in scientific studies and statistical analyses.
- Probability and statistics: Probabilities are often expressed as percentages.
- Experimental results: Scientists use percentages to express the success rate of experiments.
Everyday Life:
- Tips and gratuities: People often calculate tips as a percentage of the bill amount.
- Nutrition labels: Food labels use percentages to indicate the percentage of daily recommended values for various nutrients.
- Sales tax: Consumers use percentages to calculate sales tax on purchases.
Common Misconceptions about Percentages
Several misconceptions can lead to errors in percentage calculations:
- Confusing percentage change with percentage points: A change from 10% to 20% is a 100% increase, but it's also a 10 percentage point increase. These are different measures.
- Incorrectly calculating percentage increases and decreases: Always remember to base percentage changes on the original value, not the changed value.
- Assuming percentages are additive: For example, a 10% increase followed by a 10% decrease does not result in the original value, due to the changing base value.
Conclusion: Mastering Percentages for Success
Understanding percentages is a fundamental skill with widespread applications. While the answer to "What is 100 percent of 100?" might seem obvious, exploring this question reveals the underlying principles and practical uses of percentages. By mastering the concepts and avoiding common misconceptions, individuals can confidently apply percentage calculations in various aspects of their personal and professional lives. The ability to accurately interpret and calculate percentages is a powerful tool for informed decision-making and problem-solving in a data-driven world. From financial planning to scientific research, a solid grasp of percentages empowers individuals and organizations to succeed.
Latest Posts
Latest Posts
-
2 3 3 8 In Fraction Form
May 12, 2025
-
How Many Months Is 199 Days
May 12, 2025
-
Inches To Ring Size Converter Mens
May 12, 2025
-
Lcm Of 2 4 And 7
May 12, 2025
-
Cuanto Falta Para El 14 De Marzo
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 100 Percent Of 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.