What Is 15 Percent Of 24
Treneri
May 12, 2025 · 5 min read
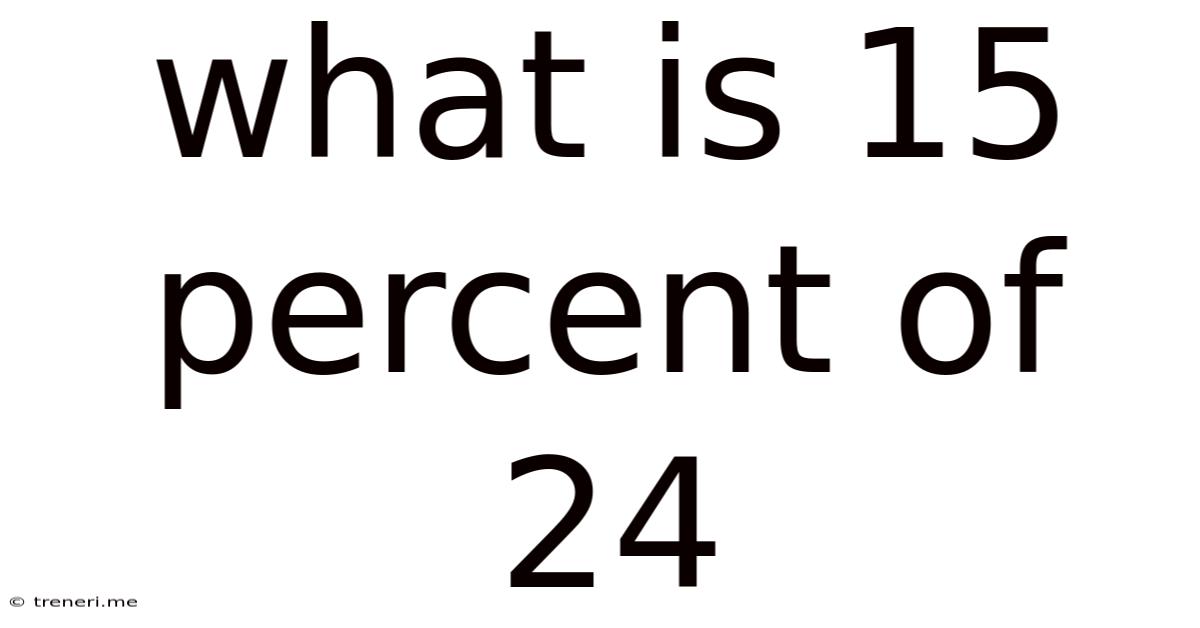
Table of Contents
What is 15 Percent of 24? A Deep Dive into Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from everyday budgeting and shopping to complex financial analysis and scientific research. This article will not only answer the question, "What is 15 percent of 24?" but will also explore the underlying concepts, methods, and practical applications of percentage calculations, making you comfortable tackling similar problems in the future.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" ( per cent). Therefore, 15% means 15 out of 100, or 15/100, which can be simplified to 3/20 as a fraction or 0.15 as a decimal.
Understanding this fundamental relationship is crucial for solving percentage problems. Knowing that a percentage is essentially a fraction allows us to use various mathematical techniques to find solutions.
Method 1: Using the Basic Percentage Formula
The most straightforward method to calculate a percentage of a number is to use the basic percentage formula:
Percentage = (Part / Whole) x 100
Alternatively, if you want to find the part, you would use this slightly rearranged formula:
Part = (Percentage / 100) x Whole
Let's apply this to our problem: "What is 15 percent of 24?"
Here, the Percentage is 15, and the Whole is 24. We want to find the Part. Substituting these values into the formula:
Part = (15 / 100) x 24
Part = 0.15 x 24
Part = 3.6
Therefore, 15 percent of 24 is 3.6.
Method 2: Using Proportions
Another effective method involves setting up a proportion. A proportion is an equation that states that two ratios are equal. We can represent the problem as:
15/100 = x/24
Where 'x' represents the unknown value (15% of 24). To solve for 'x', we cross-multiply:
15 x 24 = 100 x x
360 = 100x
Now, divide both sides by 100:
x = 360 / 100
x = 3.6
This method confirms our previous result: 15 percent of 24 is 3.6.
Method 3: Using Decimal Equivalents
As we established earlier, 15% is equivalent to the decimal 0.15. We can directly multiply this decimal by the whole number to find the percentage:
0.15 x 24 = 3.6
This method is quick and efficient, particularly when dealing with simpler percentage calculations.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in numerous real-world scenarios:
1. Finance and Budgeting:
- Calculating interest: Interest rates on loans, savings accounts, and investments are expressed as percentages.
- Determining discounts: Sales and discounts in stores are often advertised as percentage reductions (e.g., 20% off).
- Calculating taxes: Sales tax, income tax, and other taxes are usually calculated as a percentage of the total amount.
- Analyzing investment returns: Investment performance is often expressed as a percentage return on investment.
2. Retail and Sales:
- Calculating profit margins: Businesses use percentages to determine their profit margin (profit as a percentage of revenue).
- Pricing strategies: Retailers use percentages to determine pricing based on cost and desired profit margin.
- Calculating markups: Markups on products are frequently expressed as percentages.
- Analyzing sales data: Sales trends and growth are often expressed as percentages.
3. Science and Statistics:
- Calculating statistical measures: Many statistical measures, such as percentages, are used to analyze data and make inferences.
- Representing data: Percentages are used to represent proportions and relationships in data visually through charts and graphs.
- Scientific experiments: Percentages are used in experiments to quantify results and draw conclusions.
4. Everyday Life:
- Calculating tips: Restaurant tips are often calculated as a percentage of the bill.
- Understanding nutritional information: Nutritional labels on food products list percentages of daily recommended values for various nutrients.
- Comparing prices: Percentages help in comparing prices of different products or services.
Advanced Percentage Calculations
While the examples above cover basic percentage calculations, more complex scenarios might require multiple steps. For instance:
- Calculating percentage increase or decrease: This involves finding the difference between two values and expressing that difference as a percentage of the original value.
- Finding the original value after a percentage change: This involves working backward from the final value to find the original value before the percentage change was applied.
- Calculating compound interest: This involves calculating interest on both the principal amount and accumulated interest from previous periods.
Mastering Percentage Calculations: Tips and Tricks
Here are some tips to improve your percentage calculation skills:
- Practice regularly: Consistent practice is key to mastering any mathematical skill. Solve various percentage problems to build confidence and speed.
- Use different methods: Try different calculation methods (formula, proportions, decimals) to find the one that suits you best.
- Understand the concepts: Make sure you have a solid understanding of the underlying concepts of percentages and fractions.
- Use online calculators (for verification only): While online calculators can be helpful for verification, it's crucial to understand the underlying concepts and be able to solve problems manually.
- Break down complex problems: For more complex problems, break them down into smaller, manageable steps.
Conclusion: Beyond the Answer
While we have definitively answered the question, "What is 15 percent of 24?" (which is 3.6), this article has gone beyond a simple numerical answer. We have explored the core principles of percentage calculations, demonstrating multiple methods to reach the solution and highlighting their extensive applications across diverse fields. By understanding the underlying concepts and practicing regularly, you will not only be able to solve percentage problems efficiently but also apply this crucial skill to various aspects of your personal and professional life. The ability to confidently handle percentages empowers you to make informed decisions, analyze data effectively, and navigate the numerical world with greater ease and accuracy.
Latest Posts
Latest Posts
-
307 451 Rounded To The Nearest Thousand
May 12, 2025
-
Highest Common Factor Of 28 And 70
May 12, 2025
-
How Many Weeks Is 80 Hours
May 12, 2025
-
What Uv Is Good To Tan
May 12, 2025
-
What Is The Greatest Common Factor Of 27 And 72
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 15 Percent Of 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.