What Is 2/1 As A Fraction
Treneri
May 13, 2025 · 5 min read
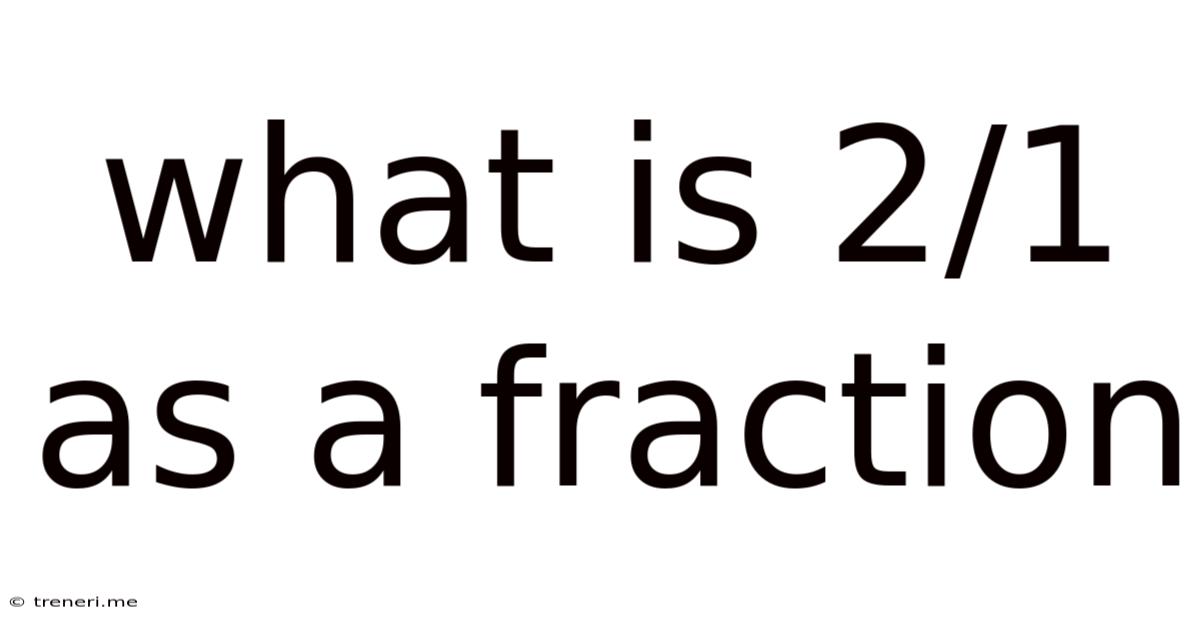
Table of Contents
What is 2/1 as a Fraction? Understanding Fractions and Their Representations
The question "What is 2/1 as a fraction?" might seem deceptively simple. After all, fractions are a fundamental part of mathematics. However, understanding the intricacies of fractions, especially how they represent division and their relationship to whole numbers, is crucial for a solid mathematical foundation. This article delves deep into the meaning of 2/1, exploring its various representations and applications within broader mathematical concepts.
Deconstructing the Fraction 2/1
The fraction 2/1 is composed of two key components: the numerator (2) and the denominator (1). The numerator indicates the number of parts we have, while the denominator indicates the number of equal parts the whole is divided into. In the case of 2/1, we have two parts out of a whole that has been divided into only one part. This immediately suggests that 2/1 represents a whole number, not a fraction in the traditional sense of representing a portion less than one.
Fractions as Division
A fundamental understanding of fractions involves recognizing that a fraction is essentially a division problem. The fraction a/b is equivalent to a divided by b. Applying this to 2/1, we see that 2/1 = 2 ÷ 1 = 2. This clearly shows that 2/1 is simply the whole number 2.
Visual Representation of 2/1
Imagine a single pizza. If we represent the whole pizza as 1, then 2/1 represents having two whole pizzas. There's no need to divide a single pizza into parts; we have two complete units. This visual example reinforces the concept that 2/1 is not a fraction representing a portion of a whole, but rather a representation of two whole units.
Equivalent Fractions and Simplification
While 2/1 is the simplest form, it's important to understand the concept of equivalent fractions. Equivalent fractions represent the same value, even though they appear different. For instance, 4/2, 6/3, 8/4, and indeed any fraction where the numerator is double the denominator, are all equivalent to 2/1. This is because all these fractions simplify to 2 when you divide the numerator by the denominator.
The process of simplifying fractions involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by this GCD. In the case of 2/1, the GCD of 2 and 1 is 1, so the fraction is already in its simplest form.
Illustrative Examples of Equivalent Fractions
Let's illustrate with some examples:
- 4/2: The GCD of 4 and 2 is 2. Dividing both the numerator and denominator by 2 gives us 2/1.
- 6/3: The GCD of 6 and 3 is 3. Dividing both the numerator and denominator by 3 gives us 2/1.
- 10/5: The GCD of 10 and 5 is 5. Dividing both by 5 results in 2/1.
All of these examples highlight the concept of equivalent fractions and how simplification leads to the simplest form, which in this case is 2/1, representing the whole number 2.
2/1 in Different Mathematical Contexts
The representation 2/1, although seemingly straightforward, plays a significant role in various mathematical operations and contexts:
Improper Fractions and Mixed Numbers
The term "improper fraction" refers to a fraction where the numerator is greater than or equal to the denominator. 2/1 is an example of an improper fraction. Improper fractions can be converted to mixed numbers, which combine a whole number and a proper fraction (where the numerator is less than the denominator). However, in the case of 2/1, the conversion is trivial: it's simply the whole number 2.
Ratios and Proportions
Fractions are often used to represent ratios. For example, if there are 2 apples and 1 orange, the ratio of apples to oranges is 2:1, which can also be expressed as the fraction 2/1. This demonstrates the versatile nature of fractions in expressing comparisons between quantities.
Algebraic Expressions
In algebra, fractions are used extensively. The fraction 2/1 might appear in algebraic expressions as a coefficient or part of a larger equation. Its simplicity makes it easy to manipulate and solve within algebraic contexts.
Expanding on the Concept of Whole Numbers
Understanding 2/1 helps reinforce the idea that whole numbers are a subset of rational numbers. Rational numbers are numbers that can be expressed as a fraction a/b, where a and b are integers and b is not zero. Since 2/1 fits this definition, it's evident that whole numbers are a specific type of rational number.
Practical Applications of Understanding 2/1
The seemingly simple concept of 2/1 has practical implications in various real-world scenarios:
- Recipe scaling: If a recipe calls for 1 cup of flour and you want to double it, you need 2 cups, represented by the fraction 2/1.
- Unit conversion: Converting units often involves fractions. For instance, converting feet to yards might involve multiplying by the fraction 1 yard/3 feet (which is equivalent to 1/3). While not directly 2/1, understanding the concept of fractions in these conversions is vital.
- Data interpretation: Understanding fractions is essential for interpreting data presented in charts and graphs, which often use fractions or ratios to represent portions of a whole.
Conclusion: The Significance of 2/1 in Mathematics
The fraction 2/1, while a simple representation of the whole number 2, provides a crucial link between the concepts of whole numbers, fractions, division, and ratios. It highlights the versatility of fractions and how they are fundamental building blocks within broader mathematical concepts. Understanding 2/1 and similar fractions solidifies foundational mathematical skills and opens the door to more advanced mathematical studies. The ability to represent a whole number as a fraction is a key stepping stone in understanding more complex fraction operations and their applications in numerous fields. From simple calculations to complex data analysis, the principles touched upon here are essential for mathematical literacy.
Latest Posts
Latest Posts
-
Cuantos Dias Faltan Para El 30 De Mayo
May 13, 2025
-
Mortgage Calculator Bi Weekly With Extra Payments
May 13, 2025
-
60 Ml Equals How Many Tablespoons
May 13, 2025
-
Cuanto Son 30 Oz En Litros
May 13, 2025
-
What Percentage Of 36 Is 24
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Is 2/1 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.