What Is 2.5 Percent Of 100
Treneri
May 10, 2025 · 5 min read
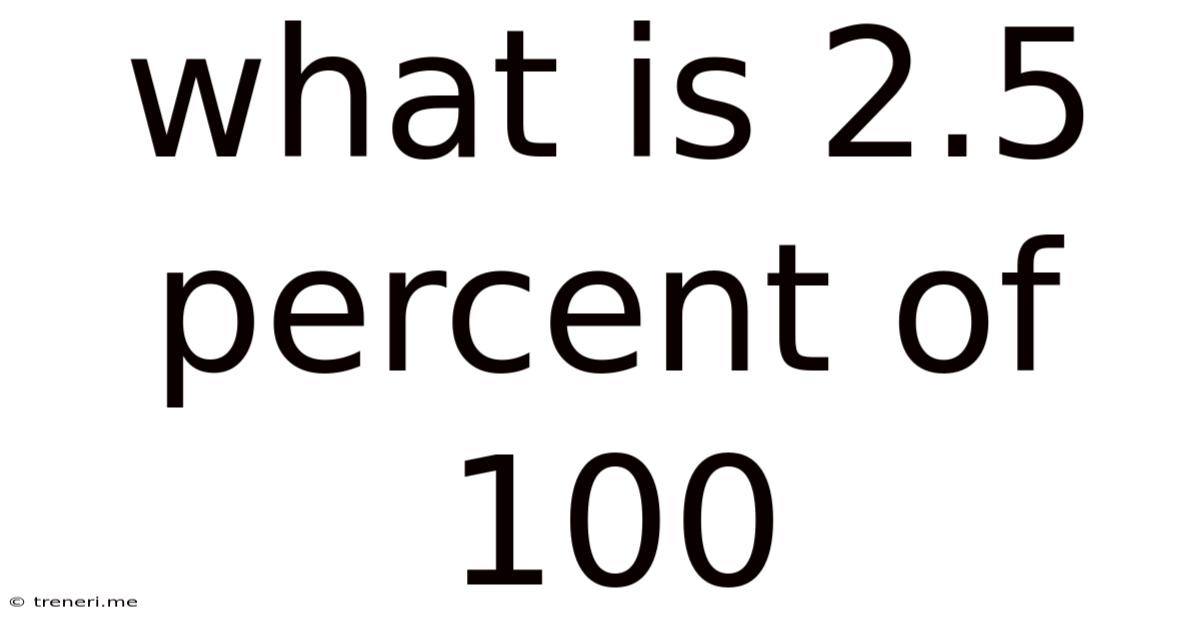
Table of Contents
What is 2.5 Percent of 100? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill in many areas of life, from everyday budgeting and shopping to complex financial analyses and scientific research. Understanding how to calculate percentages allows you to make informed decisions, compare values effectively, and grasp the significance of proportional changes. This article will thoroughly explore the seemingly simple question: "What is 2.5 percent of 100?" We'll move beyond the basic calculation to delve into the broader context of percentages, their applications, and the importance of mastering this mathematical concept.
Understanding Percentages: The Basics
Before we tackle the specific calculation, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Therefore, 2.5 percent can be represented in several ways:
- As a fraction: 2.5/100 or 1/40 (simplified)
- As a decimal: 0.025
- As a ratio: 2.5:100 or 1:40 (simplified)
This flexibility in representation is crucial for understanding and applying percentages in diverse contexts.
Calculating 2.5 Percent of 100
Now, let's address the core question: What is 2.5 percent of 100? There are several ways to approach this calculation:
Method 1: Using the Decimal Equivalent
The simplest method involves converting the percentage to its decimal equivalent and multiplying it by the whole number.
- Convert the percentage to a decimal: 2.5% = 0.025
- Multiply the decimal by the whole number: 0.025 * 100 = 2.5
Therefore, 2.5 percent of 100 is 2.5.
Method 2: Using the Fraction Equivalent
We can also use the fraction equivalent of 2.5%.
- Convert the percentage to a fraction: 2.5% = 2.5/100 = 1/40
- Multiply the fraction by the whole number: (1/40) * 100 = 100/40 = 2.5
Again, we arrive at the answer: 2.5 percent of 100 is 2.5.
Method 3: Using Proportions
This method is particularly useful for understanding the underlying relationships involved. We can set up a proportion:
- Let 'x' represent 2.5% of 100.
- We can write the proportion as: x/100 = 2.5/100
- To solve for 'x', we can cross-multiply: 100x = 2.5 * 100
- Dividing both sides by 100, we get: x = 2.5
This confirms that 2.5 percent of 100 is 2.5.
Practical Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous real-world situations. Here are a few examples:
1. Finance and Budgeting:
- Interest calculations: Banks and financial institutions use percentages to calculate interest on loans, savings accounts, and investments. Understanding percentage calculations is crucial for making informed decisions about financial products.
- Discounts and sales tax: Stores use percentages to advertise discounts and calculate sales tax. Being able to quickly calculate these values is essential for savvy shopping.
- Investment returns: Investors use percentage calculations to track their investment returns and assess the performance of their portfolios.
2. Science and Statistics:
- Data analysis: Percentages are frequently used to represent proportions and changes in data. For example, scientists use percentages to express the concentration of a substance in a solution or the percentage increase or decrease in a population.
- Statistical inference: Percentages play a key role in statistical analysis, enabling researchers to draw inferences from data and make predictions.
3. Everyday Life:
- Tips and gratuities: Calculating tips in restaurants often involves determining a percentage of the total bill.
- Grading and assessments: Schools and universities use percentages to represent students' scores on tests and assignments.
- Sales commissions: Salespeople often earn commissions based on a percentage of their sales.
Beyond the Basics: More Complex Percentage Calculations
While calculating 2.5% of 100 is relatively straightforward, many real-world applications involve more complex scenarios. Here are some examples:
- Calculating percentage increase or decrease: This involves finding the difference between two values, dividing by the original value, and multiplying by 100. For instance, if a price increases from $100 to $105, the percentage increase is [(105-100)/100] * 100 = 5%.
- Finding the original value given a percentage and a result: For example, if a discounted price is $75 and the discount is 25%, you can find the original price by setting up an equation: 0.75x = 75, where 'x' represents the original price. Solving for x gives x = $100.
- Compound interest: This involves calculating interest on both the principal amount and accumulated interest. Compound interest calculations are more complex but crucial for understanding long-term investments.
Mastering Percentages: Tips and Strategies
To effectively master percentage calculations, consider the following:
- Practice regularly: The more you practice, the more comfortable and efficient you'll become. Start with simple calculations and gradually progress to more complex ones.
- Use different methods: Experiment with various calculation methods (decimal, fraction, proportion) to find the approach that best suits your understanding and problem-solving style.
- Understand the underlying concepts: Don't just memorize formulas; strive to understand the underlying principles of percentages and their applications.
- Utilize online resources: Numerous online resources, including calculators and tutorials, can help you refine your skills and tackle challenging problems.
- Relate percentages to real-world scenarios: Try applying percentage calculations to real-life situations to enhance your understanding and retention.
Conclusion: The Significance of Percentage Calculations
The simple question "What is 2.5 percent of 100?" serves as a springboard for exploring the vast world of percentage calculations and their far-reaching applications. Mastering this fundamental mathematical concept is essential for navigating various aspects of personal finance, professional endeavors, and everyday life. By understanding the different methods of calculating percentages and practicing regularly, you can build a strong foundation for confidently tackling more complex problems and making informed decisions in various contexts. The ability to efficiently and accurately calculate percentages is a valuable asset in today's world, contributing to both personal and professional success.
Latest Posts
Latest Posts
-
Common Factors Of 14 And 42
May 11, 2025
-
What Is The Distance Between Points M And N
May 11, 2025
-
What Grade Is 92 Out Of 100
May 11, 2025
-
How Many Days Is June 1
May 11, 2025
-
How Many Minutes Is 75 Minutes
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 2.5 Percent Of 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.