What Is 2 To The 4 Power
Treneri
May 14, 2025 · 5 min read
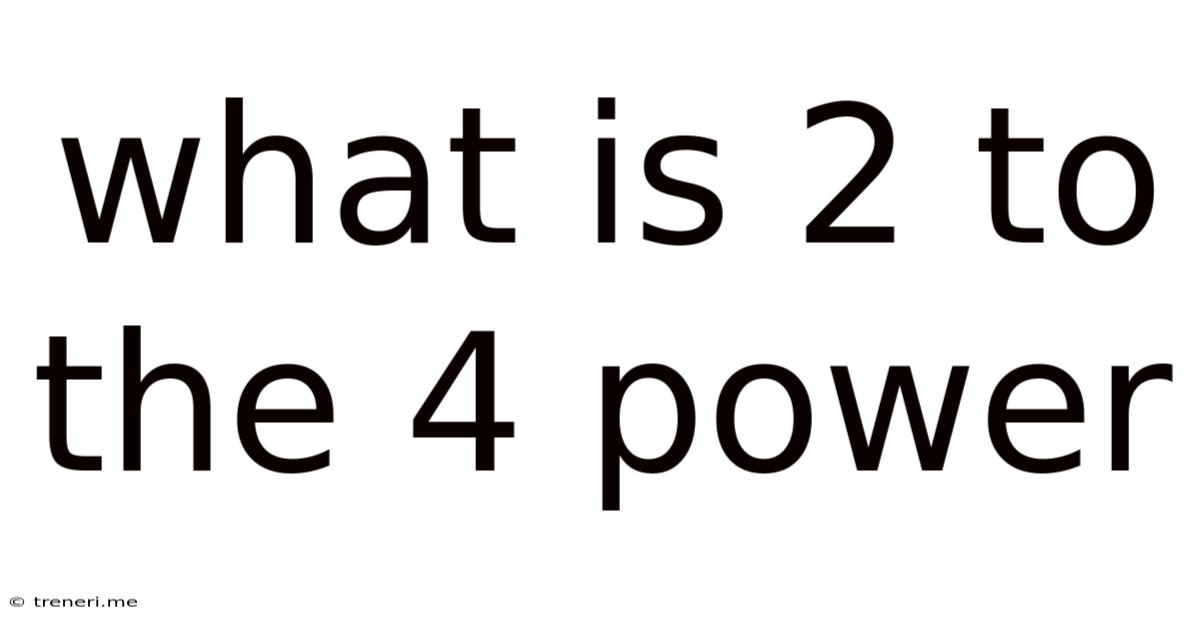
Table of Contents
What is 2 to the 4th Power? A Comprehensive Exploration of Exponents and Their Applications
Understanding exponents is fundamental to mathematics and numerous scientific fields. This article delves deep into the meaning of "2 to the 4th power," explaining not only the calculation but also the underlying concepts, various applications, and related mathematical principles. We'll explore different ways to approach this problem, ensuring a comprehensive understanding for readers of all mathematical backgrounds.
Understanding Exponents: The Basics
Before tackling "2 to the 4th power," let's establish a solid foundation in exponential notation. An exponent, also known as a power or index, indicates how many times a number (the base) is multiplied by itself. It's represented as a superscript number to the right of the base.
For instance, in the expression 2<sup>4</sup>, '2' is the base, and '4' is the exponent. This means 2 is multiplied by itself 4 times. This can be written as:
2<sup>4</sup> = 2 × 2 × 2 × 2
Calculating 2 to the 4th Power
Now, let's calculate 2 to the 4th power:
2 × 2 = 4 4 × 2 = 8 8 × 2 = 16
Therefore, 2 to the 4th power equals 16. This simple calculation forms the basis for many more complex mathematical operations.
Beyond the Calculation: Understanding the Implications
While the calculation itself is straightforward, understanding the implications of exponents extends far beyond this single example. Exponents are crucial for representing:
-
Growth and Decay: Exponential functions are used to model phenomena that grow or decay at a rate proportional to their current size. This includes population growth, radioactive decay, compound interest, and the spread of diseases. Understanding 2<sup>4</sup> helps to grasp the rapid acceleration inherent in exponential growth.
-
Scientific Notation: In science, very large or very small numbers are often expressed using scientific notation, which relies on exponents to represent powers of 10. For example, the speed of light is approximately 3 x 10<sup>8</sup> meters per second.
-
Computer Science: Binary numbers, the foundation of computer systems, utilize powers of 2. Understanding exponents is essential for comprehending how computers store and process information. The number 16, the result of 2<sup>4</sup>, is a common value in computer memory and data structures.
-
Geometry and Algebra: Exponents are frequently used in geometric calculations, such as calculating the volume of cubes (side<sup>3</sup>) or the area of squares (side<sup>2</sup>). They also appear prominently in algebraic equations and formulas.
-
Probability and Statistics: Calculating probabilities of events often involves exponents. For example, the probability of getting heads four times in a row when flipping a fair coin is (1/2)<sup>4</sup> = 1/16.
Different Ways to Represent 2<sup>4</sup>
While 2<sup>4</sup> = 16 is the direct calculation, it's helpful to understand other ways to express this relationship:
-
Repeated Multiplication: As discussed earlier, this is the most fundamental approach: 2 × 2 × 2 × 2 = 16.
-
Using a Calculator: Scientific calculators have an exponent function (usually denoted as x<sup>y</sup> or ^) which simplifies the calculation. Simply input 2, then the exponent function, then 4, and the calculator will display 16.
-
Logarithms: Logarithms are the inverse of exponents. If 2<sup>4</sup> = 16, then the logarithm base 2 of 16 is 4 (log<sub>2</sub>16 = 4). This shows the relationship between exponents and logarithms.
-
Binary Representation: In binary (base-2) number system, 16 is represented as 10000, illustrating the direct link between powers of 2 and binary notation.
Expanding the Concept: Larger Exponents and Negative Exponents
The principle of exponents extends far beyond 2<sup>4</sup>. Let's explore some related concepts:
-
Larger Exponents: Calculating 2<sup>5</sup>, 2<sup>10</sup>, or even larger exponents follows the same principle of repeated multiplication. The result increases rapidly as the exponent grows, demonstrating the power of exponential growth.
-
Negative Exponents: A negative exponent indicates the reciprocal of the base raised to the positive exponent. For example:
2<sup>-4</sup> = 1/2<sup>4</sup> = 1/16
This concept is vital in various scientific and engineering applications, including electrical engineering and physics.
-
Fractional Exponents: Fractional exponents represent roots. For example:
2<sup>1/2</sup> = √2 (the square root of 2) 2<sup>1/3</sup> = ∛2 (the cube root of 2)
Fractional exponents introduce the concept of radicals and their relationship to exponents.
Real-World Applications: Examples
Let's consider some practical examples where understanding 2<sup>4</sup> = 16 proves useful:
-
Data Storage: A computer memory unit with 4 bits can store 2<sup>4</sup> = 16 different values. This concept extends to larger memory units like kilobytes, megabytes, and gigabytes, which are all based on powers of 2.
-
Compound Interest: If you invest $1000 at an annual interest rate of 100% compounded annually (an unrealistic but illustrative scenario), after 4 years, your investment will be 1000 × 2<sup>4</sup> = $16,000. This showcases the significant effect of compounding over time.
-
Chessboard Problem: The classic chessboard problem illustrates exponential growth. If you were to place one grain of rice on the first square of a chessboard, two on the second, four on the third, and so on, doubling the number of grains on each subsequent square, the total number of grains would be an enormous 2<sup>64</sup> -1 . This demonstrates the rapid growth potential of exponential sequences.
-
Game Theory: In many games of strategy or chance, understanding exponential growth can provide a competitive advantage. For instance, in some scenarios, a small initial advantage can escalate exponentially, leading to a significant difference in the final outcome.
Conclusion: The Significance of 2<sup>4</sup>
While the calculation of 2<sup>4</sup> = 16 may seem simple, its underlying concepts and applications are vast and significant. Understanding exponents is crucial for comprehending exponential growth, scientific notation, computer science principles, and various mathematical applications across numerous fields. Mastering this fundamental concept paves the way for tackling more complex mathematical problems and unlocks a deeper understanding of the world around us. The simple equation, 2<sup>4</sup> = 16, is a gateway to a broader world of mathematical possibilities.
Latest Posts
Latest Posts
-
How Many More Hours Until 5 Am
May 15, 2025
-
How Many Days Left Of September
May 15, 2025
-
How To Find Board Feet In A Log
May 15, 2025
-
22 Out Of 30 As A Percentage Grade
May 15, 2025
-
Convert 3 4 Cup To Ounces
May 15, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 To The 4 Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.