What Is 25 Percent Of 4000
Treneri
May 10, 2025 · 4 min read
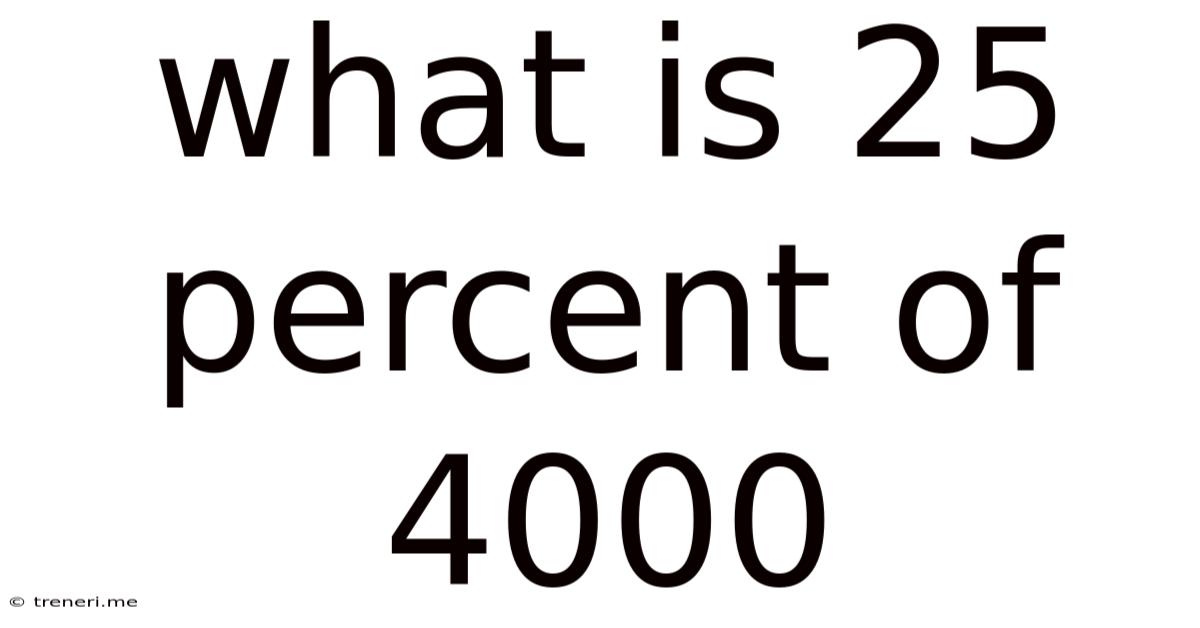
Table of Contents
What is 25 Percent of 4000? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with wide-ranging applications in various aspects of life, from everyday budgeting and shopping to complex financial analysis and scientific research. This article delves into the question, "What is 25 percent of 4000?", providing not only the answer but also a detailed explanation of the methods and concepts involved. We'll explore different approaches to percentage calculations, highlight practical examples, and equip you with the knowledge to tackle similar problems with confidence.
Understanding Percentages
Before diving into the specific calculation, let's establish a clear understanding of what percentages represent. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 25% can be expressed as the fraction 25/100 or the decimal 0.25.
Understanding this fundamental concept is crucial for grasping percentage calculations. Every percentage problem essentially involves finding a portion of a whole. In our case, we're looking for 25% of 4000, meaning we need to find 25 out of every 100 parts of 4000.
Method 1: Using the Decimal Equivalent
This is arguably the most straightforward method. We convert the percentage to its decimal equivalent and then multiply it by the whole number.
-
Step 1: Convert the percentage to a decimal. To do this, divide the percentage by 100. So, 25% becomes 25/100 = 0.25
-
Step 2: Multiply the decimal by the whole number. In this case, multiply 0.25 by 4000: 0.25 * 4000 = 1000
Therefore, 25% of 4000 is 1000.
Method 2: Using the Fraction Equivalent
Percentages can also be expressed as fractions. This method offers a different perspective and can be helpful in visualizing the problem.
-
Step 1: Convert the percentage to a fraction. 25% is equivalent to the fraction 25/100. This fraction can be simplified by dividing both the numerator and the denominator by their greatest common divisor (25): 25/100 simplifies to 1/4.
-
Step 2: Multiply the fraction by the whole number. Multiply 1/4 by 4000: (1/4) * 4000 = 4000/4 = 1000
Again, we arrive at the answer: 25% of 4000 is 1000.
Method 3: Proportion Method
This method utilizes the concept of proportions, setting up an equation to solve for the unknown value.
-
Step 1: Set up a proportion. We can represent the problem as a proportion: 25/100 = x/4000, where 'x' represents the unknown value (25% of 4000).
-
Step 2: Cross-multiply. Cross-multiplying gives us: 100x = 25 * 4000 = 100000
-
Step 3: Solve for x. Divide both sides of the equation by 100: x = 100000/100 = 1000
This method also confirms that 25% of 4000 is 1000.
Real-World Applications: Why is This Important?
Understanding percentage calculations is vital in many real-world situations:
-
Financial planning: Calculating discounts, interest rates, taxes, and investment returns all rely heavily on percentage calculations. For example, if you're buying a $4000 item with a 25% discount, you'll save $1000.
-
Sales and marketing: Determining profit margins, analyzing sales data, and calculating commission all involve working with percentages. Understanding percentage changes is crucial for tracking business performance.
-
Everyday budgeting: Tracking your spending, allocating funds for different expenses, and calculating savings rates often require percentage calculations.
-
Scientific research and data analysis: Percentages are used extensively in representing data, calculating statistical probabilities, and expressing changes in experimental results.
Advanced Percentage Calculations: Beyond the Basics
While the above methods cover the basics, let's explore more complex scenarios:
-
Calculating Percentage Increase/Decrease: If a value increases or decreases by a certain percentage, you can calculate the new value using the following formulas:
- Increase: New Value = Original Value * (1 + Percentage Increase)
- Decrease: New Value = Original Value * (1 - Percentage Decrease)
-
Finding the Percentage of a Number: If you know the part and the whole, you can calculate the percentage using the formula: Percentage = (Part/Whole) * 100
-
Finding the Original Value: If you know the percentage and the resulting value after a percentage increase or decrease, you can work backward to find the original value using algebraic manipulation.
Practice Problems
To solidify your understanding, try solving these problems:
- What is 15% of 6000?
- What is 75% of 200?
- A shirt originally costs $50 is on sale for 30% off. What is the sale price?
- A store's revenue increased from $10,000 to $12,000. What is the percentage increase?
Conclusion
Calculating percentages, even seemingly simple ones like finding 25% of 4000, is a fundamental skill with widespread practical applications. Mastering different calculation methods, as demonstrated above, will enable you to tackle various percentage problems with confidence and accuracy. Remember, consistent practice is key to improving your proficiency. Understanding percentages will empower you to make informed decisions in various aspects of your personal and professional life. This knowledge is invaluable in navigating the complexities of finances, sales, data analysis, and countless other areas where percentage calculations are essential.
Latest Posts
Latest Posts
-
0 6 To The Power Of 3
May 10, 2025
-
What Is The Greatest Common Factor Of 14 And 16
May 10, 2025
-
180 Days From February 9 2024
May 10, 2025
-
How Many Days Since January 21
May 10, 2025
-
16 Out Of 20 As A Percentage Grade
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is 25 Percent Of 4000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.