What Is 30 Percent Of 2400
Treneri
May 12, 2025 · 5 min read
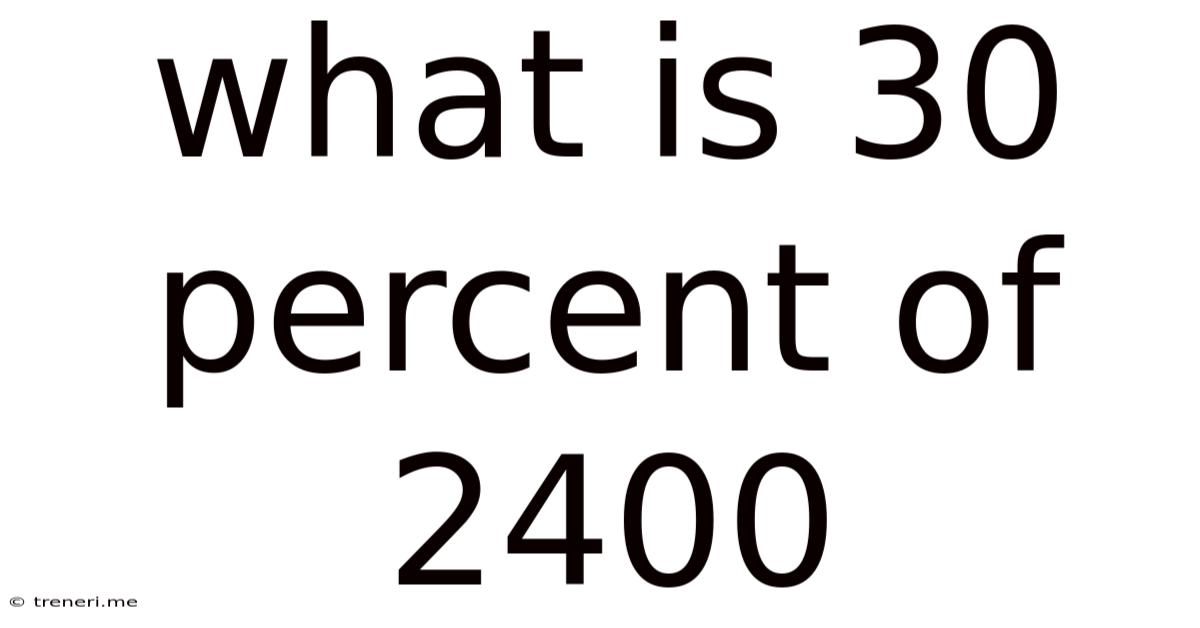
Table of Contents
What is 30 Percent of 2400? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from everyday budgeting and shopping to complex financial analysis and scientific research. Understanding how to determine a percentage of a number is crucial for making informed decisions and accurately interpreting data. This article delves into the calculation of "What is 30 percent of 2400?", providing a detailed explanation of the process, exploring different calculation methods, and offering practical examples to solidify your understanding. We'll also touch upon the broader significance of percentage calculations and how they impact various fields.
Understanding Percentages
Before we dive into the calculation, let's clarify the concept of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For example, 30% means 30 out of 100, or 30/100, which simplifies to 3/10 as a fraction and 0.3 as a decimal.
Method 1: Using the Decimal Method
This is arguably the most straightforward and commonly used method for calculating percentages. It involves converting the percentage into a decimal and then multiplying it by the given number.
Steps:
-
Convert the percentage to a decimal: To convert 30% to a decimal, divide it by 100: 30% / 100 = 0.30
-
Multiply the decimal by the number: Multiply the decimal (0.30) by the number you want to find the percentage of (2400): 0.30 * 2400 = 720
Therefore, 30% of 2400 is 720.
This method is efficient and easily adaptable for various percentage calculations. It's particularly useful when working with calculators or spreadsheets.
Method 2: Using the Fraction Method
This method involves converting the percentage into a fraction and then multiplying it by the given number.
Steps:
-
Convert the percentage to a fraction: 30% can be written as the fraction 30/100.
-
Simplify the fraction (if possible): The fraction 30/100 can be simplified by dividing both the numerator and denominator by their greatest common divisor (GCD), which is 10: 30/100 = 3/10
-
Multiply the fraction by the number: Multiply the simplified fraction (3/10) by the number (2400): (3/10) * 2400 = 720
Therefore, 30% of 2400 is 720.
This method enhances understanding of the underlying fractional representation of percentages. While it might take slightly longer than the decimal method, it's valuable for developing a stronger grasp of mathematical concepts.
Method 3: Using Proportions
This method involves setting up a proportion to solve for the unknown value.
Steps:
-
Set up a proportion: We can set up a proportion: x/2400 = 30/100, where 'x' represents the unknown value (30% of 2400).
-
Cross-multiply: Cross-multiply the terms: 100x = 30 * 2400
-
Solve for x: Solve for 'x' by dividing both sides by 100: x = (30 * 2400) / 100 = 720
Therefore, 30% of 2400 is 720.
The proportion method provides a structured approach to solving percentage problems, especially helpful when dealing with more complex scenarios.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in various real-world situations:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For example, understanding the interest rate on a loan or the discount percentage on a sale is crucial for making financial decisions.
-
Retail and Sales: Sales tax calculations, discounts on merchandise, and calculating markups on products all involve percentages. Understanding these calculations is important for both consumers and retailers.
-
Statistics and Data Analysis: Percentages are widely used to represent proportions and trends in data. Researchers use percentages to summarize findings, compare groups, and identify patterns. For example, understanding the percentage of a population that supports a particular candidate is critical in political analysis.
-
Science and Engineering: Percentage calculations are essential in various scientific and engineering disciplines. Determining the percentage of a particular component in a mixture, calculating efficiency ratios, and expressing experimental results often involve percentages.
-
Everyday Life: From calculating tips at restaurants to understanding the nutritional information on food labels, percentages are embedded in our daily routines.
Beyond the Basics: Advanced Percentage Calculations
While calculating 30% of 2400 is straightforward, the principles can be extended to more complex scenarios:
-
Calculating percentage increase or decrease: Suppose a quantity increases from 2000 to 2400. To determine the percentage increase, calculate the difference (400), divide it by the original value (2000), and multiply by 100: (400/2000) * 100 = 20%. Percentage decrease is calculated similarly.
-
Finding the original value: If you know the percentage and the resulting value, you can work backward to find the original value. For example, if 720 represents 30% of a number, you would divide 720 by 0.30 to find the original number: 720 / 0.30 = 2400.
-
Calculating multiple percentages: You might need to calculate successive percentages, such as applying a discount and then adding sales tax. Each percentage calculation is done sequentially.
-
Using percentage formulas in spreadsheets: Software like Microsoft Excel or Google Sheets provides built-in functions for percentage calculations, making complex calculations easier and more efficient.
Conclusion
Understanding how to calculate percentages is a vital skill for navigating the complexities of the modern world. Whether you are managing your personal finances, analyzing data, or tackling scientific problems, a thorough understanding of percentage calculations will empower you to make informed decisions and interpret information accurately. The simple calculation of "What is 30 percent of 2400?" serves as a gateway to a broader understanding of percentages and their widespread applications in various fields. By mastering the different methods explained above, you can confidently tackle a wide range of percentage-related problems and unlock a deeper understanding of numerical relationships. Remember, practice is key to mastering any mathematical concept, so don't hesitate to work through additional examples and apply these techniques to your own real-world situations.
Latest Posts
Latest Posts
-
What Fraction Is Equivalent To 2 6
May 12, 2025
-
10 Cups Equals How Many Ounces
May 12, 2025
-
Cuanto Es 1000 Cm En Metros
May 12, 2025
-
Can You Tan In Uv Index 6
May 12, 2025
-
How Many Milligrams Are In A Drop
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 30 Percent Of 2400 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.