What Is 4 Percent Of 40
Treneri
Apr 12, 2025 · 4 min read
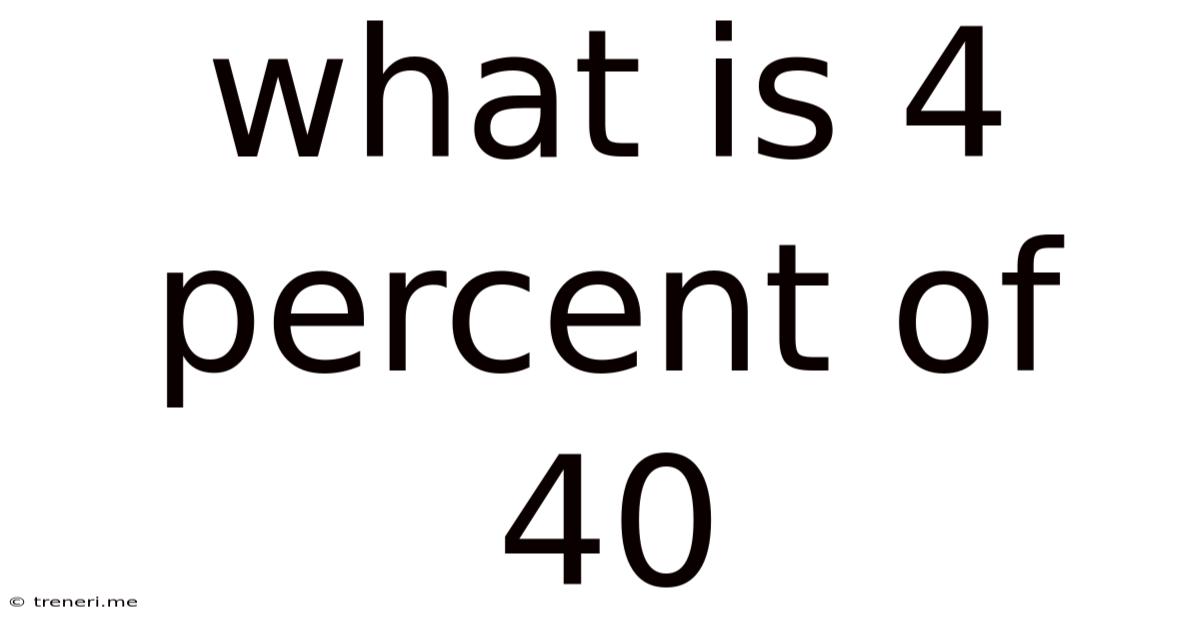
Table of Contents
What is 4 Percent of 40? A Deep Dive into Percentage Calculations
This seemingly simple question, "What is 4 percent of 40?", opens the door to a fascinating exploration of percentage calculations, their real-world applications, and the underlying mathematical principles. While the answer itself is easily obtained, understanding the how and why behind the calculation is crucial for anyone navigating the numerical world, from everyday budgeting to complex financial analyses.
Understanding Percentages: The Foundation
Before we tackle the specific problem, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent" or "out of 100". Therefore, 4% can be written as:
- 4/100 (as a fraction)
- 0.04 (as a decimal)
This decimal representation is key to performing percentage calculations efficiently.
Calculating 4 Percent of 40: The Three Main Methods
There are several ways to calculate 4 percent of 40. Let's explore three common methods:
Method 1: Using the Decimal Equivalent
This is arguably the most straightforward method. We convert the percentage to its decimal equivalent (0.04) and multiply it by the number we're finding the percentage of (40).
Calculation: 0.04 * 40 = 1.6
Therefore, 4 percent of 40 is 1.6.
Method 2: Using the Fraction Equivalent
We can also use the fraction equivalent of 4%, which is 4/100. The calculation becomes:
Calculation: (4/100) * 40 = 160/100 = 1.6
This method highlights the fundamental relationship between fractions and percentages. Simplifying the fraction before multiplication can sometimes make the calculation easier.
Method 3: Proportion Method (Cross-Multiplication)
This method is particularly useful for solving more complex percentage problems. We set up a proportion:
- x / 40 = 4 / 100
Where 'x' represents the unknown value (4 percent of 40). Cross-multiplying gives us:
- 100x = 4 * 40
- 100x = 160
- x = 160 / 100
- x = 1.6
Again, we arrive at the answer: 1.6.
Real-World Applications: Where Percentage Calculations Matter
Understanding percentage calculations isn't just an academic exercise; it's a vital skill with numerous real-world applications:
1. Finance and Budgeting:
- Interest Calculations: Calculating simple or compound interest on loans, savings accounts, or investments heavily relies on percentage calculations. Understanding how interest rates affect your financial situation is crucial for responsible money management. For instance, if you invest $40 and it earns 4% interest, you'll earn $1.60.
- Discounts and Sales Tax: Determining the final price of an item after a discount or adding sales tax requires accurate percentage calculations. A 4% discount on a $40 item, for example, would save you $1.60.
- Investment Returns: Tracking the performance of investments often involves calculating percentage changes in value. A 4% increase in the value of a $40 investment means a $1.60 gain.
- Tip Calculations: Calculating a tip in a restaurant or any service industry often involves using percentages.
2. Data Analysis and Statistics:
- Analyzing survey results: Percentages are frequently used to represent data in surveys, polls, and market research. Understanding these percentages helps interpret the overall findings.
- Calculating proportions: Percentages are essential for expressing proportions or ratios within a dataset. For instance, finding the percentage of a specific group within a larger population.
- Probability and Risk Assessment: Percentages are used to express probabilities and risks in various fields, including finance, insurance, and healthcare.
3. Everyday Life:
- Cooking and Baking: Recipes often require adjusting ingredient quantities based on percentages. For instance, increasing a recipe by 25% involves calculating percentages to find the right proportions.
- Shopping and Comparison: Comparing prices of similar products often involves calculating percentage differences to find the best value.
- Understanding Tax Rates: Understanding tax brackets and rates requires interpreting and working with percentages.
- Tracking Progress: Calculating percentage completion on projects helps track progress and manage timelines effectively.
Beyond the Basics: More Complex Percentage Problems
While "4 percent of 40" is a relatively simple problem, the underlying principles can be applied to more complex scenarios:
- Finding the original amount: If you know the percentage and the resulting value, you can work backward to find the original amount.
- Calculating percentage increase or decrease: Determining the percentage change between two values requires understanding how to calculate percentage differences.
- Working with multiple percentages: Some problems involve applying multiple percentages sequentially, such as calculating sales tax on a discounted item.
Mastering Percentage Calculations: Tips and Tricks
- Practice regularly: The more you practice percentage calculations, the more comfortable and efficient you'll become.
- Use different methods: Experiment with different calculation methods to find the one that works best for you.
- Break down complex problems: Divide complex problems into smaller, manageable steps.
- Check your work: Always double-check your calculations to avoid errors.
- Utilize online calculators: While it's essential to understand the underlying principles, online calculators can help verify your answers and save time on complex calculations.
Conclusion: The Power of Percentage Understanding
The seemingly simple question, "What is 4 percent of 40?", highlights the fundamental importance of understanding percentage calculations. This skill is not just useful for solving mathematical problems but is crucial for navigating various aspects of daily life, professional settings, and financial decision-making. By mastering these calculations and understanding their applications, you equip yourself with a valuable tool for making informed decisions and solving real-world problems. The answer, 1.6, is only a small part of the much larger and more significant understanding of percentages.
Latest Posts
Latest Posts
-
40 Kg In Stones And Pounds
May 09, 2025
-
How Many Days Is 278 Hours
May 09, 2025
-
What Is The Greatest Common Factor Of 18 And 15
May 09, 2025
-
Calcular Galones De Agua En Piscina
May 09, 2025
-
Cuanto Falta Para El 21 De Septiembre 2024
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 4 Percent Of 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.