What Is 50 Percent Of 12
Treneri
May 14, 2025 · 5 min read
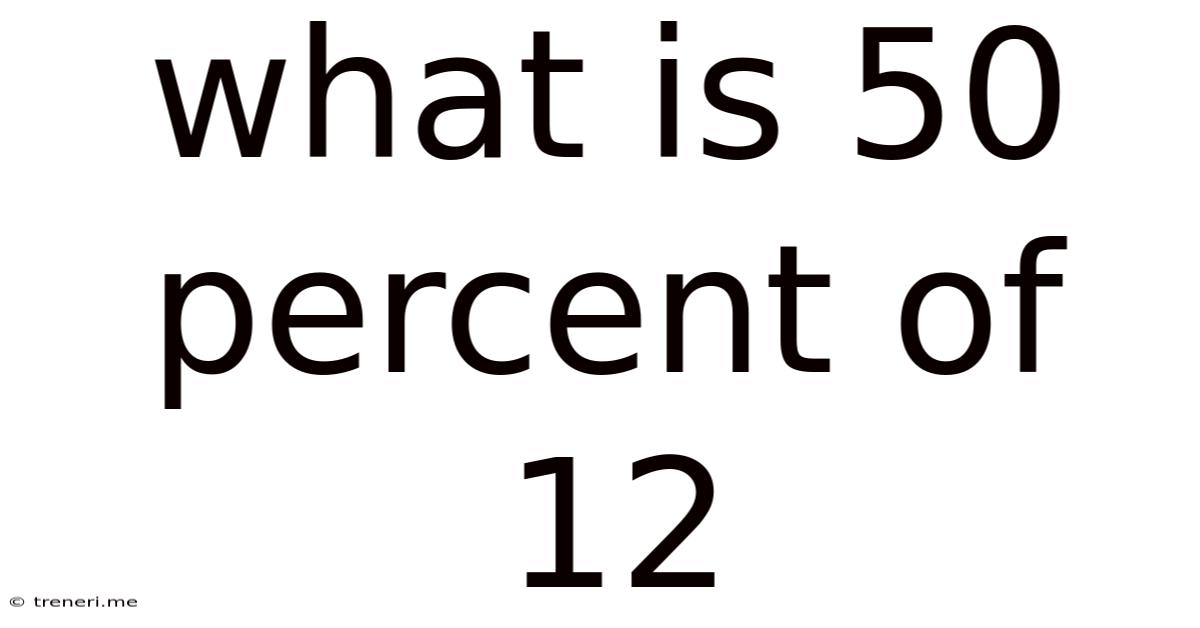
Table of Contents
What is 50 Percent of 12? A Deep Dive into Percentages and Their Applications
Finding 50% of 12 might seem like a simple calculation, and it is – the answer is 6. However, understanding the underlying concepts of percentages and how to apply them in various situations is far more profound. This article will explore the meaning of percentages, different methods for calculating them, and numerous real-world examples where this seemingly basic calculation becomes incredibly useful. We'll delve into the math, explore practical applications, and even touch upon the history of percentages to give you a comprehensive understanding.
Understanding Percentages: The Basics
A percentage is simply a fraction expressed as a part of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 50% means 50 out of 100, which can be written as the fraction 50/100 or the decimal 0.5. This foundational understanding is key to solving any percentage problem.
The Three Key Players: Percentage, Whole, and Part
To understand percentage calculations, you need to identify three key elements:
- The Percentage: This is the rate or proportion, usually expressed with the "%" symbol (e.g., 50%).
- The Whole: This is the total amount or the original number you're working with (e.g., 12 in our case).
- The Part: This is the portion of the whole that represents the percentage (e.g., the result of 50% of 12, which is 6).
The relationship between these three elements can be expressed in a simple formula:
(Percentage/100) * Whole = Part
In our example: (50/100) * 12 = 6
Calculating 50% of 12: Different Approaches
While the answer is straightforward, let's examine several ways to calculate 50% of 12, highlighting different mathematical approaches:
Method 1: Using the Formula
This is the most direct approach, using the formula mentioned above:
(50/100) * 12 = 0.5 * 12 = 6
This method is reliable and easy to understand, making it suitable for various percentage calculations.
Method 2: Fraction Conversion
Since 50% is equivalent to the fraction 1/2, we can simplify the calculation:
(1/2) * 12 = 12/2 = 6
This method emphasizes the fractional representation of percentages, offering a quick and intuitive way to find the solution.
Method 3: Mental Math
For 50%, a simple mental shortcut is to divide the whole number by 2. Half of 12 is 6. This method is efficient for quick calculations involving 50% or other easily recognizable fractions.
Real-World Applications: Beyond the Classroom
Understanding percentages extends far beyond simple mathematical exercises. Here are some real-world scenarios where calculating 50% (or other percentages) is crucial:
1. Sales and Discounts
Retail stores frequently advertise discounts as percentages. If a $24 item is on sale for 50% off, you'd calculate 50% of $24 (which is $12) and subtract that amount from the original price to find the sale price ($24 - $12 = $12).
2. Finance and Investments
Percentages are fundamental in finance. Calculating interest rates, returns on investments, and changes in stock prices all rely on percentage calculations. A 50% increase in investment value means your investment doubled.
3. Statistics and Data Analysis
Percentages are essential for interpreting statistical data. For example, understanding voter turnout (expressed as a percentage), analyzing survey results, or representing market share all involves percentage calculations.
4. Everyday Budgeting
Budgeting often involves expressing expenses as percentages of your income. Determining how much of your income is allocated to housing, food, transportation, or entertainment frequently uses percentages to visualize spending habits.
5. Science and Engineering
Scientific measurements and calculations often involve expressing data as percentages, for example, measuring the efficiency of a machine or analyzing the composition of a substance. A 50% efficiency indicates the machine is only producing half its potential output.
6. Cooking and Recipes
Recipes sometimes use percentages to represent the proportion of ingredients. A recipe calling for 50% flour by weight means half the total weight of the ingredients should be flour.
7. Taxes and Tax Rates
Tax rates are expressed as percentages. Understanding tax brackets and calculating tax liabilities involves applying percentages to taxable income.
8. Grading and Scores
Academic grading systems often use percentages to represent scores. A 50% score on an exam suggests that half of the questions were answered correctly.
9. Real Estate
Calculating commission percentages, property tax rates, and appreciation or depreciation of property values all use percentages as a fundamental part of the calculation.
10. Health and Nutrition
Nutrition labels express nutritional values as percentages of recommended daily intake. Understanding the percentage of daily value for vitamins, minerals, and macronutrients helps in making informed dietary choices.
Expanding Your Percentage Skills: Beyond 50%
While this article focused on 50% of 12, the principles and methods discussed can be applied to calculate any percentage of any number. Here's how to tackle more complex percentage problems:
- Using a Calculator: For more complex percentages, a calculator is incredibly helpful. Most calculators have a percentage function (%) that simplifies the calculation.
- Working with Decimals: Convert the percentage to a decimal (e.g., 25% = 0.25, 75% = 0.75) before multiplying it by the whole number.
- Solving for the Whole or Percentage: The formula can be rearranged to find the whole or the percentage if the other two elements are known.
Conclusion: The Power of Percentages
The simple calculation of 50% of 12 reveals a much broader understanding of percentages and their widespread applications. This seemingly basic concept forms the foundation of countless calculations in various fields, from everyday budgeting to complex financial analyses. Mastering percentage calculations equips you with a vital skill that enhances your ability to interpret data, make informed decisions, and confidently navigate the numerical aspects of life. Remember the core formula and the various methods discussed to apply your new-found knowledge across multiple domains. The ability to quickly and accurately calculate percentages is a valuable asset in personal and professional life.
Latest Posts
Latest Posts
-
5 3 X 7 3 Rug Size Chart
May 14, 2025
-
How Many Weeks In 60 Years
May 14, 2025
-
Can U Tan In Uv 1
May 14, 2025
-
How Many Gallons Is 52 Quarts
May 14, 2025
-
Find The Quotient Of 5 31 Divided By 15 23
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Is 50 Percent Of 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.