What Is 6 Percent Of 100
Treneri
May 14, 2025 · 5 min read
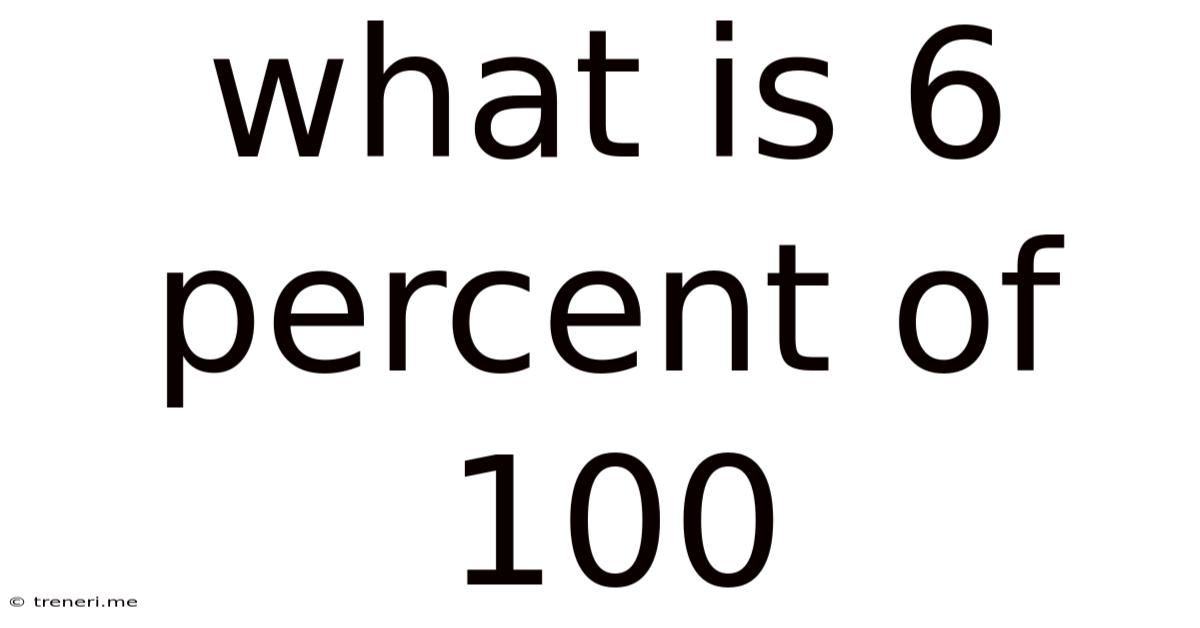
Table of Contents
What is 6 Percent of 100? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from personal finance to scientific research. Understanding how to determine a percentage of a number is crucial for making informed decisions, analyzing data, and comprehending information presented in percentage formats. This article will delve into the question, "What is 6 percent of 100?", providing a comprehensive explanation of the calculation, its practical applications, and related concepts.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" ("per cent" in Latin). Percentages are often denoted using the "%" symbol. For instance, 6% represents six parts out of one hundred. This can be written as a fraction (6/100) or a decimal (0.06).
Converting Percentages to Decimals and Fractions
To perform calculations involving percentages, it's often easier to convert them into their decimal or fractional equivalents. To convert a percentage to a decimal, simply divide the percentage by 100. For example, 6% as a decimal is 6 ÷ 100 = 0.06.
To convert a percentage to a fraction, write the percentage as the numerator and 100 as the denominator. Then, simplify the fraction if possible. In this case, 6% as a fraction is 6/100, which simplifies to 3/50.
Calculating 6 Percent of 100
Now, let's address the main question: What is 6 percent of 100?
There are three primary methods for calculating this:
Method 1: Using the Decimal Equivalent
- Convert the percentage to a decimal: 6% = 0.06
- Multiply the decimal by the number: 0.06 * 100 = 6
Therefore, 6 percent of 100 is 6.
Method 2: Using the Fraction Equivalent
- Convert the percentage to a fraction: 6% = 6/100 = 3/50
- Multiply the fraction by the number: (3/50) * 100 = 3 * (100/50) = 3 * 2 = 6
Again, 6 percent of 100 is 6.
Method 3: Using the Proportion Method
This method is particularly useful for understanding the underlying concept of percentages. We can set up a proportion:
- x/100 = 6/100 (where 'x' represents 6% of 100)
Solving for 'x':
- x = (6/100) * 100
- x = 6
This confirms that 6 percent of 100 is 6.
Practical Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous situations:
1. Finance and Budgeting:
- Calculating interest: Understanding interest rates (e.g., on loans or savings accounts) requires calculating percentages.
- Determining discounts: Sales often advertise discounts as percentages (e.g., a 20% discount on a $100 item).
- Analyzing investment returns: Investment growth is usually expressed as a percentage return on investment (ROI).
- Calculating taxes: Taxes are frequently levied as a percentage of income or the value of goods and services.
- Budgeting and Expense Tracking: Allocating a percentage of your income to different categories (housing, food, entertainment) helps in efficient budgeting.
2. Science and Research:
- Data analysis: Percentages are extensively used to represent proportions in data sets, such as the percentage of respondents who answered "yes" to a survey question.
- Statistical analysis: Many statistical measures, such as confidence intervals and error rates, are expressed as percentages.
- Scientific experiments: Percentages often represent the success rate or yield of an experiment.
3. Everyday Life:
- Tipping: Calculating a tip in a restaurant often involves determining a percentage of the bill amount (e.g., a 15% tip).
- Sales and Markups: Retailers use percentages to calculate markups on products to determine the selling price.
- Understanding nutritional information: Food labels often list the percentage of daily recommended values for various nutrients.
- Comparing prices: Percentages help to compare prices and value across different products or services.
4. Business and Economics:
- Profit margins: Businesses calculate profit margins as a percentage of revenue.
- Market share: Market share is often expressed as a percentage of the total market.
- Growth rates: Economic growth is frequently reported as a percentage increase or decrease.
- Inflation rates: Inflation measures the percentage increase in the price level of goods and services over time.
Beyond the Basics: More Complex Percentage Calculations
While calculating 6% of 100 is straightforward, many real-world situations involve more complex percentage calculations. Here are some examples:
1. Finding the Original Amount:
If you know the percentage and the resulting amount, you can work backward to find the original amount. For example, if a product costs $106 after a 6% sales tax, you can calculate the original price.
- Let 'x' be the original price.
- 1.06x = 106
- x = 106 / 1.06
- x = 100
The original price was $100.
2. Calculating Percentage Increase or Decrease:
Percentage changes represent the relative difference between two values. For example, if the price of an item increases from $100 to $106, the percentage increase is calculated as follows:
- Percentage Increase = [(New Value - Old Value) / Old Value] * 100
- Percentage Increase = [(106 - 100) / 100] * 100
- Percentage Increase = 6%
3. Calculating Percentage of a Percentage:
Sometimes you might need to calculate a percentage of a percentage. For example, you might need to find 20% of 6%. This is done by multiplying the two percentages (converted to decimals):
- 0.20 * 0.06 = 0.012 or 1.2%
Conclusion
Understanding how to calculate percentages is a crucial life skill with numerous applications across various fields. This article has explored the fundamental concept of percentages and provided different methods to calculate 6 percent of 100, highlighting its simplicity and practical significance. By mastering these concepts and applying them to different scenarios, you can make more informed decisions, analyze data more effectively, and navigate various aspects of life with greater confidence and understanding. The ability to calculate percentages effortlessly empowers you to make sense of numerical information and apply it effectively in various contexts. Remember that consistent practice and exposure to real-world applications are key to reinforcing your understanding and mastering this essential mathematical skill.
Latest Posts
Latest Posts
-
How Many Drapery Panels Do I Need
May 14, 2025
-
What Is The Gcf Of 54 And 24
May 14, 2025
-
How Long Would It Take To Travel 40 Light Years
May 14, 2025
-
Cuanto Es 1 Ano En Horas
May 14, 2025
-
How To Find Area Of A Triangle With Points
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Is 6 Percent Of 100 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.