What Is The Greatest Common Factor Of 72 And 45
Treneri
May 10, 2025 · 5 min read
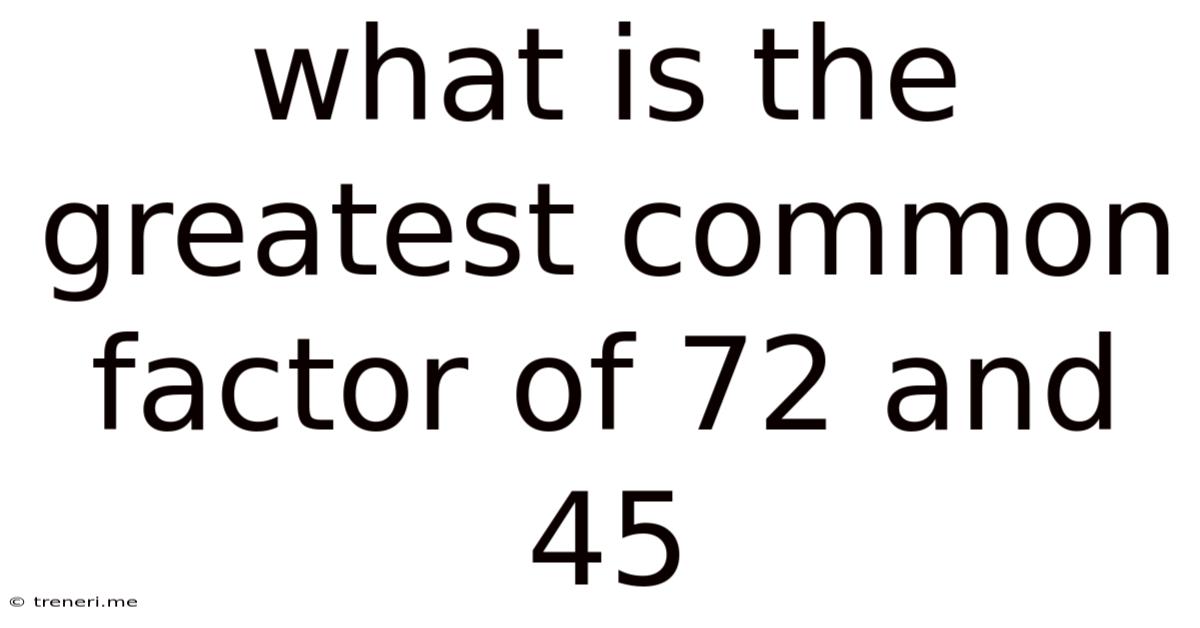
Table of Contents
What is the Greatest Common Factor of 72 and 45? A Deep Dive into Number Theory
Finding the greatest common factor (GCF) of two numbers might seem like a simple arithmetic task, but it's a fundamental concept in number theory with applications far beyond basic math. This article will delve into determining the GCF of 72 and 45, exploring multiple methods and demonstrating the underlying mathematical principles. We'll also touch upon the broader significance of GCFs in various fields.
Understanding Greatest Common Factors (GCF)
The greatest common factor, also known as the greatest common divisor (GCD), is the largest number that divides evenly into two or more numbers without leaving a remainder. It's a crucial concept in simplifying fractions, solving algebraic equations, and understanding relationships between numbers. For example, the GCF of 12 and 18 is 6 because 6 is the largest number that divides both 12 and 18 perfectly.
Method 1: Prime Factorization
This is arguably the most fundamental method for finding the GCF. It involves breaking down each number into its prime factors—numbers divisible only by 1 and themselves.
Step 1: Prime Factorization of 72
72 can be factored as follows:
72 = 2 x 36 = 2 x 2 x 18 = 2 x 2 x 2 x 9 = 2 x 2 x 2 x 3 x 3 = 2³ x 3²
Step 2: Prime Factorization of 45
45 can be factored as follows:
45 = 3 x 15 = 3 x 3 x 5 = 3² x 5
Step 3: Identifying Common Factors
Now, we compare the prime factorizations of 72 and 45:
72 = 2³ x 3² 45 = 3² x 5
The common factors are 3² (or 3 x 3 = 9).
Step 4: Calculating the GCF
The GCF is the product of the lowest powers of the common prime factors. In this case, the only common prime factor is 3, and the lowest power is 3². Therefore:
GCF(72, 45) = 3² = 9
Method 2: Euclidean Algorithm
The Euclidean algorithm is an efficient method, particularly useful for larger numbers. It's based on repeated application of the division algorithm.
Step 1: Divide the Larger Number by the Smaller Number
Divide 72 by 45:
72 ÷ 45 = 1 with a remainder of 27
Step 2: Replace the Larger Number with the Remainder
Now, we replace 72 with the remainder, 27:
45 ÷ 27 = 1 with a remainder of 18
Step 3: Repeat the Process
Repeat the process until the remainder is 0:
27 ÷ 18 = 1 with a remainder of 9
18 ÷ 9 = 2 with a remainder of 0
Step 4: The GCF is the Last Non-Zero Remainder
The last non-zero remainder is 9. Therefore:
GCF(72, 45) = 9
Method 3: Listing Factors
This method is straightforward but can be time-consuming for larger numbers.
Step 1: List the Factors of 72
The factors of 72 are: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72
Step 2: List the Factors of 45
The factors of 45 are: 1, 3, 5, 9, 15, 45
Step 3: Identify Common Factors
Compare the two lists and identify the common factors: 1, 3, 9
Step 4: Determine the Greatest Common Factor
The greatest of these common factors is 9. Therefore:
GCF(72, 45) = 9
Applications of GCF in Real-World Scenarios
The concept of GCF extends beyond classroom exercises; it has practical applications in various fields:
-
Simplifying Fractions: Finding the GCF allows us to simplify fractions to their lowest terms. For example, the fraction 72/45 can be simplified to 8/5 by dividing both the numerator and denominator by their GCF, 9.
-
Geometry and Measurement: GCF is crucial when dealing with problems involving area, perimeter, and volume, particularly when finding the largest square or cube that can be used to tile or fill a given space. Imagine trying to tile a rectangular floor with square tiles; finding the GCF of the floor's dimensions will determine the size of the largest square tile that can be used without any cutting.
-
Data Analysis and Number Theory: GCF plays a significant role in various advanced mathematical concepts like modular arithmetic, cryptography, and abstract algebra. Understanding GCFs helps solve complex problems related to divisibility and relationships between numbers.
-
Music Theory: In music theory, GCF is implicitly used when determining the greatest common divisor of two musical intervals. This helps understand the relationships between notes and build harmonious melodies.
Beyond the Basics: Extending the Concept
While we've focused on finding the GCF of two numbers, the concept can be extended to find the GCF of three or more numbers. The prime factorization method and the Euclidean algorithm can be adapted to handle multiple numbers. The prime factorization method requires finding the common prime factors across all numbers, and then taking the product of their lowest powers. For the Euclidean algorithm, you would iteratively apply the process to pairs of numbers until you obtain the GCF for all.
Conclusion: The Power of the GCF
The greatest common factor, seemingly a simple mathematical concept, underpins numerous applications across diverse fields. Understanding its calculation through different methods—prime factorization, the Euclidean algorithm, and listing factors—empowers us to solve practical problems and appreciate the intricate connections within number theory. From simplifying fractions to tackling complex geometrical problems, the GCF remains a powerful tool in the mathematical arsenal. Mastering the GCF not only enhances our understanding of numbers but also opens doors to more advanced mathematical concepts and real-world applications. The determination of the GCF(72, 45) = 9 serves as a fundamental example showcasing the utility and elegance of this core mathematical principle.
Latest Posts
Latest Posts
-
De 12 A 8 Cuantas Horas Son
May 10, 2025
-
51 Rounded To The Nearest Hundred
May 10, 2025
-
1 Cup Of Pasta In Grams
May 10, 2025
-
How Long Is 109 Minutes In Hours
May 10, 2025
-
How To Calculate Elastic Modulus From Stress Strain Curve
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Greatest Common Factor Of 72 And 45 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.