What Is The Percentage Of 30
Treneri
Apr 06, 2025 · 5 min read
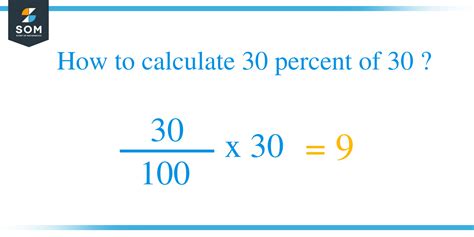
Table of Contents
What is the Percentage of 30? Understanding Percentages and Their Applications
The question "What is the percentage of 30?" is inherently incomplete. A percentage always represents a portion of a whole. To determine the percentage, we need to know what 30 represents relative to a larger quantity. This article will delve into understanding percentages, explore different scenarios where 30 might represent a percentage, and provide practical examples to solidify your understanding.
Understanding the Basics of Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, a percentage signifies how many parts out of 100 make up a given quantity. For example, 50% means 50 out of 100, or one-half.
Key Concepts:
- The Whole: This is the total amount or quantity you are considering.
- The Part: This is the portion of the whole that you're expressing as a percentage.
- The Percentage: This is the numerical representation (e.g., 30%, 75%, etc.) of the part relative to the whole.
The formula for calculating a percentage is:
(Part / Whole) x 100% = Percentage
Scenario 1: 30 as a Percentage of a Larger Number
Let's say you scored 30 out of 50 on a test. To find the percentage, you would use the formula:
(30 / 50) x 100% = 60%
Therefore, 30 represents 60% of 50.
Different Examples:
-
Scenario 2: Sales Targets: If your sales target was 100 units, and you sold 30 units, your achievement would be (30 / 100) x 100% = 30%.
-
Scenario 3: Survey Results: If 30 out of 100 respondents answered "yes" to a survey question, then 30% of respondents answered "yes."
-
Scenario 4: Discount: A 30% discount means that for every 100 units of currency, 30 units are deducted from the price. For a 100$ item, the discount would be 30$. If the item was 200$, the discount would be 60$ (30% of 200$).
-
Scenario 5: Population Percentage: Imagine a town with a population of 1000 people. If 30 of them are left-handed, then the percentage of left-handed individuals is (30 / 1000) x 100% = 3%.
These examples highlight how versatile percentages are. They are used in various fields, including:
- Finance: Calculating interest rates, returns on investment, profit margins, and discounts.
- Statistics: Representing data, analyzing trends, and making comparisons.
- Science: Expressing concentrations, experimental results, and error margins.
- Everyday Life: Understanding discounts, tips, taxes, and many other aspects of daily transactions.
Calculating the Whole When the Percentage and Part are Known
Sometimes, you know the percentage and the part, and you need to find the whole. For instance, if 30 represents 15% of a quantity, how do you find that quantity?
The formula is modified as:
Whole = (Part / Percentage) x 100
In this case:
Whole = (30 / 15) x 100 = 200
Therefore, 30 is 15% of 200.
Calculating the Part When the Percentage and Whole are Known
Conversely, if you know the percentage and the whole, you can easily calculate the part.
The formula remains:
Part = (Percentage / 100) x Whole
For example, if you want to find 30% of 500:
Part = (30 / 100) x 500 = 150
So, 150 is 30% of 500.
Percentage Increase and Decrease
Percentages are also essential for calculating percentage increases or decreases.
Percentage Increase:
To calculate the percentage increase, use the following formula:
[(New Value - Original Value) / Original Value] x 100%
Example: If the original value was 100 and the new value is 130, the percentage increase is:
[(130 - 100) / 100] x 100% = 30%
Percentage Decrease:
The formula for percentage decrease is similar:
[(Original Value - New Value) / Original Value] x 100%
Example: If the original value was 100 and the new value is 70, the percentage decrease is:
[(100 - 70) / 100] x 100% = 30%
Practical Applications and Real-World Examples
Percentages are everywhere in our daily lives. Here are some more examples demonstrating their diverse applications:
- Interest Rates: Banks and financial institutions use percentages to determine interest on loans and savings accounts. A 3% interest rate on a savings account means you earn 3 units of currency for every 100 units saved.
- Taxes: Governments use percentages to levy taxes on income, goods, and services. A 5% sales tax means you pay 5 units of currency for every 100 units spent.
- Inflation: The rate of inflation is expressed as a percentage, reflecting the increase in the general price level of goods and services.
- Investment Returns: Investment returns are often expressed as a percentage of the initial investment. A 10% return on a 1000$ investment means a profit of 100$.
- Health Statistics: Percentages are commonly used in healthcare to represent the prevalence of diseases, success rates of treatments, and other important health metrics.
- Academic Performance: Grades are frequently presented as percentages to indicate a student's performance in a particular course or subject.
Advanced Percentage Calculations
While the basic percentage calculations are relatively straightforward, some situations require more advanced techniques. These might involve:
- Compound Interest: Interest calculated not only on the principal but also on accumulated interest.
- Percentage Points: A difference in percentage values (e.g., a change from 10% to 13% is a 3-percentage-point increase, not a 30% increase).
- Weighted Averages: Calculating an average that takes into account the relative importance (weights) of different values.
Mastering these concepts allows for a deeper understanding and application of percentages across a broader range of scenarios.
Conclusion
The seemingly simple question, "What is the percentage of 30?" highlights the fundamental importance of understanding the context. A percentage is always relative to a whole. By grasping the basic formulas and their applications, you can effectively utilize percentages in various aspects of life, from financial calculations to analyzing data and interpreting information presented in percentages. This ability translates to better decision-making in numerous situations. Remember that the key to successfully working with percentages lies in clearly identifying the 'part' and the 'whole' within the problem. Once you establish these values, the calculation itself becomes straightforward and yields meaningful results.
Latest Posts
Latest Posts
-
How Many Miles Is 10 000 Km
Apr 07, 2025
-
How Many Calories Does The Elliptical Burn
Apr 07, 2025
-
1 Quart Of Milk In Cups
Apr 07, 2025
-
How Much Longer Until 10 Am
Apr 07, 2025
-
How Many Ounces Is 14 Pounds
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
