What Percent Is 8 Of 30
Treneri
May 14, 2025 · 4 min read
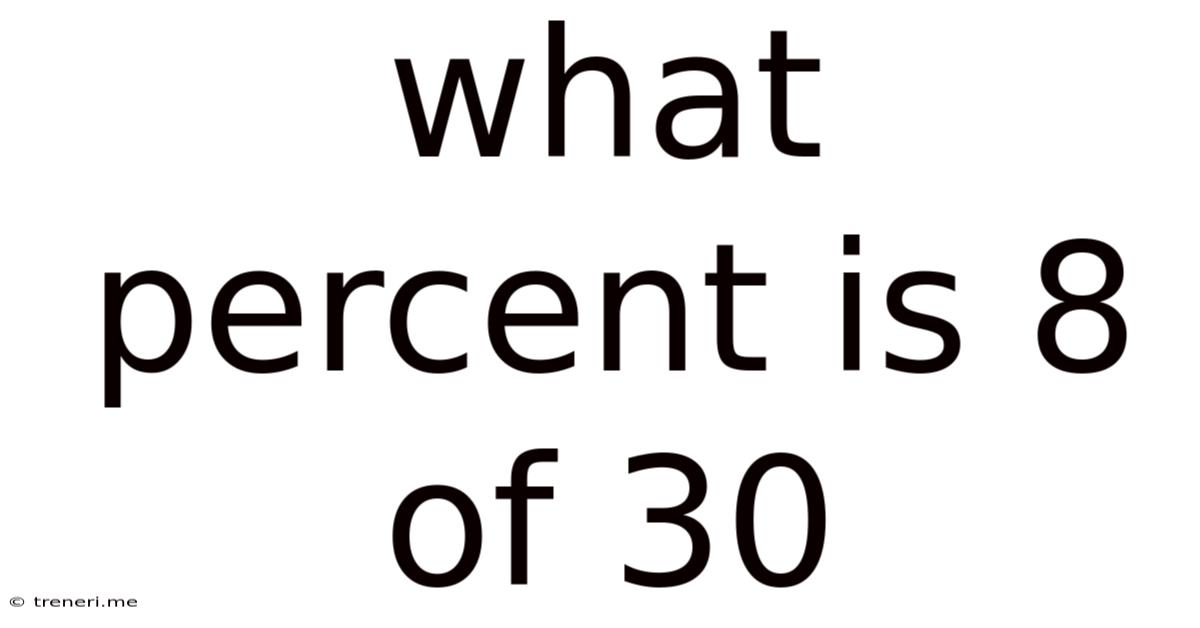
Table of Contents
What Percent is 8 of 30? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in numerous aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This article delves into the question, "What percent is 8 of 30?" We'll not only solve this specific problem but also equip you with the knowledge and tools to tackle any percentage calculation with confidence.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred." Therefore, 25% means 25 out of 100, which can also be written as the fraction 25/100 or the decimal 0.25. Understanding this basic concept is crucial for tackling percentage problems.
Calculating "What Percent is 8 of 30?"
There are several ways to calculate what percentage 8 represents of 30. Let's explore the most common methods:
Method 1: Using the Proportion Method
This method relies on setting up a proportion. We can represent the problem as:
- 8/30 = x/100
Where:
- 8 represents the part.
- 30 represents the whole.
- x represents the unknown percentage we want to find.
- 100 represents the total percentage (100%).
To solve for x, we cross-multiply:
- 30x = 8 * 100
- 30x = 800
- x = 800 / 30
- x ≈ 26.67
Therefore, 8 is approximately 26.67% of 30.
Method 2: Using the Decimal Method
This method involves converting the fraction 8/30 to a decimal and then multiplying by 100 to express it as a percentage.
- Divide the part by the whole: 8 / 30 ≈ 0.2667
- Multiply by 100: 0.2667 * 100 ≈ 26.67%
Again, we arrive at the answer: 8 is approximately 26.67% of 30.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply divide 8 by 30 and then multiply the result by 100. The calculator will automatically handle the conversion to a percentage.
Understanding the Result: 26.67%
The result, 26.67%, tells us that 8 represents approximately 26.67 out of every 100 parts of 30. This percentage is a useful way to express the relative size of 8 compared to 30.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in many real-world situations:
1. Retail and Finance:
- Discounts: Calculating the final price after a percentage discount. For example, a 20% discount on a $50 item.
- Taxes: Determining the amount of sales tax or VAT added to a purchase.
- Interest Rates: Calculating interest earned on savings accounts or interest paid on loans.
- Investment Returns: Measuring the percentage increase or decrease in the value of an investment.
2. Data Analysis and Statistics:
- Proportions: Representing parts of a whole as percentages to visualize data effectively.
- Trends: Analyzing percentage changes over time to identify trends and patterns.
- Surveys and Polls: Presenting survey results as percentages to illustrate the distribution of responses.
3. Everyday Life:
- Tip Calculations: Determining the appropriate tip amount in a restaurant.
- Recipe Adjustments: Scaling up or down a recipe by a certain percentage.
- Grade Calculation: Determining your overall grade in a class based on individual assignment percentages.
Advanced Percentage Calculations:
While the example of 8 out of 30 is straightforward, percentage problems can become more complex. Here are some advanced scenarios:
1. Finding the Whole:
If you know a percentage and the part, you can find the whole. For example:
- "20% of what number is 10?"
To solve this, set up the equation:
- 0.20 * x = 10
- x = 10 / 0.20
- x = 50
2. Finding the Part:
If you know a percentage and the whole, you can find the part. For example:
- "What is 35% of 200?"
To solve this, multiply the percentage (as a decimal) by the whole:
- 0.35 * 200 = 70
3. Percentage Increase/Decrease:
Calculating percentage change requires understanding the difference between the original value and the new value. For example:
- A value increases from 50 to 60. The percentage increase is calculated as: ((60-50)/50) * 100 = 20%.
4. Compound Interest:
Compound interest involves earning interest not only on the principal amount but also on accumulated interest. Calculating compound interest requires more complex formulas.
Mastering Percentage Calculations: Tips and Tricks
- Practice Regularly: The more you practice, the more comfortable you'll become with percentage calculations.
- Use Different Methods: Experiment with different methods (proportion, decimal, calculator) to find the approach you find most intuitive.
- Understand the Concepts: Make sure you understand the underlying principles of percentages before tackling complex problems.
- Check Your Answers: Always double-check your calculations to ensure accuracy.
- Utilize Online Resources: Many online resources offer calculators and tutorials on percentage calculations.
Conclusion:
Understanding percentages is a crucial skill for navigating various aspects of life. By mastering the methods outlined in this guide, you'll be equipped to confidently tackle percentage calculations of any complexity, from simple problems like determining what percent 8 is of 30 to more advanced scenarios involving compound interest and percentage change. Remember to practice regularly and utilize different approaches to find the method that best suits your learning style. This will not only improve your mathematical abilities but also enhance your analytical skills and problem-solving capabilities.
Latest Posts
Latest Posts
-
How To Find Perimeter Of A Cylinder
May 14, 2025
-
How Many Thirds Are In The Trapezoid
May 14, 2025
-
2 5 Inches To Cm Ring Size
May 14, 2025
-
How Much Is 45 Liters In Gallons
May 14, 2025
-
What Is 17 Out Of 20 As A Grade
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 8 Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.