What Percent Is 80 Of 200
Treneri
Apr 06, 2025 · 4 min read
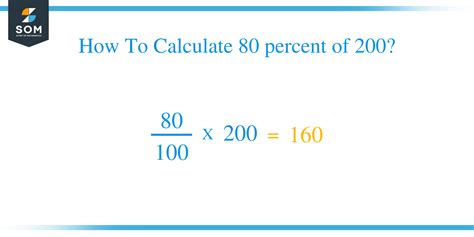
Table of Contents
What Percent is 80 of 200? A Deep Dive into Percentage Calculations
Calculating percentages is a fundamental skill applicable across various fields, from everyday budgeting to complex financial analysis. Understanding how to determine what percent one number represents of another is crucial for making informed decisions and interpreting data effectively. This comprehensive guide will not only answer the question, "What percent is 80 of 200?" but also explore the underlying principles and provide you with the tools to tackle similar percentage problems with confidence.
Understanding Percentages: The Basics
Before diving into the specific calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For instance, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Key Terms and Concepts
- Base: The total amount or the whole. In our example, 200 is the base.
- Part: The portion of the base we're interested in. In our case, 80 is the part.
- Percentage: The fraction of the base expressed as a number out of 100. This is what we aim to calculate.
Calculating "What Percent is 80 of 200?"
There are several ways to approach this problem. Let's explore the most common methods:
Method 1: Using the Formula
The fundamental formula for calculating percentages is:
(Part / Base) * 100 = Percentage
Substituting our values:
(80 / 200) * 100 = 40%
Therefore, 80 is 40% of 200.
Method 2: Simplifying the Fraction
We can simplify the fraction 80/200 before multiplying by 100. Both 80 and 200 are divisible by 80:
80/200 simplifies to 1/2.5
Now, we can calculate:
(1/2.5) * 100 = 40%
Method 3: Using Proportions
This method is particularly helpful for visualizing the relationship between the parts and the whole. We can set up a proportion:
80/200 = x/100
Where 'x' represents the percentage we're trying to find. To solve for 'x', we can cross-multiply:
200x = 8000
x = 8000/200
x = 40
Therefore, x = 40%, confirming our previous calculations.
Beyond the Basics: Expanding Your Percentage Skills
While solving "What percent is 80 of 200?" provides a clear understanding of basic percentage calculations, let's delve into more complex scenarios and practical applications.
Calculating the Percentage Increase or Decrease
Often, we need to determine the percentage change between two values. This is particularly relevant when analyzing trends, comparing data sets, or understanding growth rates.
The formula for percentage change is:
[(New Value - Old Value) / Old Value] * 100 = Percentage Change
Example: If a product's price increases from $100 to $120, the percentage increase is:
[(120 - 100) / 100] * 100 = 20%
Conversely, if the price decreases from $120 to $100, the percentage decrease is:
[(100 - 120) / 120] * 100 = -16.67% (Note the negative sign indicating a decrease)
Applying Percentages to Real-World Problems
Percentage calculations are essential in various everyday situations:
- Shopping: Calculating discounts, sales tax, or tips.
- Finance: Determining interest rates, loan repayments, or investment returns.
- Science: Expressing experimental results, analyzing data, or calculating error margins.
- Data Analysis: Interpreting survey results, analyzing market trends, or visualizing proportions.
Let's consider a few more examples:
Example 1: Discount Calculation
A shirt costs $50, and there's a 20% discount. To find the discounted price:
Discount amount = 20% of $50 = (20/100) * 50 = $10
Discounted price = $50 - $10 = $40
Example 2: Sales Tax Calculation
A book costs $25, and the sales tax is 6%. To find the total cost:
Sales tax amount = 6% of $25 = (6/100) * 25 = $1.50
Total cost = $25 + $1.50 = $26.50
Example 3: Investment Returns
An investment of $1000 grows to $1250. To find the percentage return:
Percentage return = [(1250 - 1000) / 1000] * 100 = 25%
Mastering Percentages: Tips and Tricks
- Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations.
- Use a calculator: For complex calculations, a calculator can save time and ensure accuracy.
- Understand the context: Pay close attention to the problem's wording to ensure you're applying the correct formula.
- Check your work: Always double-check your calculations to avoid errors.
- Break down complex problems: Divide complex problems into smaller, manageable steps.
Conclusion: Percentages in the Real World
Understanding percentages is a critical skill for navigating the complexities of everyday life and professional endeavors. From simple calculations like determining a discount to more advanced applications in financial analysis and data interpretation, the ability to work with percentages empowers you to make informed decisions, analyze data effectively, and understand the numerical world around you. By mastering the fundamental concepts and applying the methods outlined in this guide, you'll be well-equipped to tackle a wide range of percentage problems with confidence and accuracy. Remember that consistent practice and a clear understanding of the underlying principles are key to mastering this essential skill.
Latest Posts
Latest Posts
-
How To Convert Vertex Form Into Standard Form
Apr 09, 2025
-
How Many Hours Is 10 Years
Apr 09, 2025
-
2 5 Ml Is How Many Drops
Apr 09, 2025
-
How Many Days Is 119 Hours
Apr 09, 2025
-
1 8 Km Is How Many Miles
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 80 Of 200 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.