What Percent Of 200 Is 50
Treneri
May 10, 2025 · 4 min read
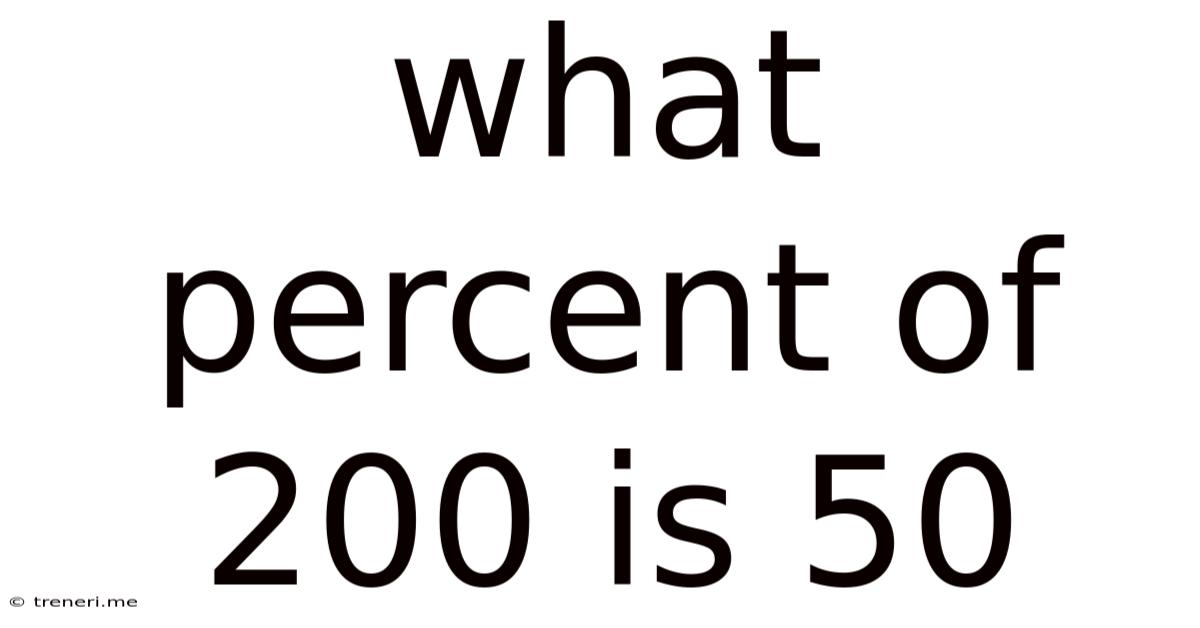
Table of Contents
What Percent of 200 is 50? A Deep Dive into Percentages
This seemingly simple question, "What percent of 200 is 50?", opens the door to a much broader understanding of percentages, their applications, and how to solve percentage problems efficiently. While the answer itself is straightforward, exploring the underlying concepts strengthens mathematical skills and provides a solid foundation for more complex percentage-based calculations.
Understanding Percentages: The Basics
A percentage is simply a fraction expressed as a part of 100. The word "percent" literally means "out of one hundred" (per centum in Latin). Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Understanding this fundamental concept is crucial for solving percentage problems. We can represent percentages in three ways:
- Fraction: 50/100
- Decimal: 0.5
- Percentage: 50%
Solving "What Percent of 200 is 50?"
To solve this specific problem, we can use a simple formula:
(Part / Whole) * 100 = Percentage
In our case:
- Part: 50 (the number we're considering as a portion of the whole)
- Whole: 200 (the total amount)
Plugging these values into the formula:
(50 / 200) * 100 = 25%
Therefore, 50 is 25% of 200.
Alternative Methods
While the above formula is the most direct approach, there are other ways to solve this problem:
-
Using Proportions: We can set up a proportion: 50/200 = x/100. Solving for x (the percentage) gives us x = 25.
-
Mental Math: With practice, you can solve simpler percentage problems mentally. Recognizing that 200 is double 100, you can quickly deduce that half of 100% (which is 50%) is 25%. Since 50 is half of 100, and 100 is half of 200, then 50 is 25% of 200.
Real-World Applications of Percentages
Understanding percentages is essential in numerous real-world scenarios:
1. Finance and Budgeting:
- Interest rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts.
- Discounts and sales: Retailers often advertise discounts as percentages (e.g., "20% off").
- Taxes: Sales tax, income tax, and property tax are all calculated as percentages.
- Investment returns: The performance of investments is often expressed as a percentage return.
2. Science and Statistics:
- Data analysis: Percentages are used extensively in data analysis to represent proportions and trends within datasets.
- Probability: The likelihood of an event occurring is often expressed as a percentage.
- Scientific measurements: Many scientific measurements involve expressing results as percentages (e.g., percentage error).
3. Everyday Life:
- Tip calculations: Calculating tips in restaurants typically involves determining a percentage of the bill.
- Grading systems: Many educational institutions use percentages to represent grades and scores.
- Comparing quantities: Percentages allow for easy comparison of quantities with different base values.
Advanced Percentage Problems and Techniques
While the initial problem was straightforward, percentage calculations can become more complex. Let's explore some advanced scenarios:
1. Finding the Whole when the Percentage and Part are Known:
Let's say you know that 25% of a number is 50. How do you find the whole number? We can modify our formula:
(Part / Percentage) * 100 = Whole
(50 / 25) * 100 = 200
2. Finding the Percentage Increase or Decrease:
Calculating percentage changes is crucial for tracking growth or decline. The formula is:
((New Value - Old Value) / Old Value) * 100 = Percentage Change
For example, if a product's price increased from $100 to $125:
((125 - 100) / 100) * 100 = 25% increase.
3. Compound Interest:
Compound interest involves earning interest on both the principal amount and accumulated interest. The formula is more complex but utilizes percentages at its core.
Tips and Tricks for Mastering Percentages
- Practice regularly: Consistent practice is key to mastering percentage calculations.
- Understand the underlying concepts: Don't just memorize formulas; understand why they work.
- Use visual aids: Diagrams and charts can help visualize percentage relationships.
- Utilize online calculators and resources: Numerous online tools can assist with complex percentage calculations.
- Break down complex problems: Divide larger problems into smaller, manageable steps.
Conclusion: The Significance of Percentage Calculations
The simple question, "What percent of 200 is 50?", serves as a gateway to a vast and practical world of percentage calculations. Mastering these calculations is crucial for success in various fields, from finance and science to everyday life. By understanding the underlying principles and practicing regularly, you can confidently tackle complex percentage problems and utilize this essential mathematical tool effectively. Remember to always approach percentage problems methodically, utilizing the appropriate formulas and techniques, and check your work for accuracy. The ability to confidently and correctly calculate percentages will undoubtedly improve your problem-solving skills and enhance your understanding of quantitative data.
Latest Posts
Latest Posts
-
How Much Time Till 3 05
May 11, 2025
-
14 96 Rounded To The Nearest Tenth
May 11, 2025
-
How Old Is Someone Born In 87
May 11, 2025
-
Least Common Multiple Of 6 8 10
May 11, 2025
-
How Much Drop Should A Tablecloth Have
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 200 Is 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.