What Percent Of 21 Is 7
Treneri
May 14, 2025 · 4 min read
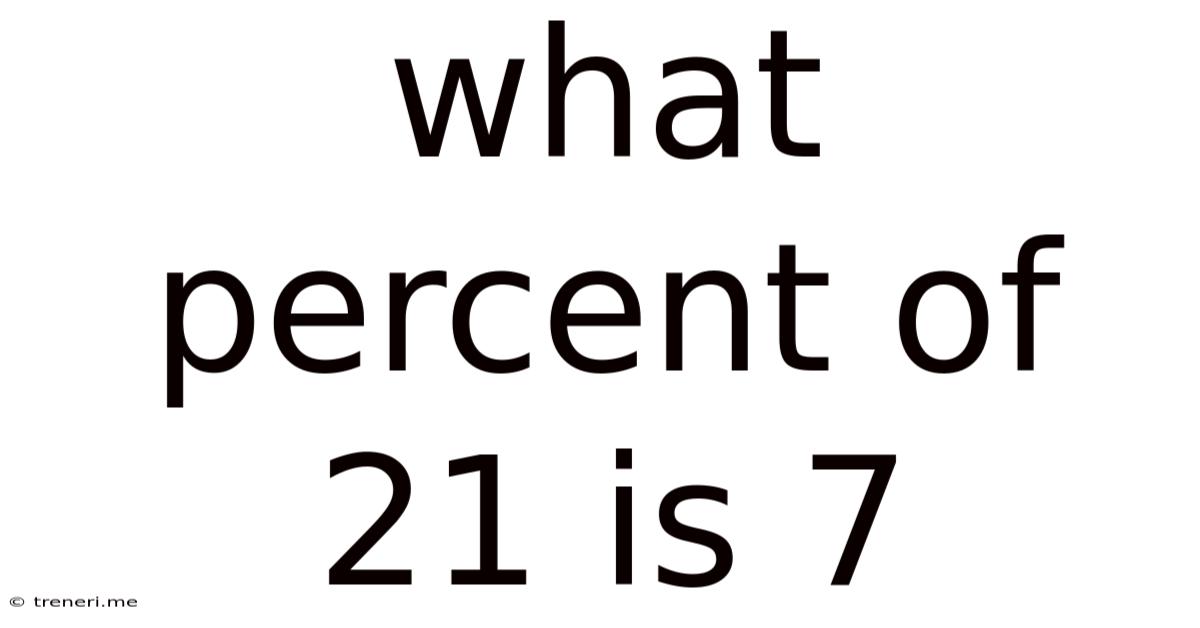
Table of Contents
What Percent of 21 is 7? A Comprehensive Guide to Percentage Calculations
Determining what percentage one number represents of another is a fundamental mathematical skill with wide-ranging applications in various fields, from finance and budgeting to statistics and data analysis. Understanding percentage calculations is crucial for interpreting data, making informed decisions, and communicating effectively. This comprehensive guide will delve into the question, "What percent of 21 is 7?", providing not only the answer but also a detailed explanation of the process, different methods of calculation, and real-world applications.
Understanding Percentages
Before we tackle the specific problem, let's establish a clear understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction ½ or the decimal 0.5.
Calculating "What Percent of 21 is 7?" - Method 1: Using Proportions
One of the most straightforward methods to solve this problem is by setting up a proportion. A proportion is an equation stating that two ratios are equal. We can represent the problem as follows:
- x/100 = 7/21
Where 'x' represents the percentage we are trying to find.
To solve for 'x', we can cross-multiply:
- 21x = 700
Then, divide both sides by 21:
-
x = 700/21
-
x ≈ 33.33
Therefore, 7 is approximately 33.33% of 21.
Calculating "What Percent of 21 is 7?" - Method 2: Using Decimal Conversion
Another approach involves converting the fraction 7/21 into a decimal and then multiplying by 100 to express it as a percentage.
-
Convert the fraction to a decimal: Divide 7 by 21: 7 ÷ 21 ≈ 0.3333
-
Multiply by 100 to express as a percentage: 0.3333 x 100 ≈ 33.33%
This method provides the same result as the proportion method: 7 is approximately 33.33% of 21.
Understanding the Recurring Decimal
Notice that in both methods, we encountered a recurring decimal (0.3333...). This is because the fraction 7/21 simplifies to 1/3, which is a fraction with a non-terminating decimal representation. In practical applications, we often round the decimal to a suitable number of decimal places (e.g., 33.33%). The level of precision needed will depend on the context of the problem.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in numerous real-world scenarios:
1. Finance and Budgeting:
- Calculating interest rates: Understanding percentages is crucial for comprehending interest rates on loans, savings accounts, and investments.
- Determining discounts: Sales and discounts are often expressed as percentages. Calculating the actual discount amount requires percentage calculations.
- Analyzing financial statements: Financial reports use percentages extensively to present key financial metrics like profit margins, debt ratios, and return on investment.
- Tax calculations: Taxes are typically calculated as a percentage of income or the value of goods and services.
2. Statistics and Data Analysis:
- Interpreting survey results: Survey data is often presented as percentages to illustrate the distribution of responses.
- Calculating probabilities: Probabilities are often expressed as percentages to indicate the likelihood of an event occurring.
- Analyzing data trends: Percentage changes are used to track trends and make comparisons over time.
3. Everyday Life:
- Calculating tips: Restaurant tips are usually calculated as a percentage of the bill.
- Determining sale prices: Sales often advertise discounts as percentages.
- Understanding nutritional information: Nutritional labels on food products often express the percentage of daily recommended values for various nutrients.
Advanced Percentage Calculations: Finding the Whole
While this article focuses on determining the percentage one number represents of another, it's useful to also understand how to find the whole when given a percentage and a part. Let's say we know that 33.33% of a number is 7. How do we find the original number?
We can set up the equation:
- 0.3333 * x = 7
Solving for x:
-
x = 7 / 0.3333
-
x ≈ 21
This confirms our original calculation: 7 is approximately 33.33% of 21.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill that enhances your problem-solving abilities across various disciplines. The examples provided, along with the detailed explanations of different calculation methods, equip you with the tools to confidently tackle percentage-related problems. Remember that practice is key to mastering any mathematical concept, so try solving various percentage problems to solidify your understanding. By understanding percentages, you will be better equipped to interpret data, make informed decisions, and navigate the quantitative aspects of everyday life and professional endeavors. From budgeting and financial analysis to understanding statistical data and making everyday purchases, the ability to quickly and accurately work with percentages is an invaluable asset. So next time you encounter a percentage problem, remember the methods discussed here and approach it with confidence and clarity.
Latest Posts
Latest Posts
-
How Much Days Until June 1
May 14, 2025
-
How Many Quarts Are In 28 Cups
May 14, 2025
-
How To Calculate Z Score On Calculator
May 14, 2025
-
12x20 Is How Many Square Feet
May 14, 2025
-
What Grade Is 11 Out Of 20
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 21 Is 7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.