What Percent Of 250 Is 70
Treneri
May 14, 2025 · 4 min read
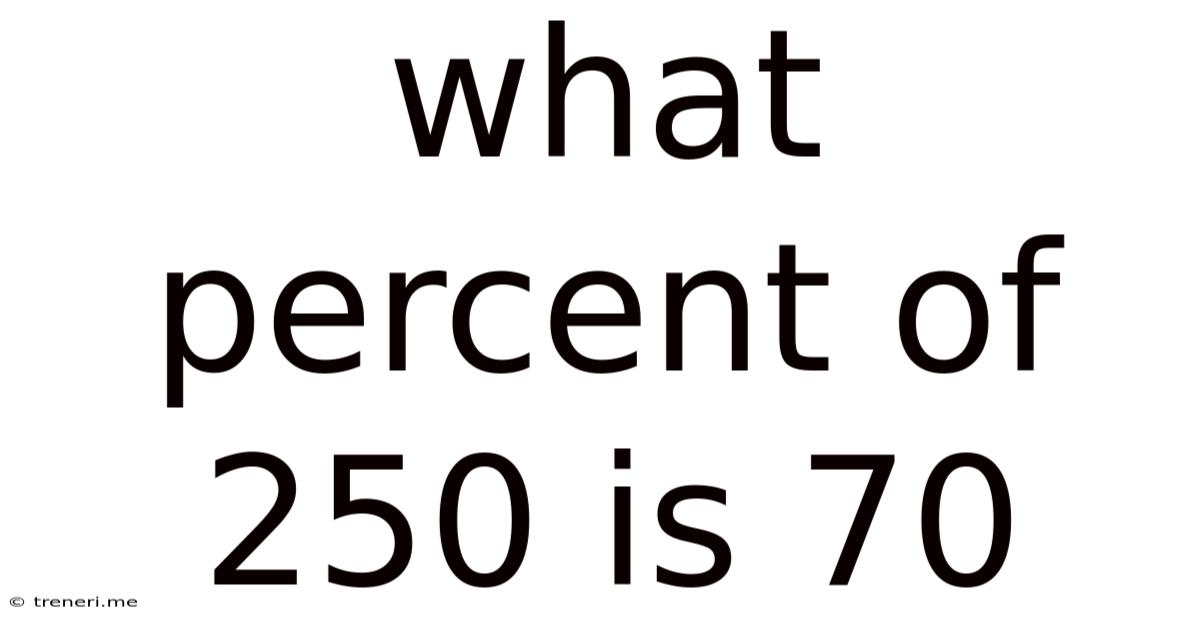
Table of Contents
What Percent of 250 is 70? A Comprehensive Guide to Percentage Calculations
Determining what percentage one number represents of another is a fundamental skill in mathematics with broad applications in various fields, from finance and statistics to everyday life. This article will delve into the process of calculating what percentage 70 represents of 250, providing a step-by-step explanation, exploring different calculation methods, and offering practical examples to solidify your understanding. We'll also touch upon the broader context of percentage calculations and their importance.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. It represents a portion of a whole. The symbol used to denote percentage is "%". For example, 50% means 50 out of 100, which is equivalent to the fraction ½ or the decimal 0.5.
Calculating "What Percent of 250 is 70?"
There are several ways to approach this problem. Let's explore the most common methods:
Method 1: Using the Formula
The fundamental formula for percentage calculations is:
(Part / Whole) x 100% = Percentage
In our case:
- Part: 70
- Whole: 250
Substituting these values into the formula:
(70 / 250) x 100% = 28%
Therefore, 70 is 28% of 250.
Method 2: Using Proportions
We can also solve this using proportions. We set up a proportion where x represents the unknown percentage:
x / 100 = 70 / 250
To solve for x, we cross-multiply:
250x = 7000
Then, divide both sides by 250:
x = 7000 / 250 = 28
Therefore, x = 28%, confirming our previous result.
Method 3: Using Decimal Conversion
First, divide the part by the whole:
70 / 250 = 0.28
Then, multiply the result by 100% to convert the decimal to a percentage:
0.28 x 100% = 28%
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world scenarios:
- Finance: Calculating interest rates, discounts, tax percentages, profit margins, and investment returns. For example, if a product is discounted by 28%, you can easily calculate the final price.
- Statistics: Representing data in charts and graphs, analyzing survey results, calculating probabilities, and understanding statistical significance. Percentages provide a concise and readily interpretable way to present statistical information.
- Science: Expressing concentrations of solutions, measuring reaction yields, and representing data from experiments. Scientific research often relies on precise percentage calculations for accurate analysis.
- Everyday Life: Calculating tips in restaurants, determining sale prices, understanding nutritional information on food labels, and comparing different deals and offers. Percentage knowledge helps in making informed decisions in daily life.
Beyond the Basics: More Complex Percentage Problems
While the example of "What percent of 250 is 70?" provides a foundational understanding, percentage problems can become more complex. Let's explore some variations:
Finding the Whole Given the Part and Percentage
If we know that 28% of a number is 70, how can we find the original number (the whole)?
We can use the formula:
Whole = (Part / Percentage) x 100
Whole = (70 / 28) x 100 = 250
This confirms our original whole number.
Finding the Part Given the Whole and Percentage
If we know that 28% of 250 is what number? We can use this formula:
Part = (Percentage/100) x Whole
Part = (28/100) x 250 = 70
This reiterates the original problem's solution.
Percentage Increase and Decrease
Percentage change calculations are frequently used to show the increase or decrease in a value over time. The formula for percentage change is:
Percentage Change = [(New Value - Old Value) / Old Value] x 100%
For example, if a stock price increases from $100 to $128, the percentage increase is:
[(128 - 100) / 100] x 100% = 28%
Tips for Mastering Percentage Calculations
- Practice Regularly: The more you practice, the more comfortable you'll become with percentage calculations. Work through various examples and try different methods.
- Understand the Formula: Ensure you understand the underlying formula and how it applies to different scenarios.
- Use a Calculator: Calculators can significantly speed up the calculation process, especially for complex problems.
- Check Your Work: Always double-check your answers to avoid errors.
Conclusion
Calculating what percent of 250 is 70, which equals 28%, is a straightforward but essential mathematical operation with wide-ranging applications. By mastering this fundamental skill and understanding the different calculation methods, you'll enhance your problem-solving abilities and improve your understanding of numerical data across diverse fields. The ability to confidently handle percentage calculations empowers you to make better decisions in various aspects of your life, from managing personal finances to analyzing complex data sets. Remember to practice consistently to build fluency and accuracy in your calculations. The more you work with percentages, the more intuitive and natural this vital mathematical skill will become.
Latest Posts
Latest Posts
-
3 4 To The Second Power
May 14, 2025
-
How Many Grams Is 1 4 Oz
May 14, 2025
-
Long Course To Short Course Conversion Asa
May 14, 2025
-
How To Calculate Cause Specific Mortality Rate
May 14, 2025
-
Which Of The Following Are Geometric Sequences
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 250 Is 70 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.