What Percent Of 27 Is 18
Treneri
May 13, 2025 · 4 min read
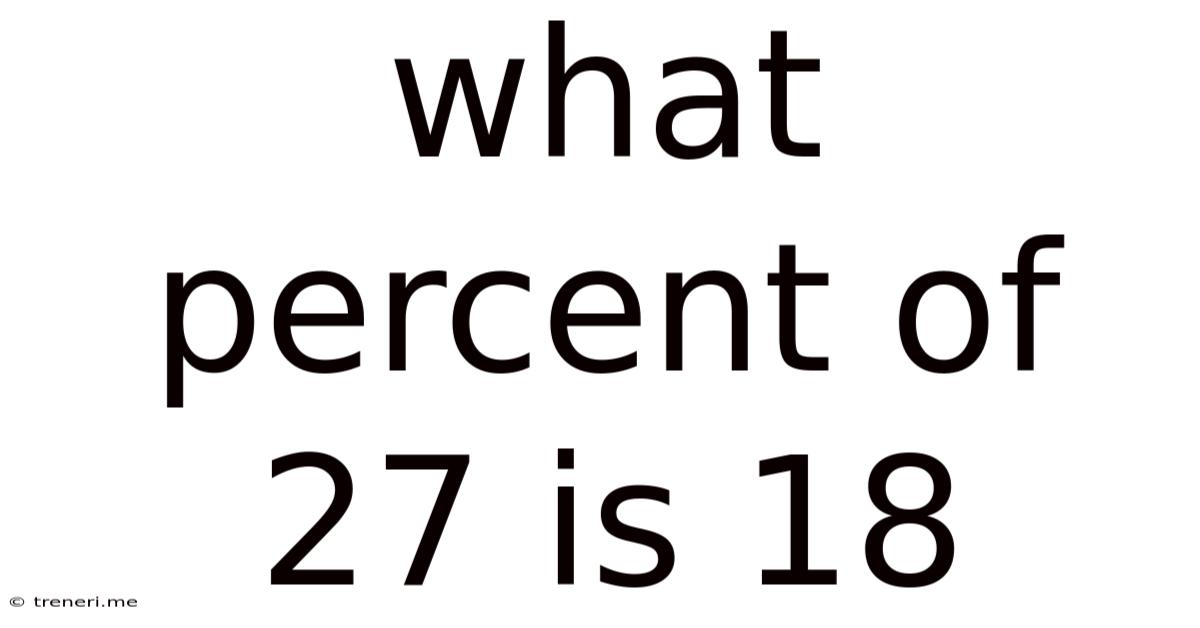
Table of Contents
What Percent of 27 is 18? A Comprehensive Guide to Percentage Calculations
Finding what percentage one number represents of another is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts and tax rates to understanding statistics and financial reports. This comprehensive guide will delve into the question, "What percent of 27 is 18?", providing not just the answer but a thorough understanding of the underlying principles and various methods to solve similar percentage problems.
Understanding Percentages
Before tackling the specific problem, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" is used to denote percentage. For instance, 50% means 50 out of 100, which simplifies to 1/2 or 0.5.
Percentages are crucial for representing proportions and comparing different quantities. They provide a standardized way to express parts of a whole, making it easy to understand and interpret data across diverse contexts.
Method 1: Using the Formula
The most straightforward method to determine what percent of 27 is 18 is to use the basic percentage formula:
(Part / Whole) x 100% = Percentage
In our case:
- Part: 18 (the number we want to express as a percentage)
- Whole: 27 (the total number)
Substituting these values into the formula:
(18 / 27) x 100% = Percentage
Solving the equation:
(0.6667) x 100% ≈ 66.67%
Therefore, 18 is approximately 66.67% of 27.
Understanding the Calculation
The formula works by first finding the ratio of the part to the whole. Dividing 18 by 27 gives us a decimal value (0.6667). Multiplying this decimal by 100% converts it into a percentage, representing the proportional relationship between 18 and 27.
Method 2: Setting up a Proportion
Another effective approach involves setting up a proportion. We can represent the problem as:
18 / 27 = x / 100
Where 'x' represents the percentage we're trying to find. To solve for x, we can cross-multiply:
18 * 100 = 27 * x
1800 = 27x
x = 1800 / 27
x ≈ 66.67
This confirms our earlier result: 18 is approximately 66.67% of 27.
Understanding Proportions
Proportions demonstrate the equivalence of two ratios. By setting up the proportion (18/27 = x/100), we create a relationship that allows us to solve for the unknown percentage (x) by maintaining the equality between the two ratios. Cross-multiplication is a key technique for solving such proportions.
Method 3: Using a Calculator
For quick calculations, a calculator is invaluable. Simply divide 18 by 27, and then multiply the result by 100 to obtain the percentage. Most calculators have a percentage function that simplifies this process even further.
Real-World Applications
Understanding percentage calculations is essential in many real-world scenarios:
- Retail Discounts: If an item is discounted by a certain percentage (e.g., 20% off), knowing how to calculate the actual discount amount is crucial.
- Tax Calculations: Calculating sales tax or income tax involves understanding percentages.
- Financial Analysis: Investors use percentage changes to track stock performance, assess investment returns, and analyze financial statements.
- Statistical Data Interpretation: Percentages are extensively used in representing and interpreting statistical data in various fields like demographics, market research, and scientific studies.
- Grade Calculation: Many grading systems use percentages to represent student performance.
- Tip Calculation: Determining the appropriate tip amount in a restaurant often involves calculating a percentage of the bill.
Expanding Your Understanding: Variations on the Problem
While we've focused on "What percent of 27 is 18?", let's explore related questions and expand our problem-solving capabilities:
-
What is 66.67% of 27? This is the reverse of our original problem. To solve, we multiply 27 by 0.6667 (the decimal equivalent of 66.67%). The result, approximately 18, confirms our calculations.
-
18 is what percent of 54? Following the formula, we'd calculate (18/54) x 100% = 33.33%. This highlights how changing the "whole" number significantly alters the percentage.
-
What number is 75% of 27? Here, we would calculate 0.75 x 27 = 20.25. This demonstrates finding a part given the percentage and the whole.
Tips and Tricks for Solving Percentage Problems
-
Master the Formula: Familiarize yourself with the basic percentage formula: (Part / Whole) x 100% = Percentage. Understanding this formula is the cornerstone of solving various percentage problems.
-
Convert Percentages to Decimals: Converting percentages to decimals (e.g., 25% to 0.25) simplifies calculations.
-
Use Proportions Effectively: Setting up proportions can be particularly helpful in solving more complex percentage problems.
-
Practice Regularly: The more you practice solving percentage problems, the more comfortable and proficient you'll become. Start with simple problems and gradually increase the complexity.
-
Check Your Answers: Always double-check your work to ensure accuracy. Use a calculator to verify your results and identify any potential errors.
Conclusion
Determining "What percent of 27 is 18?" is more than just a simple mathematical problem; it's a gateway to understanding the power and versatility of percentage calculations. By mastering the different methods outlined in this guide – using the formula, setting up proportions, and utilizing a calculator – you can confidently tackle various percentage problems encountered in everyday life and across different academic and professional domains. Remember to practice regularly and utilize these techniques to build a strong foundation in percentage calculations. This knowledge will undoubtedly prove invaluable in navigating numerous quantitative situations.
Latest Posts
Latest Posts
-
30 000 Is 20 Percent Of What
May 14, 2025
-
How Many Sig Figs In 0 2
May 14, 2025
-
What Is A 6 Out Of 8 Letter Grade
May 14, 2025
-
What Is 28 Rounded To The Nearest Ten
May 14, 2025
-
Drag The Numbers To Order Them From Least To Greatest
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 27 Is 18 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.