What Percentage Is 3 Out Of 24
Treneri
May 15, 2025 · 5 min read
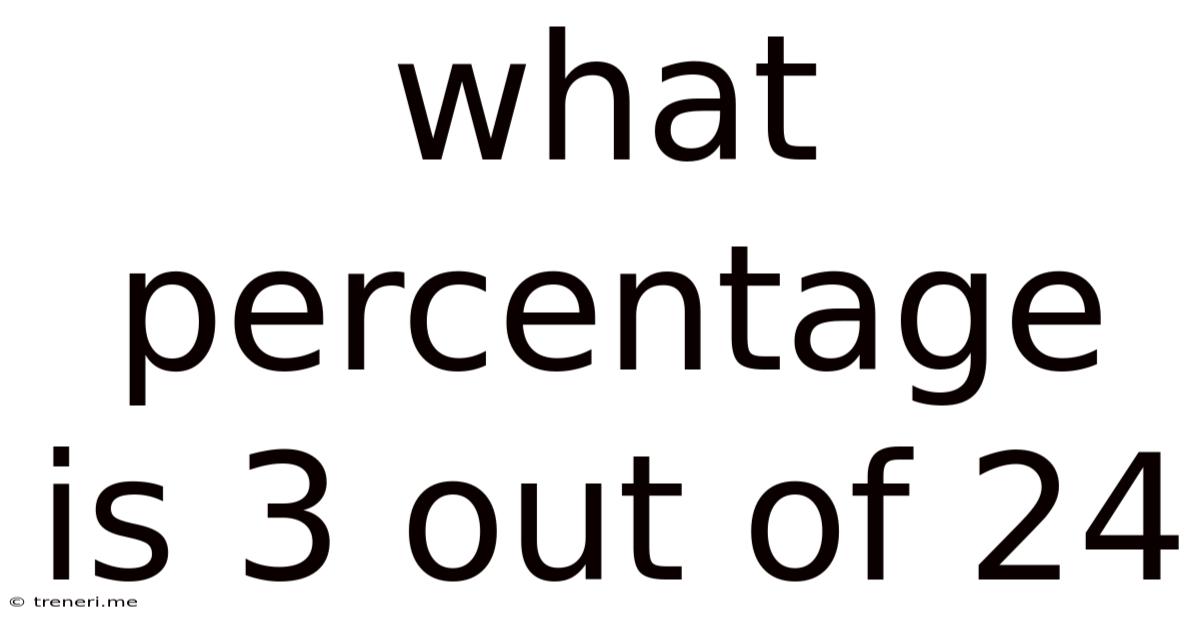
Table of Contents
What Percentage is 3 out of 24? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts in a shop to analyzing data in a professional setting. This comprehensive guide will not only answer the question "What percentage is 3 out of 24?" but also equip you with the knowledge and tools to calculate percentages confidently and efficiently in various scenarios.
Calculating the Percentage: 3 out of 24
The question "What percentage is 3 out of 24?" involves a simple yet crucial concept in mathematics: calculating a percentage from a fraction. Let's break down the process step-by-step:
1. Express the Values as a Fraction:
The first step is to represent "3 out of 24" as a fraction: 3/24.
2. Convert the Fraction to a Decimal:
To convert a fraction to a decimal, divide the numerator (the top number) by the denominator (the bottom number): 3 ÷ 24 = 0.125
3. Convert the Decimal to a Percentage:
To convert a decimal to a percentage, multiply the decimal by 100 and add the percentage symbol (%): 0.125 x 100 = 12.5%
Therefore, 3 out of 24 is 12.5%.
Understanding the Fundamentals of Percentages
Percentages are a way of expressing a number as a fraction of 100. The term "percent" originates from the Latin "per centum," meaning "out of a hundred." Understanding this basic definition is key to mastering percentage calculations.
Key Components of Percentage Calculations
-
Part: This represents the specific portion of the whole that you're interested in. In our example, the part is 3.
-
Whole: This represents the total amount or the complete entity. In our example, the whole is 24.
-
Percentage: This is the ratio of the part to the whole, expressed as a number out of 100.
Different Methods for Calculating Percentages
While the method above is straightforward, there are several approaches to calculating percentages, each suited to different situations and levels of mathematical comfort.
Method 1: The Basic Formula
The fundamental formula for calculating percentages is:
(Part / Whole) x 100 = Percentage
This formula can be applied to a wide range of problems. Let's illustrate this with a few examples:
-
Example 1: What percentage is 15 out of 60? (15/60) x 100 = 25%
-
Example 2: What percentage is 8 out of 32? (8/32) x 100 = 25%
-
Example 3: A store offers a 20% discount on an item originally priced at $50. What is the discount amount? (20/100) x $50 = $10
Method 2: Using Proportions
Proportions offer another powerful way to solve percentage problems. A proportion is an equation that states that two ratios are equal. We can set up a proportion to find the unknown percentage:
- Part / Whole = Percentage / 100
Let's solve our original problem using proportions:
3 / 24 = x / 100
To solve for x (the percentage), cross-multiply:
24x = 300
x = 300 / 24 = 12.5
Therefore, 3 out of 24 is 12.5%.
Method 3: Using Decimal Conversion (as shown in the initial example)
As demonstrated earlier, converting the fraction to a decimal and then multiplying by 100 is a direct and efficient method. This method is particularly useful when dealing with fractions that are easily converted to decimals.
Real-World Applications of Percentage Calculations
Percentages are ubiquitous in everyday life and professional settings. Here are just a few examples:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and returns on investments.
-
Retail: Determining sale prices, markups, and discounts.
-
Science: Expressing experimental results, analyzing data, and representing proportions in scientific studies.
-
Statistics: Representing data in charts, graphs, and reports, calculating probabilities and frequencies.
-
Everyday Life: Understanding tips, calculating nutritional information, and interpreting survey results.
Advanced Percentage Calculations: Percentage Increase and Decrease
Beyond the basic calculations, understanding percentage increase and decrease is crucial for a deeper understanding of percentages.
Percentage Increase
A percentage increase represents the increase in a value relative to its original value. The formula is:
[(New Value - Original Value) / Original Value] x 100 = Percentage Increase
Example: A stock price increases from $10 to $12. The percentage increase is:
[(12 - 10) / 10] x 100 = 20%
Percentage Decrease
Similarly, a percentage decrease represents the decrease in a value relative to its original value. The formula is:
[(Original Value - New Value) / Original Value] x 100 = Percentage Decrease
Example: A product's price decreases from $50 to $40. The percentage decrease is:
[(50 - 40) / 50] x 100 = 20%
Troubleshooting Common Percentage Calculation Errors
Even with a clear understanding of the concepts, errors can occur. Here are some common mistakes to watch out for:
-
Incorrect order of operations: Always follow the order of operations (PEMDAS/BODMAS) when performing calculations involving multiple steps.
-
Incorrect decimal placement: Ensure the decimal point is placed correctly when converting between decimals and percentages.
-
Using the wrong formula: Select the appropriate formula depending on the specific problem.
-
Rounding errors: Be mindful of rounding errors, especially when dealing with multiple calculations. Round your final answer appropriately to maintain accuracy.
Conclusion: Mastering Percentage Calculations
Understanding percentages is a vital skill with broad applications across numerous fields. By mastering the fundamental concepts, various calculation methods, and potential pitfalls, you'll be well-equipped to handle percentage calculations confidently and accurately, whether it's calculating discounts, analyzing data, or understanding financial statements. Remember to practice regularly to solidify your understanding and improve your efficiency. The ability to work fluently with percentages is a valuable asset in both personal and professional endeavors.
Latest Posts
Latest Posts
-
88 83 Is What Percent Of 21
May 15, 2025
-
Cuantas Semanas Tiene Un Ano De 365 Dias
May 15, 2025
-
How Many Ounce In A Kilogram
May 15, 2025
-
How Many Cubic Feet Is 55 Quarts Of Potting Soil
May 15, 2025
-
What Is The Gcf For 18 And 32
May 15, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 3 Out Of 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.